Resource Book for Geometry Answers

Mathematical challenges can often appear daunting, but with the right tools and techniques, they become more manageable. Having access to well-organized materials can significantly enhance understanding and improve problem-solving efficiency. These resources offer structured methods, clear explanations, and practical examples to aid in overcoming complex exercises.

Mastering problem-solving requires not only learning formulas but also developing a deeper understanding of the concepts behind them. With the correct materials at hand, learners can explore various approaches to resolve issues, building confidence in their skills and knowledge.

Clear guidance and examples are essential for those seeking to improve their proficiency. Whether you’re tackling basic exercises or delving into more intricate topics, well-curated references can offer the insights needed to succeed. By applying these techniques, anyone can enhance their ability to navigate even the most challenging tasks.

Comprehensive Guide to Solving Mathematical Problems

Having a well-organized reference at your disposal is crucial when tackling mathematical tasks. The right materials can provide clarity, simplify complex problems, and give structured approaches to finding solutions. These resources offer a collection of methods, examples, and step-by-step instructions to ensure that even the toughest exercises can be approached with confidence.

Key Features of Effective Study Materials

Effective learning materials should be more than just a collection of answers. They should encourage understanding, providing context and rationale for each solution. This enables learners to not only resolve specific problems but also grasp the underlying principles. Key features include:

Feature	Description
Step-by-Step Solutions	Clear, detailed instructions that guide users through the process of solving problems.
Visual Aids	Illustrations and diagrams that make abstract concepts easier to understand.
Practice Exercises	A variety of problems for learners to solve, reinforcing what they’ve learned.
Real-World Applications	Examples that show how mathematical concepts apply to everyday situations.

How to Maximize Your Learning Experience

To get the most out of these materials, it’s essential to engage with the content actively. Rather than passively reading through examples, learners should attempt problems on their own before reviewing solutions. This helps reinforce memory and understanding. Regular practice, combined with thoughtful analysis of each solution, ensures that progress is made effectively and efficiently.

Essential Problem-Solving Strategies

Solving complex mathematical exercises requires more than just applying formulas; it involves a structured approach to understanding the problem, recognizing patterns, and using logical reasoning. Mastering a few essential techniques can significantly improve your ability to tackle various types of problems effectively. These strategies help break down challenges into manageable steps, guiding you toward the right solution.

Breaking Down Problems into Manageable Steps

One of the most effective strategies is to divide a problem into smaller, more manageable parts. By focusing on one aspect at a time, the task becomes less overwhelming. Start by identifying what is given and what needs to be determined, then work through the necessary steps methodically. This systematic approach prevents confusion and ensures no details are overlooked.

Using Visual Aids to Clarify Concepts

Visual representations, such as diagrams and graphs, are invaluable tools when solving mathematical problems. They help make abstract concepts more concrete, allowing you to visualize relationships between elements. Drawing a diagram or chart can often lead to immediate insights, providing a clearer path to the solution.

Understanding Mathematical Concepts Through Study Materials

Grasping complex mathematical principles requires more than just memorizing formulas or procedures. It involves developing a deep understanding of the core concepts that govern the subject. Effective study materials can serve as guides, helping learners connect abstract ideas with real-world applications. These tools break down difficult topics, providing clarity and enhancing comprehension through examples and detailed explanations.

By engaging with well-organized materials, students can explore different approaches to solving problems, gaining insight into how various concepts interact with each other. Whether through worked-out examples, step-by-step explanations, or visual aids, these resources offer valuable perspectives that make challenging topics more accessible. They help bridge the gap between theory and practice, ensuring that learners build a strong foundation for tackling even the most complex exercises.

How to Use a Study Guide Effectively

Maximizing the benefits of any study tool requires more than just flipping through pages for answers. To truly understand and retain the material, it’s essential to approach the guide in a systematic and active way. This means engaging with the content thoughtfully, applying what you learn, and practicing regularly to reinforce your skills. An organized and strategic approach can significantly improve comprehension and problem-solving abilities.

Steps for Effective Use

To make the most of your study tool, follow these key steps:

• Familiarize Yourself with the Structure – Before diving into exercises, take time to understand how the material is organized. Identify the main sections and key concepts covered.
• Read Explanations Carefully – Thoroughly read the examples and explanations provided. Ensure you understand each step before attempting to solve similar problems on your own.
• Practice with Purpose – Don’t just solve problems randomly. Focus on the areas where you need the most improvement and track your progress.
• Seek Clarification When Needed – If a particular concept is unclear, review the related sections or seek additional explanations to reinforce your understanding.

Incorporating Active Learning

Active learning helps ensure that the information is not just passively absorbed, but deeply understood. You can achieve this by:

1. Rewriting Solutions in Your Own Words – Restate the process in your own terms to ensure you grasp the reasoning behind each step.
2. Creating Practice Problems – Challenge yourself by creating new problems based on the material you’ve studied. This reinforces your learning and helps you think critically about the concepts.
3. Reviewing Regularly – Consistently review past topics to reinforce what you’ve learned and maintain long-term retention.

Step-by-Step Solutions for Mathematical Challenges

Breaking down a complex problem into manageable steps is a crucial technique for understanding and solving difficult tasks. By following a logical sequence of actions, learners can clearly see how each part of the problem is addressed, making it easier to arrive at the correct solution. This approach not only simplifies the problem-solving process but also helps reinforce key concepts and principles.

Effective Steps to Follow

When tackling a mathematical problem, it’s essential to follow a structured approach. Here’s a breakdown of the steps that can guide you through the solution:

• Identify the Given Information – Carefully read the problem to understand what is provided and what needs to be determined.
• Choose the Right Method – Decide which mathematical technique or formula is best suited for solving the problem.
• Break the Problem into Smaller Parts – Divide the task into more manageable steps to avoid feeling overwhelmed.
• Carry Out Calculations – Perform any necessary calculations, ensuring to check each step for accuracy.
• Review the Solution – After obtaining the result, go over the steps to ensure no mistakes were made and that the solution makes sense in the context of the problem.

Common Techniques for Solving Problems

Several techniques can be applied to help clarify and solve mathematical problems. Some of the most effective include:

1. Visual Representation – Draw diagrams or graphs to help visualize the relationships between different elements of the problem.
2. Logical Deduction – Use reasoning and logical steps to eliminate incorrect possibilities and narrow down the correct solution.
3. Work Backwards – In some cases, starting from the desired outcome and working backwards can lead to a clearer understanding of the process.
4. Check for Special Cases – Sometimes problems have special conditions or exceptions, so it’s important to test for these and adjust the solution accordingly.

Finding Accurate Solutions Quickly

In mathematics, speed and accuracy are crucial when solving problems. Whether preparing for an exam or working on assignments, knowing how to quickly find the correct solution is an essential skill. By adopting efficient strategies and focusing on key methods, you can streamline the process and reduce the time spent on each problem without sacrificing precision.

Key Strategies for Fast Problem Solving

To accelerate your problem-solving ability while maintaining accuracy, consider these approaches:

• Familiarize Yourself with Common Formulas – Having essential formulas memorized saves valuable time during calculations. Understanding how and when to apply them can make the process much faster.
• Use Shortcut Techniques – For certain types of problems, there are shortcut methods that can quickly lead to the correct answer. For example, recognizing patterns in numbers or shapes can allow you to skip repetitive steps.
• Break Down the Problem – Divide complex problems into smaller, more manageable parts. By tackling each part individually, you can work faster and avoid missing crucial details.
• Check Units and Conversions – Ensuring that all units are consistent and correctly converted at the beginning of the process can prevent costly mistakes and the need for recalculations later.

Practical Tools to Improve Speed

Using certain tools and techniques can help speed up the process while ensuring accurate results. Some useful methods include:

• Visual Aids – Sketching diagrams or using graphs can help clarify the problem quickly and lead to faster insights.
• Online Calculators – For more complex problems, using reliable digital tools can speed up the calculation process and provide instant verification of results.
• Practice and Familiarity – The more you practice, the faster you’ll recognize patterns and identify the most efficient methods for solving problems.

Common Mistakes in Geometry and How to Avoid Them

Even the most experienced learners can make errors when solving mathematical problems. Recognizing common mistakes and understanding how to avoid them is key to mastering the subject. Many of these errors arise from misunderstandings of fundamental concepts or simple oversights during calculations. By staying mindful of potential pitfalls, you can improve accuracy and avoid wasting time on incorrect solutions.

Frequent Errors and How to Overcome Them

Here are some of the most common mistakes and practical tips for preventing them:

• Ignoring Units of Measurement – One of the easiest mistakes is failing to properly account for units. Always ensure that units are consistent throughout the problem, and convert them when necessary to avoid errors.
• Incorrect Use of Formulas – Misapplying formulas is a frequent mistake, especially when the variables or conditions change. Double-check the formula you are using to ensure it fits the situation correctly.
• Skipping Steps in Complex Problems – Rushing through problems can lead to skipped steps or miscalculations. Always work through each part of the process, even if it seems repetitive.
• Overlooking Special Cases – Some problems have unique conditions or exceptions. Be sure to carefully read all the instructions and watch for special cases that may require different solutions.

Tips for Improving Accuracy

To minimize mistakes and improve your problem-solving skills, consider these strategies:

• Double-Check Your Work – Before finalizing your answer, go back through the solution and verify each step. This extra review can catch small errors that might otherwise go unnoticed.
• Practice Regularly – The more you practice, the more familiar you become with the types of mistakes you are prone to make. This self-awareness helps you avoid similar errors in the future.
• Ask for Feedback – If you’re unsure about a solution, seek input from peers or instructors. An external perspective can help identify mistakes that you may have overlooked.

Benefits of Visual Learning in Mathematics

Visual learning plays a crucial role in enhancing understanding, particularly in subjects that involve spatial reasoning and abstract concepts. By using diagrams, charts, and visual aids, learners can more easily grasp complex relationships and patterns. This method of learning not only aids in comprehension but also helps retain information more effectively, as it engages both the analytical and creative parts of the brain.

Why Visual Learning is Effective

There are several reasons why incorporating visuals into problem-solving can lead to better outcomes:

• Improved Understanding of Relationships – Visual tools, such as diagrams and graphs, allow learners to see the connections between various elements in a problem, making abstract concepts more tangible and easier to comprehend.
• Enhanced Memory Retention – When information is presented visually, it is easier to remember. Our brains are wired to recall images and patterns better than text alone, making visual learning a powerful tool for long-term retention.
• Clarification of Complex Problems – Complex tasks often become simpler when visualized. Sketching out a problem or drawing a diagram can make it easier to break down the steps and identify the necessary solutions.
• Increased Engagement – Visuals make learning more engaging and dynamic. When learners actively participate in creating or interpreting visuals, it fosters deeper involvement and a more interactive learning experience.

How to Incorporate Visual Learning

Here are some effective ways to use visual learning techniques to improve problem-solving skills:

1. Draw Diagrams and Shapes – Whenever possible, draw out the problem. Visualizing shapes, angles, or relationships can make the problem clearer and help you find solutions more easily.
2. Use Color Coding – Color coding different parts of a problem, such as angles, lines, or variables, can help you better distinguish between components and see their interactions more clearly.
3. Leverage Graphs and Charts – For problems involving measurements, proportions, or trends, graphs can offer a straightforward way to visualize data and spot patterns.
4. Interactive Tools and Apps – Many digital tools allow for interactive learning. These resources let you manipulate visual elements, experiment with different configurations, and visualize the impact of changes in real-time.

Using Diagrams to Clarify Concepts

Visual aids, particularly diagrams, are invaluable when it comes to understanding complex mathematical ideas. By translating abstract concepts into clear, visual forms, diagrams help break down information into more digestible parts. This approach makes it easier to see relationships, identify patterns, and grasp the underlying principles of a problem. Whether for simplifying calculations or aiding comprehension, diagrams play a pivotal role in mastering the subject.

How Diagrams Enhance Understanding

Here’s how diagrams can improve the learning process:

• Clarification of Spatial Relationships – Diagrams allow learners to see how different elements relate to each other spatially. For example, visualizing angles, lines, and shapes makes it easier to understand their connections.
• Reduction of Cognitive Load – By turning a complex problem into a visual representation, you reduce the mental effort needed to keep track of multiple details, making the problem more manageable.
• Easy Identification of Key Elements – In problems involving multiple components, diagrams highlight the most important parts, allowing you to focus on the essential aspects of the task without distraction.
• Improved Error Detection – Diagrams can help spot mistakes by providing a visual check of the work done so far. By comparing your diagram with your written steps, you can easily identify inconsistencies or miscalculations.

Effective Ways to Use Diagrams

To get the most out of diagrams, consider the following tips:

1. Draw All Relevant Elements – Be sure to include every part of the problem in your diagram, such as lines, angles, and intersections. The more complete your diagram, the more useful it will be.
2. Label Key Features – Clearly label important elements of your diagram, such as the length of a side, the measure of an angle, or specific points. This will help you refer to them quickly during problem-solving.
3. Use Color or Shading – Differentiate parts of the diagram using color or shading to emphasize relationships or separate distinct areas of the problem. This technique can make your diagram easier to interpret.
4. Start with Simple Sketches – Begin with basic sketches and refine them as you work through the problem. Even a rough drawing can provide insight into the best approach to take.

Practical Applications of Geometry in Daily Life

The principles of spatial relationships and measurements play a vital role in many everyday activities. From designing buildings to creating art, understanding shapes, angles, and proportions helps solve problems efficiently and make informed decisions. This knowledge extends beyond theoretical learning, influencing everything from the layout of our living spaces to the design of the products we use daily.

Everyday Uses of Spatial Understanding

Here are a few common situations where spatial reasoning and measurement are essential:

• Interior Design – Whether arranging furniture or planning room layouts, knowing how objects fit together within a given space requires a solid grasp of angles, proportions, and spatial organization.
• Construction and Architecture – Builders and architects rely on precise measurements and understanding of shapes to design structures, ensuring stability and aesthetic appeal.
• Art and Design – Artists and designers often use geometric concepts to create visually balanced compositions. Symmetry, proportion, and perspective are integral to crafting appealing works.
• Sports – Athletes, especially in sports like basketball or soccer, rely on geometry to improve their strategies. Calculating distances, angles, and positioning are essential for making successful plays.

Geometry in Technology and Innovation

Advancements in technology and innovation are also closely tied to geometric principles:

• Engineering and Robotics – Engineers use geometry to design machinery, vehicles, and even robots, ensuring proper movement, function, and efficiency within defined spaces.
• Navigation and Mapping – GPS systems and mapmaking depend on geometric concepts to calculate distances, directions, and positioning, helping us find the best routes and locations.
• Product Design – In product manufacturing, geometric knowledge is essential for creating ergonomic, functional, and aesthetically pleasing items, from smartphones to furniture.

How Guides Improve Geometry Understanding

Having access to structured materials can significantly enhance one’s grasp of mathematical concepts. These resources provide organized explanations, step-by-step instructions, and clear examples, all of which support deeper comprehension. By offering a variety of methods to approach problems, they cater to different learning styles and help clarify challenging ideas, ensuring that students are well-equipped to tackle complex problems with confidence.

Key Benefits of Structured Learning Materials

Using comprehensive guides offers several advantages for mastering the subject:

• Clear and Concise Explanations – Step-by-step guides present complex topics in an easy-to-understand format, breaking down each element for better clarity.
• Multiple Examples – By providing numerous examples, these resources help learners see how various principles are applied in different contexts, making abstract concepts more concrete.
• Practice Problems – These materials often come with a wide range of practice exercises, allowing students to reinforce what they’ve learned and build problem-solving skills.
• Visual Aids – Diagrams and charts are frequently included, which can make understanding shapes, angles, and their relationships much easier.

How They Foster Independent Learning

One of the most significant advantages of using structured learning resources is that they promote self-sufficiency. Learners can work at their own pace, revisiting challenging sections as needed without feeling rushed. This fosters independent study and improves retention of the material.

Benefits	Impact on Learning
Step-by-step instructions	Clarify complex concepts and ensure no step is skipped.
Multiple examples	Provide varied approaches to the same problem, aiding in better understanding.
Practice exercises	Allow learners to apply knowledge and improve problem-solving skills.
Visual aids	Help visualize spatial relationships and make abstract ideas more tangible.

Advanced Geometry Topics for Further Study

Once the foundational concepts of shapes, angles, and measurements are mastered, learners can explore more complex and specialized areas of mathematics. Advanced topics expand on basic principles and offer new perspectives on the relationships between different geometric figures. These subjects are essential for those looking to deepen their understanding and explore the subject in greater depth, whether for academic or professional purposes.

Key Areas for In-Depth Study

Here are several advanced areas that can enhance your mathematical knowledge:

• Topology – Focuses on properties of space that are preserved under continuous transformations, such as stretching or bending, without tearing. This topic is widely applied in both theoretical and practical fields.
• Non-Euclidean Geometry – Explores geometries that reject some of the postulates of Euclidean geometry, including spherical and hyperbolic geometries. These areas are crucial for understanding advanced theories in physics and astronomy.
• Projective Geometry – Studies the properties of geometric objects that remain invariant under projection. It is vital for fields such as computer graphics and perspective drawing.
• Fractal Geometry – Examines shapes and patterns that exhibit self-similarity at various scales. This area is especially useful in understanding natural structures like coastlines and clouds.
• Riemannian Geometry – Deals with curved spaces and is a central part of Einstein’s theory of general relativity. It has applications in both physics and advanced mathematics.

Applications of Advanced Topics

These advanced concepts not only expand theoretical knowledge but also have real-world applications:

• Computer Graphics – Non-Euclidean and projective geometries help in creating realistic visual representations in virtual environments.
• Quantum Mechanics – Riemannian and differential geometry are foundational in understanding the nature of space and time in quantum theories.
• Engineering – Understanding fractals and topology can improve designs for structures and systems that need to handle complex environmental conditions.
• Art and Design – Projective geometry is heavily used in creating perspective views and 3D modeling for visual arts and architecture.

Choosing the Right Geometry Resource

Selecting the ideal reference material is crucial when aiming to master mathematical concepts related to shapes, sizes, and spatial relationships. With numerous options available, it can be challenging to identify which materials will provide the most comprehensive support for learning. Whether you’re a student, teacher, or self-learner, the right choice can significantly enhance your understanding and problem-solving skills.

Factors to Consider When Choosing a Resource

Here are several important factors to keep in mind while selecting your study guide:

• Clear Explanations – Look for resources that present concepts in a simple, easy-to-understand manner, with step-by-step explanations that guide you through problem-solving processes.
• Comprehensive Coverage – Ensure that the material covers a broad range of topics, from basic principles to more advanced concepts, to provide a complete understanding of the subject.
• Examples and Practice Problems – The best references include numerous worked examples and exercises, allowing you to apply the learned concepts and reinforce your skills.
• Visual Aids – Geometric problems often benefit from visual representations. Choose resources that include diagrams, charts, and other visual tools to aid in understanding abstract concepts.
• Structured Layout – A well-organized guide helps to break down complex topics into digestible sections, making it easier to follow along and progressively build your knowledge.

Types of Resources Available

When selecting a study aid, consider the following types of materials:

• Textual Guides – These offer detailed explanations, theories, and formulas with structured lessons. Ideal for those who prefer to read and absorb information at their own pace.
• Interactive Tools – Online resources or apps that include quizzes, tutorials, and instant feedback, offering a more hands-on approach to learning.
• Visual Resources – Books or online platforms that focus heavily on illustrations, diagrams, and step-by-step visual guides for solving problems.
• Practice Worksheets – Collections of problems with solutions that allow learners to practice skills and track their progress.

By taking into account these factors, you can choose a guide that aligns with your learning style and educational needs, ultimately enhancing your proficiency and confidence in the subject.

Improving Geometry Skills with Practice Exercises

Consistent practice is one of the most effective ways to enhance your understanding and proficiency in spatial reasoning and mathematical concepts. By working through a variety of exercises, learners can solidify their grasp on key principles, sharpen problem-solving abilities, and improve their overall confidence in tackling complex tasks. Regular practice is essential not only for reinforcing what has been learned but also for identifying areas that require further attention.

Benefits of Regular Practice

Engaging with exercises regularly offers multiple advantages for improving your skills:

• Reinforces Concepts: Repetition of problems helps to internalize the concepts, making them more intuitive and easier to apply in different scenarios.
• Increases Speed: The more problems you work on, the quicker you’ll be able to identify solutions, which is especially beneficial during timed exams or assessments.
• Builds Confidence: Successfully solving a range of problems boosts self-assurance, encouraging further exploration and learning.
• Prepares for Complex Problems: Through practice, you’ll be exposed to more challenging questions that will help you develop advanced skills necessary for handling intricate tasks.

Effective Approaches to Practice

To maximize the benefits of practice exercises, it’s important to approach them strategically:

• Start Simple: Begin with basic problems to ensure you have a strong understanding of foundational concepts before progressing to more complex topics.
• Mix Problem Types: Work on a variety of problems that cover different areas to ensure comprehensive learning and adaptability.
• Set Time Limits: Simulating exam conditions by setting time limits for exercises can help improve your speed and efficiency.
• Review Mistakes: After completing each exercise, take time to review any mistakes and understand why they happened. This will help you avoid repeating them in the future.

By incorporating practice exercises into your study routine and applying these strategies, you’ll significantly improve your skills and deepen your understanding of key concepts.

The Role of Geometry in Problem Solving

Mathematical reasoning plays a crucial role in approaching and resolving a wide range of problems, and spatial relationships are central to this process. The ability to visualize and manipulate shapes, sizes, and the relationships between objects is key not only to academic success but also to solving real-world challenges. Understanding the principles of angles, areas, and volumes provides a solid foundation for logical thinking and problem-solving strategies that extend far beyond the classroom.

Application in Everyday Life: Everyday scenarios, from architecture to navigation, require a deep understanding of spatial reasoning. Whether designing a building, creating a map, or organizing an event, knowledge of how different elements fit together and interact is essential. By applying the concepts learned in mathematical exercises, individuals can make more informed decisions, analyze structures more effectively, and approach tasks from a more organized, logical perspective.

Fostering Critical Thinking: The challenges posed by complex problems help develop critical thinking skills. When solving spatial problems, one learns to break down tasks into smaller, manageable parts, which fosters a disciplined thought process. These skills can then be transferred to other domains, such as scientific research, engineering, or even social problem-solving, where structured thinking is paramount.

Problem-Solving Techniques: Through structured exercises, learners develop methods of approaching problems step by step. Some common techniques include:

• Drawing Diagrams: Visual representations often provide clarity, helping to understand the problem more fully and revealing hidden relationships between elements.
• Using Logical Sequences: Understanding how one step leads to the next is critical in solving problems in a systematic way. Following a logical flow ensures that each part of the solution is based on sound reasoning.
• Trial and Error: In many cases, testing different approaches can lead to breakthroughs. This trial-and-error process helps build resilience and adaptability in problem-solving.

In conclusion, spatial reasoning and mathematical skills empower individuals to solve problems effectively by allowing them to approach challenges with clarity and confidence. These abilities are invaluable not only in academics but in a wide range of practical applications.

Geometry Answer Resources for Students

Accessing accurate solutions and explanations can greatly enhance a student’s ability to understand complex mathematical concepts. With the right tools and materials, learners can approach problems with greater confidence and improve their performance in mathematical exercises. There are various methods available to help students gain a deeper understanding of spatial reasoning and develop strong problem-solving skills.

Types of Available Resources

Several types of materials are available that provide comprehensive support for students seeking help with spatial reasoning tasks. These resources include online platforms, interactive tutorials, and traditional reference materials. Below are some commonly used options:

• Online Problem Solvers: Many websites offer step-by-step solutions, which can help students break down complex problems into manageable parts.
• Tutorial Videos: Visual learning through videos can be an effective way to grasp difficult concepts, as they allow students to see the problem-solving process in action.
• Practice Sheets: Printable worksheets provide additional opportunities for practice, reinforcing learned concepts and improving skills.

How to Choose the Right Support Tools

When selecting resources, it is important for students to consider what type of support will be most beneficial to their learning style. Below is a table highlighting key factors to consider when choosing the appropriate support tools:

Type of Resource	Advantages	Best For
Interactive Websites	Instant feedback, step-by-step solutions	Visual learners, those who need quick clarification
Video Tutorials	In-depth explanations, real-time demonstrations	Students who benefit from seeing concepts in action
Practice Exercises	Reinforce learning through repetition, self-paced	Students who need more practice to build confidence

By utilizing the right combination of these tools, students can strengthen their understanding and improve their ability to solve problems in this area of study. Consistent use of effective support resources leads to better retention and mastery of concepts.

Tips for Mastering Geometry with Resources

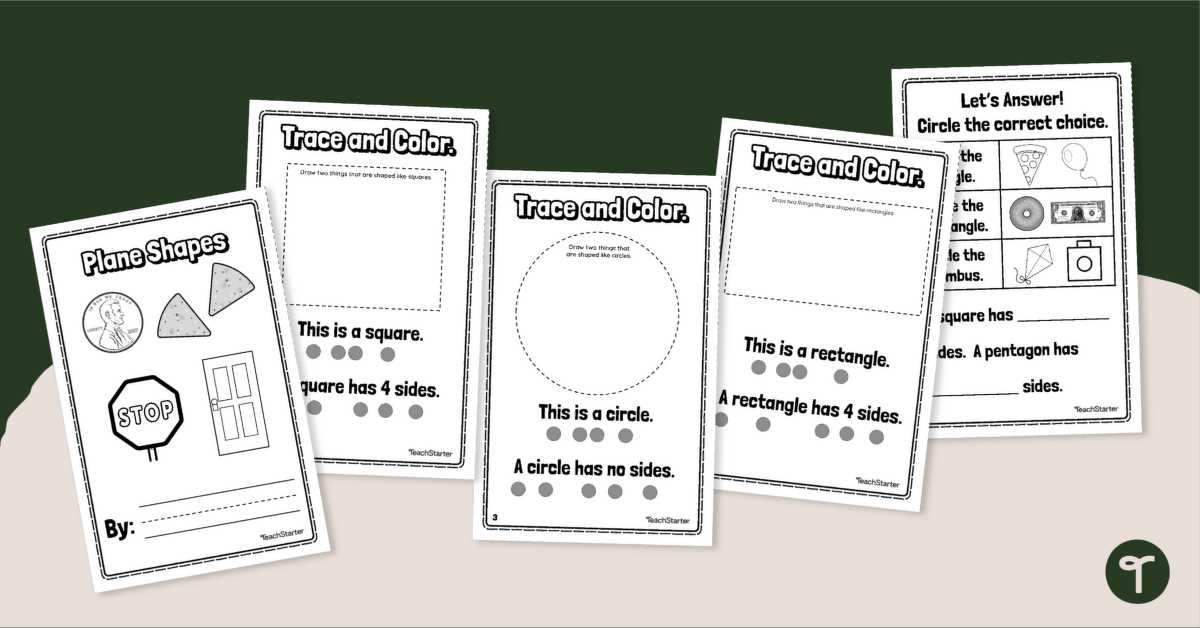
Developing proficiency in mathematical concepts, particularly those involving shapes and space, requires consistent practice and the right tools. With the right learning aids, students can break down complex concepts, clarify doubts, and reinforce their understanding. The following strategies can help learners make the most of available materials to strengthen their skills in this field.

Utilize a Variety of Learning Tools

Exploring different kinds of learning aids can provide multiple perspectives on the same concept. By using diverse resources, students can enhance their understanding and improve problem-solving abilities.

• Interactive Platforms: These tools provide dynamic ways to explore concepts, allowing students to visualize problems and solutions step-by-step.
• Printed Materials: Physical exercises such as worksheets and practice problems allow students to apply concepts independently, reinforcing knowledge through repetition.
• Video Tutorials: Watching experts solve problems in real-time can clarify complex methods and make abstract ideas more tangible.

Focus on Key Problem-Solving Techniques

Mastering fundamental problem-solving strategies is essential to improving skills. Whether you’re solving equations, calculating areas, or working with angles, understanding core methods will make each task easier.

• Breaking Down Problems: Instead of tackling a problem as a whole, break it into smaller, manageable parts. This simplifies even the most complicated questions.
• Practice Regularly: Consistency is key. Solve problems daily, even those that seem simple, to reinforce concepts and improve your ability to solve increasingly complex problems.
• Seek Explanation: If a solution doesn’t make sense, seek an explanation. Sometimes it’s about understanding the “why” behind the solution, not just the “how”.

By incorporating these strategies into their study routine, students can improve their confidence and performance in mathematical tasks, ultimately mastering the subject more effectively.

Exploring Geometry Solutions for Real-World Problems

Mathematics, particularly the study of shapes, sizes, and spatial relationships, plays a crucial role in solving everyday challenges. From architecture to engineering, and even in nature, concepts from this field are applied to address practical issues and design solutions. This section explores how such principles are used to find solutions in real-world situations, making abstract ideas more tangible and applicable.

Applications in Architecture and Design

Architects and designers rely on mathematical principles to create structures that are both functional and aesthetically pleasing. By using geometric concepts such as symmetry, proportions, and angles, they ensure stability and visual harmony in buildings and other structures.

Application	Geometric Concept Used	Benefit
Building Foundations	Area, Perimeter	Ensures the stability and balance of the structure.
Roof Design	Triangles, Angles	Improves durability and water drainage efficiency.
Interior Layout	Symmetry, Proportions	Creates functional, balanced, and visually appealing spaces.

Problem-Solving in Engineering and Technology

In engineering, geometric principles help design efficient machines, tools, and systems. Whether in creating curved surfaces for aerodynamics or determining the most efficient use of space in machinery, geometry is essential for ensuring precision and functionality in technological innovations.

• Efficient Machine Design: Engineers use geometric concepts to determine the optimal shape for various parts, improving efficiency and minimizing waste.
• Aerodynamics: In designing vehicles, aircraft, or rockets, geometric calculations help minimize drag and maximize speed and fuel efficiency.
• Structural Engineering: The study of forces and materials uses geometric principles to create buildings and bridges that are both safe and cost-effective.

Incorporating mathematical concepts into real-world scenarios not only helps solve immediate challenges but also fosters innovation, demonstrating the practical value of these ideas in daily life.