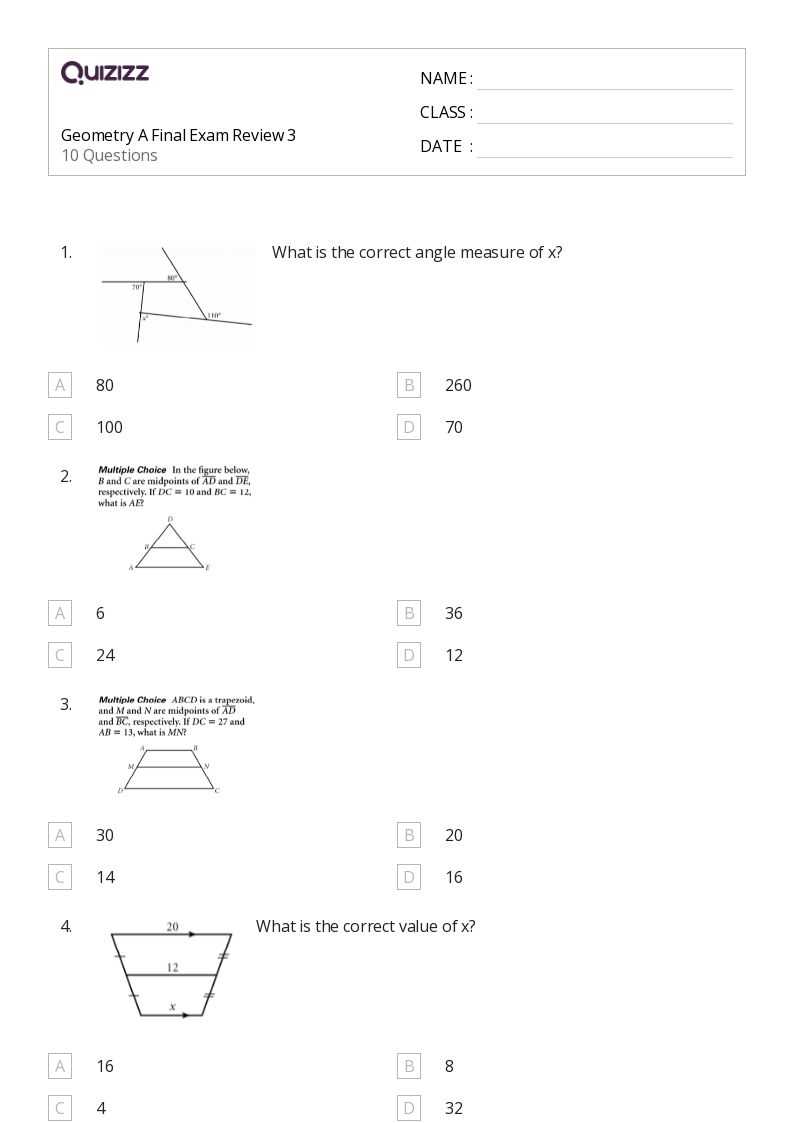
Preparing for an important assessment in mathematics can be challenging, but with the right strategies, it becomes an achievable goal. Understanding the core principles, practicing various techniques, and being able to approach problems confidently are key to excelling in this subject. Whether you’re revisiting fundamental theories or tackling advanced problems, thorough preparation is essential for success.
Knowing the right methods for solving problems, along with familiarizing yourself with common mistakes, will help you avoid pitfalls and perform at your best. Each question has its own structure, and understanding how to break down complex concepts into manageable steps will make a significant difference. By practicing and refining your skills, you can approach any problem with clarity and precision.
Stay focused on applying logical reasoning and mathematical concepts in real-world scenarios. Regular practice and review will not only solidify your understanding but also improve your speed and accuracy when solving problems. A strong grasp of essential tools and techniques is the foundation of mastering the material effectively.
Geometry Exam Review Answers
Preparing for a mathematics assessment requires understanding core principles, practicing key techniques, and refining problem-solving skills. Success is built on a foundation of logical thinking, systematic approaches, and familiarity with various types of questions. Mastering the essential concepts will help in navigating the test confidently and efficiently.
Key Concepts to Focus On
Focusing on these critical topics will provide a solid foundation for tackling a wide range of questions:
- Basic Shapes and Properties – Understanding the characteristics and relationships of shapes such as triangles, quadrilaterals, and circles is fundamental.
- Measurement and Calculation – Knowing how to calculate areas, perimeters, volumes, and angles is vital for solving many problems.
- Transformation and Symmetry – Recognizing various transformations like rotation, reflection, and translation is essential for problem-solving.
- Coordinate Systems – Mastery of graphing and solving equations on the coordinate plane will be crucial for answering many questions.
Effective Problem-Solving Strategies
To solve problems efficiently, consider these approaches:
- Read the Problem Carefully – Pay attention to the details, including units and specific instructions, to avoid making mistakes.
- Identify What You Need to Find – Understanding the goal of the question helps in choosing the right approach.
- Apply Relevant Formulas – Ensure you are using the correct formulas and techniques based on the problem type.
- Check Your Work – Double-check your calculations and reasoning to catch any errors before finalizing your answers.
Geometry Exam Review Answers
Preparing for a mathematics assessment requires understanding core principles, practicing key techniques, and refining problem-solving skills. Success is built on a foundation of logical thinking, systematic approaches, and familiarity with various types of questions. Mastering the essential concepts will help in navigating the test confidently and efficiently.
Key Concepts to Focus On
Focusing on these critical topics will provide a solid foundation for tackling a wide range of questions:
- Basic Shapes and Properties – Understanding the characteristics and relationships of shapes such as triangles, quadrilaterals, and circles is fundamental.
- Measurement and Calculation – Knowing how to calculate areas, perimeters, volumes, and angles is vital for solving many problems.
- Transformation and Symmetry – Recognizing various transformations like rotation, reflection, and translation is essential for problem-solving.
- Coordinate Systems – Mastery of graphing and solving equations on the coordinate plane will be crucial for answering many questions.
Effective Problem-Solving Strategies
To solve problems efficiently, consider these approaches:
- Read the Problem Carefully – Pay attention to the details, including units and specific instructions, to avoid making mistakes.
- Identify What You Need to Find – Understanding the goal of the question helps in choosing the right approach.
- Apply Relevant Formulas – Ensure you are using the correct formulas and techniques based on the problem type.
- Check Your Work – Double-check your calculations and reasoning to catch any errors before finalizing your answers.
How to Tackle Geometry Word Problems
When faced with challenges that require understanding spatial relationships, it’s essential to break the problem into manageable parts. Focus on interpreting the given details, translating them into meaningful information, and applying logical steps to arrive at a solution. This approach helps transform complex scenarios into solvable tasks by identifying key elements and their connections.
Start by carefully reading the problem to grasp the context. Look for clues such as dimensions, angles, or positions, which will help you visualize the situation. Drawing a diagram is often beneficial, as it allows you to see relationships between different elements clearly. Once you have a visual representation, use relevant formulas and properties to guide your calculations.
It’s important to remain organized and systematic. If the problem involves multiple steps, solve each one individually while ensuring each step builds upon the previous one. Don’t hesitate to review your work to ensure that every detail has been addressed correctly. This methodical approach leads to more accurate solutions and reduces the chances of errors.
Geometry Formulas You Must Remember
Mastering essential equations is key to solving various spatial problems quickly and accurately. Certain formulas are foundational, forming the building blocks for more complex challenges. By internalizing these relationships, you can navigate through problems with greater efficiency and confidence.
Key Formulas for Basic Shapes
Understanding the formulas for common shapes such as circles, triangles, and rectangles is crucial for many tasks. These equations allow you to calculate areas, perimeters, and other key measurements that serve as the foundation for more intricate problems.
| Shape | Formula |
|---|---|
| Circle | Area = πr², Perimeter = 2πr |
| Rectangle | Area = length × width, Perimeter = 2(length + width) |
| Triangle | Area = ½ × base × height, Perimeter = a + b + c |
Advanced Formulas for Complex Figures
As problems increase in complexity, you’ll need more advanced relationships to find solutions. These include volume calculations for three-dimensional objects and the application of Pythagorean theorem for right triangles. Familiarizing yourself with these formulas is essential when tackling higher-level questions.
| Shape | Formula |
|---|---|
| Sphere | Volume = 4/3πr³, Surface Area = 4πr² |
| Cylinder | Volume = πr²h, Surface Area = 2πr² + 2πrh |
| Pythagorean Theorem | a² + b² = c² |
Step-by-Step Guide to Solving Problems
Breaking down complex tasks into manageable steps is the key to finding accurate solutions. A structured approach helps ensure that each aspect of the problem is addressed and that the process remains clear and organized. By following a logical sequence, you can avoid confusion and make steady progress toward the correct answer.
To start, it’s important to carefully read the problem and identify the given data. Once the details are clear, choose the appropriate method or strategy to apply. Carefully perform calculations or logic, step by step, and always check your work to avoid mistakes. Finally, review the result to ensure everything aligns with the original question.
| Step | Action |
|---|---|
| 1 | Understand the problem by reading it carefully and identifying key details. |
| 2 | Organize the information you have and decide what needs to be found. |
| 3 | Choose the appropriate strategy or formula to solve the problem. |
| 4 | Carry out the necessary steps and calculations, ensuring each one is accurate. |
| 5 | Double-check the solution to confirm that all aspects of the problem have been addressed. |
Common Mistakes to Avoid in Geometry
When working through problems involving spatial relationships and measurements, it’s easy to make certain errors that can lead to incorrect solutions. These mistakes often stem from overlooking important details, misapplying formulas, or rushing through calculations. Being aware of these common pitfalls can help ensure accuracy and improve problem-solving skills.
One frequent mistake is misinterpreting the given information, such as confusing dimensions or forgetting to account for all variables. Another common error is neglecting to properly apply formulas, either by using the wrong one or forgetting to incorporate all necessary components. Additionally, errors in drawing diagrams or visualizing the problem can result in misunderstanding the relationships between different elements. Finally, it’s essential to double-check calculations, as simple math errors can easily throw off an entire solution.
Practice Questions for Review
Practicing with various types of questions helps solidify understanding and build confidence in solving problems. Working through a variety of scenarios enables you to apply concepts in different contexts, ensuring that you’re well-prepared for any challenge. Below are some practice questions designed to help reinforce key principles and test your skills.
Basic Questions
- Calculate the area of a rectangle with a length of 8 cm and a width of 5 cm.
- Find the perimeter of a triangle with sides measuring 7 cm, 9 cm, and 12 cm.
- Determine the circumference of a circle with a radius of 6 cm.
Intermediate Questions
- A right triangle has legs measuring 4 cm and 3 cm. Find the length of the hypotenuse.
- Calculate the area of a trapezoid with bases measuring 10 cm and 6 cm, and a height of 5 cm.
- Find the volume of a cylinder with a radius of 3 cm and a height of 10 cm.
Advanced Questions
- Determine the surface area of a sphere with a radius of 7 cm.
- Find the area of a sector with a central angle of 60 degrees and a radius of 5 cm.
- Calculate the volume of a cone with a radius of 4 cm and a height of 9 cm.
Time Management Strategies for Geometry Exams
Effective time management is essential when tackling complex problem-solving sessions. Properly allocating your time ensures that you can address all parts of a test while maintaining accuracy and reducing stress. By approaching each task with a clear strategy, you can maximize your efficiency and complete the test with confidence.
Start by quickly reviewing the entire set of problems to get a sense of their difficulty and length. Identify questions that seem straightforward and allocate more time to those that appear more challenging. Divide the available time into blocks, making sure to leave a few minutes at the end for review. This approach allows you to stay on track and avoid spending too much time on a single question.
Another important strategy is to prioritize questions that you know how to solve quickly. Don’t get bogged down by questions that seem unfamiliar or complex at first glance. Instead, move on to those that you feel confident about, and return to the more difficult ones later with a fresh perspective. Finally, practicing under timed conditions before the actual session helps you get accustomed to the time pressure and refine your pacing.
Visualizing Problems Effectively
Being able to visualize a problem is one of the most powerful tools when solving spatial challenges. A clear mental image can help identify relationships, simplify calculations, and guide the solution process. By turning abstract descriptions into concrete diagrams or models, you can gain a better understanding of the problem and approach it with more confidence.
Drawing Diagrams and Figures
When faced with a problem, start by sketching a diagram or figure to represent the information given. This allows you to see the problem from a different perspective and often makes it easier to identify missing pieces or patterns. Even if the problem doesn’t explicitly ask for a diagram, creating one can clarify the relationships between elements, such as angles, sides, or distances.
Using Visualization Strategies
In addition to drawing, another effective strategy is to break down the problem into smaller, manageable parts. Visualizing each part individually and then combining them can help solve more complex scenarios. For example, when working with multi-step problems, you can visualize each stage of the process separately, making it easier to follow through with the solution. This method ensures that every element of the problem is accounted for and makes tackling difficult questions much more manageable.
Visualizing Problems Effectively
Being able to visualize a problem is one of the most powerful tools when solving spatial challenges. A clear mental image can help identify relationships, simplify calculations, and guide the solution process. By turning abstract descriptions into concrete diagrams or models, you can gain a better understanding of the problem and approach it with more confidence.
Drawing Diagrams and Figures
When faced with a problem, start by sketching a diagram or figure to represent the information given. This allows you to see the problem from a different perspective and often makes it easier to identify missing pieces or patterns. Even if the problem doesn’t explicitly ask for a diagram, creating one can clarify the relationships between elements, such as angles, sides, or distances.
Using Visualization Strategies
In addition to drawing, another effective strategy is to break down the problem into smaller, manageable parts. Visualizing each part individually and then combining them can help solve more complex scenarios. For example, when working with multi-step problems, you can visualize each stage of the process separately, making it easier to follow through with the solution. This method ensures that every element of the problem is accounted for and makes tackling difficult questions much more manageable.
Strategies for Memorizing Geometry Terms
Effective memorization techniques can significantly improve recall and understanding of essential concepts. Using a combination of visual aids, association methods, and active engagement, one can enhance retention of key terminology and definitions. It’s important to employ strategies that cater to both short-term and long-term memory retention, ensuring concepts are not only learned but also easily recalled when needed.
One useful approach is creating flashcards with terms on one side and definitions or diagrams on the other. This method promotes active recall, which strengthens memory. Additionally, grouping related terms together can help form mental connections, making it easier to remember complex ideas. For example, associating different shapes with real-world objects can provide context and make terms more relatable.
Another effective technique is the use of mnemonic devices. Creating acronyms or simple rhymes can simplify difficult terms. For instance, breaking down complex words into smaller parts or using words that sound similar can create associations that are easier to recall. Furthermore, drawing diagrams and labeling them can reinforce understanding, helping to visualize how different elements relate to each other.
Working with Geometry Diagrams and Figures
Mastering visual representations of shapes and structures is crucial for solving problems effectively. Diagrams serve as powerful tools, providing clear illustrations that help understand spatial relationships and properties. By carefully analyzing these figures, one can identify patterns, apply formulas, and enhance problem-solving accuracy.
Interpreting Key Elements in Diagrams
To fully grasp the information presented in a figure, focus on the key components. Labels, angles, and lines often hold essential clues for understanding the problem. Pay close attention to markings that indicate equal lengths or angles, as these are often central to the solution. Recognizing symmetry and special properties, like parallelism or perpendicularity, can simplify complex tasks.
Practical Approaches for Drawing and Annotating
When working with drawings, it’s helpful to recreate the figure with precision. Using a ruler and compass can ensure accuracy, while annotations such as angle measures or segment lengths provide clarity. Labeling parts of the figure during problem-solving helps keep track of crucial information and can prevent mistakes. Taking a methodical approach to sketching out shapes and figures is essential for approaching problems with confidence.
How to Use Geometry Review Worksheets
Worksheets are valuable resources for reinforcing key concepts and ensuring thorough understanding of fundamental ideas. By actively engaging with these exercises, individuals can identify areas of strength while addressing areas that need improvement. A well-structured worksheet helps in practicing different types of problems, which contributes to building confidence and proficiency.
Organizing Your Approach
Start by reviewing the instructions for each set of problems. Focus on the types of tasks provided, whether they involve calculations, reasoning, or identifying patterns. Break down each problem into smaller steps, ensuring that you understand what is being asked before attempting a solution. Work through problems sequentially, and if you encounter difficulty, go back and study the related concepts before trying again.
Effective Strategies for Practice
To maximize the benefit of each worksheet, it’s important to simulate timed conditions once you feel comfortable with the material. This will help you improve speed and accuracy under pressure. Additionally, consider reviewing the completed worksheet with a teacher, tutor, or peer to discuss any errors and reinforce the learning process. This collaborative approach can provide insight into different methods of solving problems, enhancing your overall grasp of the material.
How to Use Geometry Review Worksheets
Worksheets are valuable resources for reinforcing key concepts and ensuring thorough understanding of fundamental ideas. By actively engaging with these exercises, individuals can identify areas of strength while addressing areas that need improvement. A well-structured worksheet helps in practicing different types of problems, which contributes to building confidence and proficiency.
Organizing Your Approach
Start by reviewing the instructions for each set of problems. Focus on the types of tasks provided, whether they involve calculations, reasoning, or identifying patterns. Break down each problem into smaller steps, ensuring that you understand what is being asked before attempting a solution. Work through problems sequentially, and if you encounter difficulty, go back and study the related concepts before trying again.
Effective Strategies for Practice
To maximize the benefit of each worksheet, it’s important to simulate timed conditions once you feel comfortable with the material. This will help you improve speed and accuracy under pressure. Additionally, consider reviewing the completed worksheet with a teacher, tutor, or peer to discuss any errors and reinforce the learning process. This collaborative approach can provide insight into different methods of solving problems, enhancing your overall grasp of the material.
How to Use Geometry Review Worksheets
Worksheets are valuable resources for reinforcing key concepts and ensuring thorough understanding of fundamental ideas. By actively engaging with these exercises, individuals can identify areas of strength while addressing areas that need improvement. A well-structured worksheet helps in practicing different types of problems, which contributes to building confidence and proficiency.
Organizing Your Approach
Start by reviewing the instructions for each set of problems. Focus on the types of tasks provided, whether they involve calculations, reasoning, or identifying patterns. Break down each problem into smaller steps, ensuring that you understand what is being asked before attempting a solution. Work through problems sequentially, and if you encounter difficulty, go back and study the related concepts before trying again.
Effective Strategies for Practice
To maximize the benefit of each worksheet, it’s important to simulate timed conditions once you feel comfortable with the material. This will help you improve speed and accuracy under pressure. Additionally, consider reviewing the completed worksheet with a teacher, tutor, or peer to discuss any errors and reinforce the learning process. This collaborative approach can provide insight into different methods of solving problems, enhancing your overall grasp of the material.