In this section, we explore essential principles that form the foundation of mathematical problem-solving in the third unit of your curriculum. The focus is on equipping learners with the tools necessary to tackle complex exercises through systematic approaches.
Whether you’re working through exercises on your own or reviewing with a study group, it’s important to break down each task into manageable steps. By doing so, you can confidently address even the most challenging questions. Understanding the underlying techniques will greatly enhance your ability to solve problems accurately and efficiently.
Effective strategies and clear reasoning are central to mastering this material. By reviewing the solutions and reasoning behind each step, you’ll gain deeper insights into how to approach similar problems in the future.
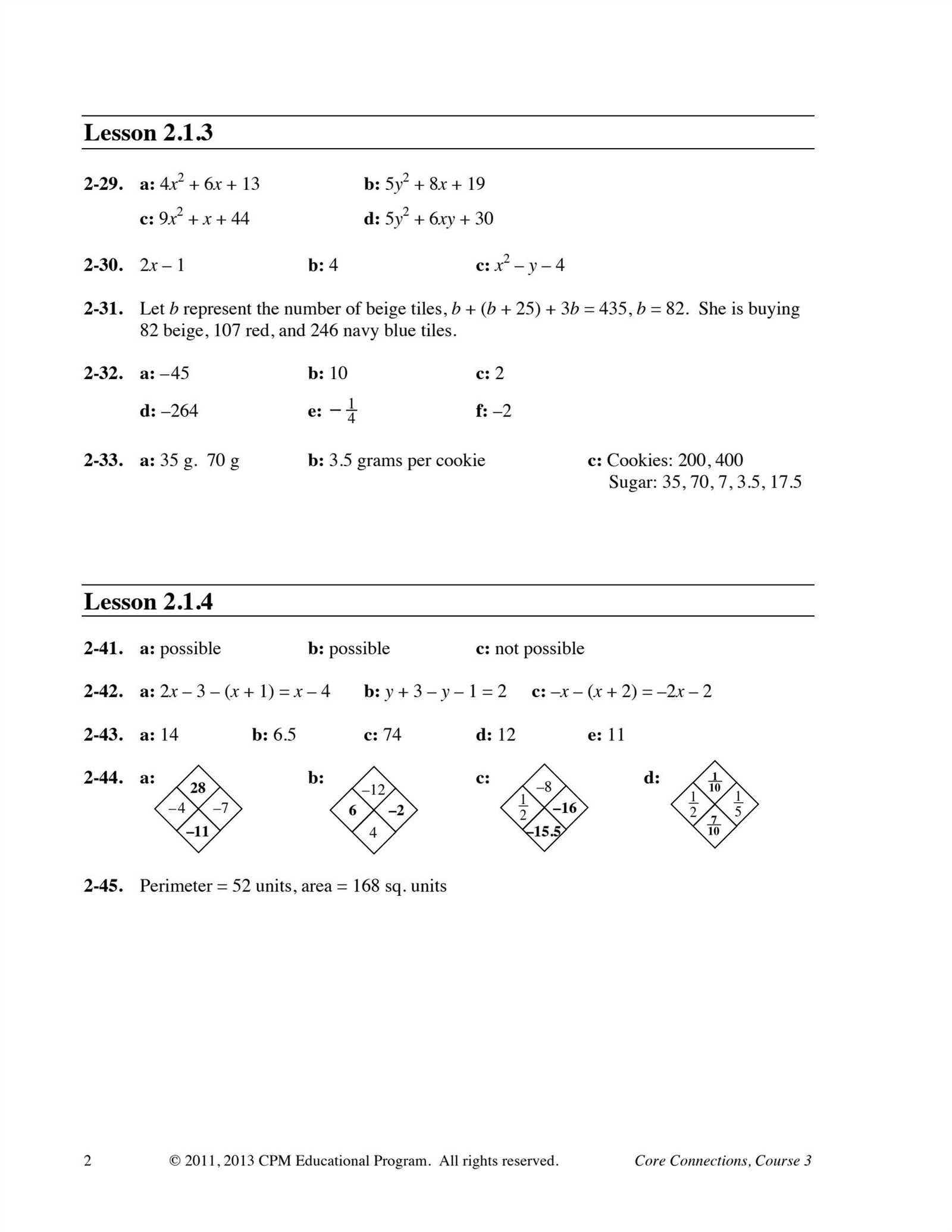
Core Connections Course 3 Chapter 3 Answers
This section provides the necessary explanations and solutions to the problems presented in the third unit. It aims to clarify the concepts and methods that are crucial for solving the exercises effectively.
By following a step-by-step approach, you’ll be able to grasp the logical flow behind each problem and understand how to reach the correct outcomes. These solutions are designed to build a strong foundation for tackling increasingly complex tasks.
Reviewing each solution helps reinforce key skills, ensuring that you’re well-prepared for future challenges. With a clear understanding of the processes involved, you’ll be able to approach similar problems with confidence and precision.
Understanding Key Concepts in Chapter 3
This section focuses on the foundational ideas that are essential for solving the problems in the third unit. It covers the core methods and principles that will allow you to approach each task with clarity and precision.
Mastering Problem-Solving Techniques
To succeed in this unit, it’s important to first understand the core principles behind the problems. Recognizing patterns, breaking down complex tasks into simpler steps, and applying the right strategies are key to finding accurate solutions.
Critical Thinking and Application
Each problem requires not only technical knowledge but also the ability to think critically and apply your understanding to new situations. By strengthening your problem-solving skills, you will gain confidence and improve your overall performance.
How to Approach Course 3 Problems
When faced with a series of complex tasks, it is essential to develop an effective strategy for tackling each one. A structured approach allows you to break down each question into smaller, more manageable parts, helping you achieve accurate results efficiently.
Step-by-Step Problem Solving
Follow these steps to approach the exercises with confidence:
- Understand the Problem: Carefully read the problem statement and identify the key information.
- Break it Down: Simplify the task by dividing it into smaller steps or sections.
- Choose the Right Method: Select the appropriate technique or formula to solve the problem.
- Work Through the Steps: Apply the chosen method systematically, ensuring accuracy at each stage.
- Check Your Answer: Review the final result to verify it makes sense in the context of the problem.
Common Challenges and Solutions
While working through exercises, you may encounter challenges. Here are some common issues and tips for overcoming them:
- Misunderstanding the Question: Reread the problem carefully and highlight key terms to ensure you understand the task.
- Forgetting Important Steps: Double-check each step and make sure nothing is overlooked.
- Using the Wrong Formula: Revisit the concepts and ensure you are applying the correct method for the problem type.
Step-by-Step Solutions for Chapter 3
This section provides detailed instructions for solving the problems from the third unit. By breaking down each exercise into manageable steps, you can better understand the reasoning behind each solution and gain clarity on the methods used to arrive at the correct answers.
Follow the outlined procedures carefully to ensure a full grasp of the concepts and to develop a more effective problem-solving approach.
| Step | Description |
|---|---|
| Step 1 | Read the problem thoroughly and identify the key information provided. |
| Step 2 | Break down the task into smaller parts to simplify the process. |
| Step 3 | Select the appropriate technique or formula to solve the problem. |
| Step 4 | Work through the problem methodically, ensuring you don’t skip any steps. |
| Step 5 | Review the final solution to verify its accuracy and relevance to the original question. |
Common Mistakes in Chapter 3 Exercises
While working through the exercises in the third unit, students often make certain errors that can lead to incorrect solutions. Recognizing these common pitfalls is crucial to avoiding them and ensuring accurate results. By understanding where mistakes typically occur, you can sharpen your problem-solving skills and improve your overall performance.
Frequent Errors and How to Avoid Them
Below are some of the most common mistakes that learners make when tackling tasks in this unit, along with tips on how to avoid them:
| Mistake | How to Avoid It |
|---|---|
| Misinterpreting the Problem | Read the problem carefully and highlight key information before starting. Ensure you understand what is being asked. |
| Skipping Steps | Work through the problem step by step and don’t skip any calculations or procedures, even if they seem obvious. |
| Using Incorrect Formulas | Ensure you select the correct formula or method based on the specific type of problem you are solving. |
| Rushing the Process | Take your time and double-check your work to avoid careless mistakes that can be easily corrected with careful attention. |
Improving Accuracy in Problem Solving
By being aware of these common mistakes, you can improve your problem-solving approach and avoid the errors that frequently hinder progress. Patience and practice will ultimately help you become more confident and accurate in tackling future exercises.
Practical Tips for Solving Core Connections
Successfully solving exercises requires a blend of strategy, focus, and careful application of learned principles. Developing a clear approach and following efficient methods can greatly improve the accuracy and speed with which you tackle each task. In this section, we outline practical tips that will help you navigate through the problems with confidence.
Organizing Your Approach
Start by breaking down each problem into smaller, manageable steps. This will allow you to focus on one element at a time, reducing the chances of feeling overwhelmed. Use a methodical approach to ensure that no step is skipped, and always double-check your work as you progress through each exercise.
Effective Time Management
Managing your time efficiently is essential when solving tasks. Set aside specific intervals to focus on each problem and avoid rushing. Give yourself time to think critically and evaluate your solutions carefully. If you find yourself stuck, take a break and return to the problem with a fresh perspective.
Analyzing Course Structure
Understanding the structure of the material is key to mastering any subject. The design of the learning path, from one concept to the next, is intended to build a strong foundation, helping students progressively grasp more complex ideas. By analyzing how the exercises and lessons are organized, you can better navigate through the content and apply your knowledge effectively.
The structure typically starts with basic concepts and gradually introduces more advanced topics. This ensures that you not only understand the fundamental principles but also develop the skills necessary to tackle more intricate problems. Recognizing the flow and connection between different sections will help you stay on track and approach each topic with clarity.
Breaking Down Problem Types
In this section, we will explore the different types of problems that you will encounter throughout this unit. By breaking them down into categories, you can better understand their structure and approach each one with a clear strategy. Identifying the type of problem is the first step toward choosing the most effective solution method.
Common Problem Categories
Problems in this unit can be grouped into several key categories. Here are the most common types:
- Arithmetic Operations: These problems involve basic calculations like addition, subtraction, multiplication, and division.
- Algebraic Expressions: Tasks that require simplifying or solving algebraic equations and expressions.
- Word Problems: Problems that present real-life scenarios, requiring the translation of the information into mathematical expressions.
- Geometric Reasoning: Questions that involve the application of geometry principles such as area, volume, and angles.
Approaching Each Problem Type
Each problem type requires a unique method for solving. Here are some tips for approaching them:
- Arithmetic Operations: Double-check your calculations to avoid simple errors. Break the problem into smaller parts if necessary.
- Algebraic Expressions: Isolate variables and apply proper algebraic rules. Don’t forget to simplify expressions step by step.
- Word Problems: Read the problem carefully, identify the knowns and unknowns, and translate the text into equations.
- Geometric Reasoning: Draw diagrams to visualize the problem. Use known formulas for area, perimeter, and volume when applicable.
Critical Thinking in Exercises
To succeed in solving problems, it’s essential to apply critical thinking skills. These exercises require not only a good understanding of the material but also the ability to analyze, evaluate, and apply concepts in creative ways. By developing these skills, you can approach problems with a deeper understanding and find solutions more effectively.
Critical thinking involves looking at problems from multiple angles, questioning assumptions, and considering all possible solutions before choosing the best approach. This mindset will help you move beyond memorization and towards true comprehension, enabling you to tackle complex challenges with confidence.
| Critical Thinking Skill | How to Apply It |
|---|---|
| Analysis | Break down problems into smaller, more manageable parts to understand the underlying structure. |
| Evaluation | Assess the validity of different approaches and weigh their potential outcomes before proceeding. |
| Creativity | Think outside the box and explore alternative methods for solving problems when traditional solutions are insufficient. |
| Reasoning | Use logical steps to build your solution, ensuring each step follows from the previous one in a coherent manner. |
Math Strategies for Chapter 3 Success
Mastering mathematical problems requires a combination of strategy, practice, and the ability to adapt to different types of challenges. By applying effective strategies, students can tackle even the most difficult exercises with confidence. In this section, we will explore several methods that will help ensure success in the problems you face in this unit.
Organize Your Work
One of the most effective strategies for solving math problems is to keep your work organized. Clearly write down each step as you go along, and make sure to label your work properly. This not only helps prevent mistakes but also allows you to easily trace back your steps if you need to check your reasoning.
Practice Mental Math
Developing strong mental math skills can significantly improve your efficiency. While working through exercises, try to solve simpler problems in your head before committing to paper. This will speed up the process and help you recognize patterns in problems more easily.
Reviewing Answer Keys for Accuracy
When solving mathematical problems, reviewing the solutions is just as important as solving them in the first place. Answer keys provide a reference for verifying your work, but it’s essential to critically assess them for accuracy. By carefully comparing your results to the provided solutions, you can identify mistakes and gain a deeper understanding of the process.
Why Verification is Important
Answer keys serve as tools to help confirm that you have followed the correct approach, but they can sometimes contain errors or may not explain the reasoning behind the solution. It’s important to not only compare the final result but also ensure that each step leading to the solution is logical and consistent with the concepts you’ve learned.
Steps for Reviewing Answer Keys
- Compare Step-by-Step: Don’t just look at the final answer–go through each step provided in the key and ensure that it matches your thought process.
- Double-Check Calculations: Confirm that all arithmetic and algebraic operations were carried out correctly in both your solution and the key.
- Look for Alternative Methods: If you find that the answer key uses a different method, try understanding it and see if it leads to a more efficient solution.
- Understand Mistakes: If you find an error in the answer key, analyze where the mistake occurred and learn from it to avoid similar errors in the future.
Key Learning Outcomes from Chapter 3
By engaging with the material in this section, students are expected to gain a deeper understanding of the fundamental concepts and techniques that form the foundation for more advanced problem-solving. The key learning outcomes focus on applying mathematical principles to a variety of scenarios and developing skills that can be used beyond this unit.
Building Analytical Skills
One of the main goals of this unit is to help students develop stronger analytical thinking. This includes breaking down complex problems into manageable steps and identifying patterns that can simplify calculations. The ability to approach problems methodically and with a clear process is an invaluable skill for academic success in mathematics.
Mastering Core Mathematical Concepts
Students will also gain a solid grasp of essential mathematical ideas, such as equations, functions, and numerical relationships. Mastering these concepts is crucial, as they provide the building blocks for understanding more sophisticated topics in later units. By achieving a clear understanding of the material, learners will be better equipped to apply these principles in practical and real-world contexts.
Best Practices for Chapter 3 Assignments
Completing assignments effectively requires a strategic approach, ensuring that each task is tackled with care and precision. By following best practices, students can maximize their understanding and improve the quality of their work. This section highlights key strategies to help students succeed in their assignments and develop strong problem-solving skills.
Planning Your Approach
- Read Instructions Carefully: Start by thoroughly reading the instructions to understand what is being asked. Clarify any doubts before beginning.
- Break Tasks into Smaller Steps: Divide larger problems into smaller, more manageable sections. This approach makes tackling complex problems less overwhelming.
- Manage Your Time: Allocate sufficient time for each assignment. Avoid cramming and ensure you can revisit your work to check for errors.
Review and Reflect
- Double-Check Your Work: After completing each task, take time to review your answers. Look for any mistakes or missed steps in your calculations.
- Seek Feedback: If possible, ask a peer or instructor to review your work. Feedback can help identify areas for improvement.
- Learn from Mistakes: If errors are found, reflect on why they occurred and what steps can be taken to avoid them in the future.
Using Resources for Effective Learning
To truly grasp complex concepts, students must take advantage of a variety of resources that enhance their understanding and reinforce key ideas. Whether through textbooks, online materials, or peer collaboration, utilizing available tools can lead to more efficient learning and greater academic success. This section explores how different resources can be leveraged to support and improve the learning process.
Textbooks and Supplementary Materials

Textbooks often provide a structured approach to learning, offering explanations, examples, and practice problems that help solidify your understanding. Supplementary materials, such as workbooks and online guides, can offer additional practice and deeper insights into challenging topics. Don’t overlook these valuable tools as they can offer a fresh perspective on difficult concepts.
Online Learning Platforms
Online resources such as video tutorials, educational websites, and interactive tools can be highly beneficial for reinforcing difficult topics. These platforms allow for self-paced learning, where students can revisit lessons, watch demonstrations, and complete interactive exercises. Engaging with multimedia content can enhance understanding and cater to different learning styles.
Collaborative Learning
Learning with peers can provide a unique opportunity to reinforce concepts through discussion and problem-solving. Group study sessions or online discussion forums allow students to clarify doubts, share insights, and tackle problems together. Working collaboratively can also help in understanding diverse approaches to solving problems, which can deepen your own understanding.
Exploring Alternative Problem-Solving Methods
Effective problem-solving often involves looking beyond traditional approaches and considering new methods that might offer a more efficient or creative solution. By exploring alternative techniques, students can expand their understanding and develop flexible strategies for tackling various challenges. This section highlights several methods that can be used to solve problems more effectively, offering diverse approaches to suit different learning styles.
Visualization and Diagrams
One powerful technique for solving complex problems is the use of visualization. Creating diagrams, flowcharts, or graphs can help break down intricate concepts into simpler, more understandable parts. By mapping out a problem visually, it becomes easier to identify patterns, relationships, and potential solutions that might not be immediately obvious through text-based methods alone.
Trial and Error Method
Trial and error involves testing different solutions until the correct one is found. While this method can be time-consuming, it encourages hands-on learning and can help build persistence. By experimenting with different approaches, students gain a deeper understanding of the problem and the reasoning behind each potential solution. This method can be especially useful in areas where multiple approaches may lead to correct outcomes.
Critical Thinking and Logical Reasoning
Critical thinking involves breaking down a problem into smaller, more manageable components and analyzing each part systematically. By using logic and reasoning, students can eliminate incorrect answers and narrow down possible solutions. This method is often effective when dealing with problems that require precise steps and a clear understanding of underlying principles.
Collaborative Problem Solving
Working with others to solve problems can bring fresh perspectives and diverse approaches. Collaborative problem-solving encourages discussion, idea sharing, and joint decision-making. In many cases, the combined knowledge of a group can lead to quicker, more innovative solutions, especially when different individuals bring expertise from various areas.
Building Confidence with Chapter 3 Problems
Overcoming challenging exercises and problems often starts with building a strong foundation of confidence. When students approach complex tasks with the right mindset, they are more likely to engage with the material, persist through difficulties, and ultimately succeed. This section provides strategies for boosting confidence and achieving better results with each problem set.
Start with Familiar Concepts
One effective way to gain confidence is by first focusing on problems that feel familiar. By revisiting concepts that you already understand well, you can reinforce your knowledge and ease into more difficult topics. Successfully solving these problems serves as a confidence boost and helps build momentum for tackling tougher exercises.
Break Down Problems into Manageable Steps
When faced with complex problems, it’s easy to feel overwhelmed. Breaking the problem down into smaller, more manageable steps makes it easier to approach. Focus on solving one part of the problem at a time rather than trying to solve everything at once. This method not only simplifies the task but also makes progress feel more achievable, increasing your confidence as you move forward.
Practice Regularly to Build Mastery
Confidence grows with practice. The more you work on similar problems, the more proficient you become at identifying patterns and applying solutions. Consistent practice helps reinforce key concepts and improves problem-solving speed, leading to greater self-assurance. It’s important to approach practice with patience and persistence, understanding that mastery comes over time.
Learn from Mistakes and Stay Positive
Making mistakes is a natural part of the learning process. Instead of letting errors discourage you, use them as opportunities for growth. Analyze where things went wrong, learn from your mistakes, and adjust your approach for the next attempt. Maintaining a positive attitude and viewing mistakes as learning experiences can foster resilience and boost your confidence in the long run.
Time Management for Core Connections Work
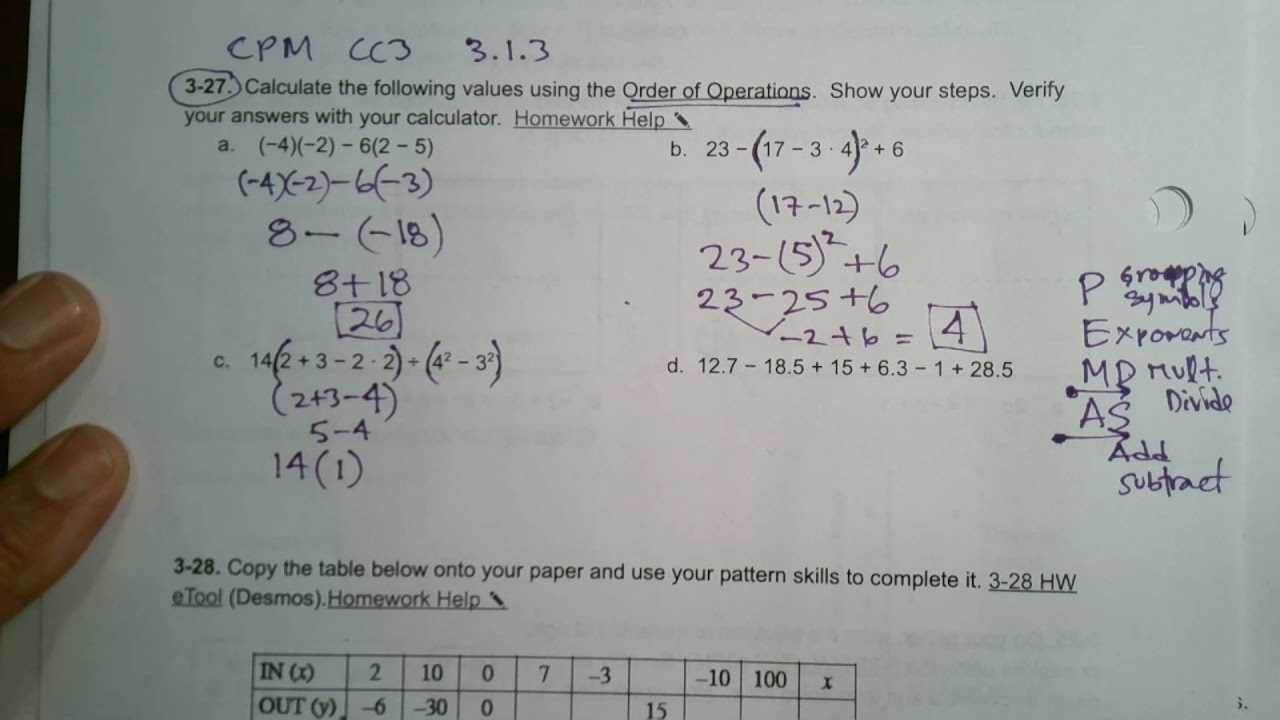
Effective time management is essential for tackling assignments and tasks efficiently. By allocating enough time for each section and maintaining a consistent pace, you can ensure that all problems are addressed thoughtfully without the stress of last-minute rushes. This section explores strategies to optimize your approach to work, balancing productivity with quality.
Prioritize Tasks Based on Difficulty
One way to manage your time effectively is by assessing the difficulty of each problem. Start with the ones you find easier or more familiar to gain confidence and build momentum. Once you’ve successfully completed the simpler tasks, shift focus to more challenging ones. This helps ensure that you make steady progress while not feeling overwhelmed by the more complex exercises.
Set Clear Deadlines and Breaks
Setting a clear timeline for each task can significantly boost your productivity. Break larger assignments into smaller, more manageable segments and assign deadlines for each part. This helps avoid procrastination and ensures you’re consistently moving forward. Be sure to schedule regular breaks to recharge your focus and energy, preventing burnout and maintaining a high level of performance.
Avoid Multitasking to Stay Focused
While it may seem like multitasking saves time, it can actually reduce your efficiency. Focusing on one task at a time allows for deeper concentration and better problem-solving. Turn off distractions, such as social media or background noise, and dedicate uninterrupted time to each assignment. This method will lead to more accurate and thoughtful results, and you’ll find that completing tasks is quicker than expected when done with full focus.
Track Your Progress and Adjust Plans
Tracking your progress allows you to assess how well you’re managing your time. After completing each section, take a moment to reflect on how long it took and whether adjustments need to be made. If certain types of problems take longer, consider allocating more time for them in the future. This ongoing self-assessment will help you fine-tune your approach and become more efficient over time.
Applying Core Connections to Real Life

The concepts explored in academic exercises can be incredibly useful when applied to everyday situations. By recognizing the real-world value of the skills developed through problem-solving and analytical thinking, students can bridge the gap between theory and practice. This section delves into how the techniques learned in the classroom can be translated into tangible, practical applications that enhance decision-making and efficiency in various aspects of life.
Problem-Solving in Everyday Situations
The ability to break down complex problems into manageable steps is a crucial skill. Here are a few examples of how you can apply problem-solving skills to daily activities:
- Financial Planning: Analyzing budgets, calculating expenses, and planning savings are all tasks that require logical reasoning and organization, similar to solving math problems.
- Home Improvement: When tackling projects like renovation or assembling furniture, breaking down tasks into a series of steps mirrors the methodical approach used in academic exercises.
- Decision Making: Whether choosing a career path or making a significant purchase, weighing options and considering pros and cons can be approached using the same decision-making processes.
Enhancing Efficiency and Time Management
Learning how to manage your time and approach tasks strategically can help you become more productive in both personal and professional settings. Some everyday applications include:
- Work Scheduling: Prioritize tasks based on deadlines or complexity, similar to organizing academic work.
- Multitasking Efficiency: By focusing on one task at a time, you can improve your ability to manage multiple responsibilities effectively.
- Setting Realistic Goals: Breaking long-term goals into smaller, achievable steps can help maintain motivation and track progress.
By applying these principles, you’ll find that skills developed in learning environments are not only useful for academic success but are also crucial for navigating everyday challenges. The ability to think critically, manage time, and solve problems can enhance both personal growth and professional development.