
Mathematics can be a challenging subject, especially when it comes to mastering complex concepts and solving intricate problems. Students often find themselves struggling with specific topics, searching for reliable ways to enhance their understanding and improve their performance. Whether you’re dealing with basic equations or more advanced calculations, having the right resources can make all the difference in achieving success.
One of the most effective approaches to learning is practicing regularly with accessible tools that provide step-by-step explanations and exercises. By breaking down each problem and following a structured method, it becomes easier to grasp even the most difficult material. As you gain more confidence, the process of learning mathematics becomes less daunting and more rewarding.
Utilizing available platforms designed to offer personalized help can help you build a solid foundation and address areas where you might need extra attention. These platforms offer valuable support, making it easier to tackle your homework and prepare for tests with greater efficiency.
Ple Platoweb Algebra 1 Answers
When it comes to tackling mathematical problems, finding reliable resources to guide you through complex tasks is essential. With the right tools, students can approach each problem with confidence and clarity, making it easier to understand the steps involved in solving them. These tools provide not just the correct solutions but also detailed explanations that help strengthen overall comprehension.
One valuable resource for students is an online platform that offers structured lessons and practice exercises. By using such a platform, learners can access immediate feedback and support for each problem, ensuring they are on the right track. This method promotes active learning and encourages deeper engagement with the material, making it easier to progress and master key concepts.
| Problem Type | Solution Approach | Explanation |
|---|---|---|
| Linear Equations | Solve for x using addition or subtraction | Begin by isolating the variable on one side of the equation |
| Quadratic Equations | Use factoring or the quadratic formula | Rewrite the equation in standard form, then apply the formula or factorization |
| Graphing | Plot points based on coordinates | Graph each equation by plotting the points and drawing the line or curve |
By using these resources effectively, students not only find answers to individual problems but also gain a broader understanding of the mathematical techniques involved. This method of guided practice helps reinforce critical thinking and problem-solving skills that will prove valuable in future studies.
How to Find Algebra 1 Solutions
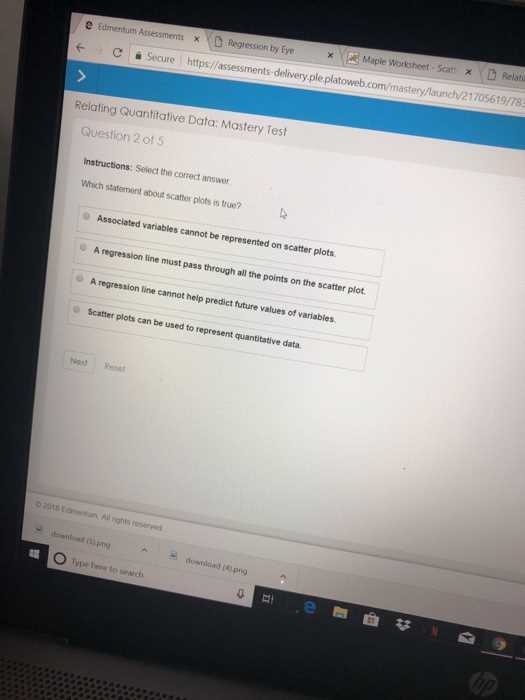
Successfully solving mathematical problems requires a strategic approach that helps break down each challenge into manageable steps. Whether you’re working with equations, graphs, or inequalities, understanding the right techniques and using the proper tools can significantly improve your problem-solving skills. By following a clear method, you can efficiently find the correct results and enhance your comprehension of the subject.
Key Strategies for Solving Problems
There are several effective techniques you can use to solve problems systematically:
- Identify the type of problem: Recognize whether you are dealing with linear equations, quadratic expressions, or other mathematical concepts.
- Isolate the variable: For most problems, the goal is to solve for the unknown. Begin by isolating the variable on one side of the equation.
- Use logical operations: Apply addition, subtraction, multiplication, or division to simplify the equation and solve for the unknown.
- Check your work: After solving, substitute your result back into the original equation to verify that it satisfies the conditions.
Using Online Resources for Practice
Leveraging digital platforms can provide instant feedback and step-by-step explanations, which are especially helpful when learning new techniques. These platforms allow you to practice a wide range of problems, ensuring that you can reinforce your understanding and identify any areas where you might need more attention.
- Interactive exercises: Many platforms offer exercises that adjust in difficulty based on your performance, helping you challenge yourself progressively.
- Detailed step-by-step guides: For each problem, look for resources that break down the steps involved in the solution, making complex concepts easier to grasp.
By using these strategies and resources, you will improve your ability to find solutions more efficiently and effectively. Consistent practice and application of the right methods are key to mastering any mathematical topic.
Understanding Key Algebraic Concepts
Mastering mathematical topics requires a solid understanding of the fundamental principles that form the foundation of the subject. By grasping core ideas, such as variables, equations, and functions, students can tackle more complex problems with confidence. These concepts not only appear frequently in problems but also serve as the building blocks for advanced learning in mathematics.
One of the most important concepts is the use of variables to represent unknown quantities. This allows for the formulation of equations that can be manipulated and solved to find specific values. Understanding how to work with variables and the operations that affect them is crucial for success in more advanced topics.
Another key area is the understanding of how functions represent relationships between different quantities. Recognizing how to interpret graphs, tables, and equations in terms of these relationships is essential. Functions provide a powerful way to model real-world situations, making them one of the most versatile tools in mathematics.
By focusing on these foundational principles, students can improve their problem-solving abilities and build a deeper understanding of the subject. With practice, these concepts will become more intuitive, allowing for easier navigation through both simple and complex tasks.
Common Algebra 1 Mistakes to Avoid
When learning mathematical concepts, it’s easy to make mistakes that can hinder your progress. These errors often stem from misunderstandings of the core principles or rushing through steps without fully grasping the process. Identifying common mistakes and knowing how to avoid them can significantly improve your accuracy and efficiency in solving problems.
Many learners struggle with basic operations, misapplying rules, or overlooking small details, which can lead to incorrect results. By understanding these common pitfalls, you can better navigate through challenges and build a stronger foundation for more advanced topics.
| Mistake | Cause | How to Avoid |
|---|---|---|
| Skipping steps | Rushing through the solution | Always write out each step, even if it seems simple |
| Sign errors | Misunderstanding the rules of negative numbers | Double-check the signs at every step, especially when adding or subtracting |
| Incorrect distribution | Forgetting to distribute terms correctly in equations | Review the distributive property and apply it carefully to each term |
| Confusing variable terms | Mixing up different variables or constants | Always clearly identify and separate variables and constants in your work |
By recognizing these common errors and being mindful of the steps involved, you can improve both the speed and accuracy of your work. Consistent practice and attention to detail are key to mastering any mathematical topic.
Effective Strategies for Algebra Success
Achieving success in mathematics requires more than just memorizing formulas; it involves developing a systematic approach to problem-solving and mastering the essential concepts. By adopting the right strategies, students can significantly improve their understanding and performance. The key to excelling in any mathematical subject is consistent practice and a focus on mastering the fundamentals.
One important strategy is to break down complex problems into smaller, manageable steps. Rather than trying to solve everything at once, approach each part of the problem individually. This allows for a clearer understanding of how each step contributes to the overall solution.
Another effective method is to reinforce learning through practice exercises. The more problems you work through, the more familiar you become with the various techniques and approaches needed to tackle different types of questions. Practice not only builds familiarity but also helps you develop a problem-solving mindset, making it easier to approach new challenges with confidence.
Finally, don’t underestimate the value of reviewing and reflecting on past mistakes. Each error provides a valuable lesson that can help you refine your approach. Taking the time to understand what went wrong and why ensures that you won’t make the same mistake twice and helps strengthen your mathematical intuition.
Step-by-Step Algebra 1 Problem Solving
Solving mathematical problems requires a clear and organized approach. By breaking down each problem into manageable steps, you can ensure that no part of the solution is overlooked. This methodical process helps not only in finding the correct answer but also in understanding the reasoning behind each step. Whether dealing with equations, inequalities, or more complex expressions, a structured approach is key to success.
The first step is to carefully read the problem and identify what is being asked. Understanding the goal is essential before proceeding with any calculations. Make sure you know which variables are involved and what information is provided in the problem.
Next, simplify the equation or expression as much as possible. This might involve combining like terms, eliminating parentheses, or performing basic operations such as addition or subtraction. The goal is to reduce the problem to a more straightforward form that makes it easier to isolate the variable or solve the equation.
Once simplified, proceed by applying the appropriate operations to isolate the unknown. This often involves using inverse operations, such as adding the opposite of a number or dividing both sides of an equation by the same value. Each step should bring you closer to the solution.
Finally, check your solution by substituting your answer back into the original equation. If both sides are equal, then you know your solution is correct. If not, revisit the steps to identify where an error might have occurred.
How to Use Platoweb Efficiently
Maximizing the potential of online learning platforms can significantly enhance your understanding and performance in various subjects. With the right strategies, you can navigate the system more effectively, save time, and get the most out of the resources available. Whether you are tackling assignments, reviewing materials, or practicing skills, knowing how to use the platform efficiently will ensure that you stay on track and make the most of your study sessions.
Organize Your Tasks and Priorities
Start by creating a clear plan for what needs to be done. Break down your tasks into smaller, manageable chunks and prioritize them based on deadlines or difficulty. This will help you stay organized and avoid feeling overwhelmed by a long list of assignments. Use the platform’s tools to track your progress and set reminders for upcoming due dates.
Utilize Available Resources
Most online learning systems offer a range of materials designed to reinforce your understanding. Make sure to take full advantage of tutorials, practice exercises, and feedback provided by the platform. If you’re struggling with a specific topic, spend extra time reviewing related content and use practice quizzes to test your comprehension before moving on.
By staying organized and leveraging all available resources, you can approach your learning in a more focused and effective way, ultimately improving your mastery of the subject matter. Consistency and strategic use of the platform will lead to better results and greater success.
Why Algebra 1 Is Important for Math
Understanding foundational mathematical concepts is crucial for success in more advanced subjects. The first level of formal mathematics introduces key principles that serve as the building blocks for future learning. Without mastering these early topics, students may struggle with more complex concepts that build upon them. The importance of this initial stage cannot be overstated, as it prepares students for challenges in both academic and real-world problem-solving.
Building Critical Problem-Solving Skills
At its core, this level of mathematics helps develop essential problem-solving abilities. It encourages logical thinking, the ability to work through abstract concepts, and an understanding of how different mathematical operations are connected. These skills are not only valuable in mathematics but also in a variety of disciplines, such as science, engineering, economics, and technology.
Establishing a Strong Mathematical Foundation
By mastering the basic techniques and principles of this mathematical field, students lay the groundwork for more advanced study. Concepts such as working with variables, solving equations, and understanding functions are necessary to tackle higher-level topics like calculus, statistics, and geometry. This early understanding ensures that students are not left behind as they progress in their academic journey.
Mastering Variables and Equations
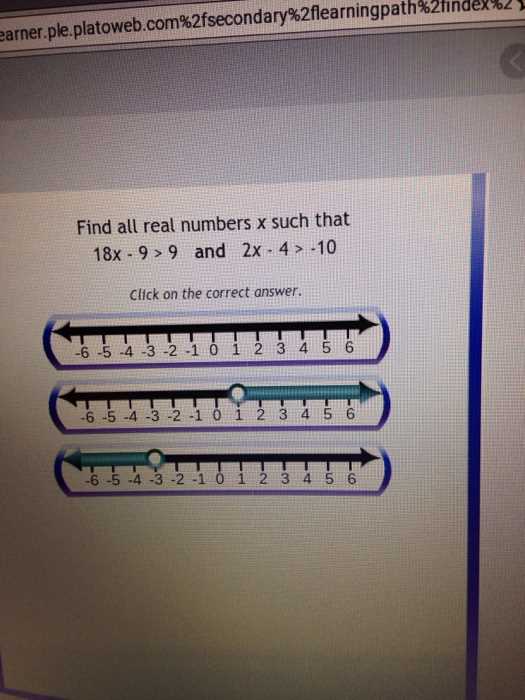
Understanding variables and equations is a fundamental skill in mathematics. These concepts serve as the foundation for solving a wide range of problems, from simple calculations to more complex scenarios. Mastering how to work with unknown values and relationships between numbers allows students to approach a variety of mathematical challenges with confidence and clarity.
At the heart of working with variables is the ability to translate real-world situations into mathematical expressions. Variables represent unknown quantities, and equations provide the structure to solve for these unknowns. By learning how to manipulate these equations–whether through addition, subtraction, multiplication, or division–students can find the value of the variable and gain insight into the relationships between different components.
Through consistent practice, students can improve their ability to simplify, solve, and understand equations. This skill not only strengthens problem-solving abilities but also prepares students for advanced topics that build upon these concepts, ensuring they are ready for more complex mathematical and scientific challenges in the future.
The Role of Graphs in Algebra 1
Graphs play a crucial role in understanding the relationships between different variables in mathematical problems. They provide a visual representation that allows students to see how changes in one quantity affect another, offering a clearer understanding of abstract concepts. By learning to interpret and create graphs, students gain valuable tools for solving problems and making connections between numerical and graphical data.
Graphs help to illustrate key concepts such as slope, intercepts, and the nature of functions. By plotting points on a coordinate plane, students can easily see patterns and trends that may be difficult to identify through equations alone. This visual approach helps to reinforce the understanding of how variables interact and provides a more intuitive grasp of the material.
Furthermore, graphs are essential for problem-solving, allowing students to approach challenges from a new angle. For example, solving equations graphically often involves finding the point where two lines intersect, which represents the solution to the equation. This method not only makes abstract problems more concrete but also gives students the ability to verify their answers and gain deeper insights into mathematical relationships.
Solving Word Problems in Algebra
Word problems are a common challenge in mathematics, requiring students to translate real-life situations into mathematical equations. While they may seem complex at first, breaking them down into smaller steps makes them more manageable. The key is to carefully read the problem, identify relevant information, and then set up the appropriate equations or expressions. By practicing this approach, students can improve their problem-solving skills and become more confident in tackling real-world mathematical challenges.
Steps to Solve Word Problems
- Read the problem carefully – Identify what is being asked and what information is provided.
- Define variables – Choose variables to represent unknown quantities.
- Set up equations – Translate the problem’s information into mathematical expressions or equations.
- Solve the equations – Use the appropriate mathematical methods to find the value of the unknown variable(s).
- Check your solution – Substitute your answer back into the problem to ensure it makes sense.
Common Strategies for Word Problems
- Look for keywords – Certain words like “total,” “difference,” “product,” and “sum” can help identify which operations to use.
- Draw diagrams – Visualizing the problem can make it easier to understand and set up the correct equation.
- Work backwards – If possible, start from the desired outcome and work backwards to determine the unknowns.
By following these steps and using strategies like identifying key terms and visualizing the problem, students can develop a more structured approach to solving word problems. With practice, they can turn complex scenarios into solvable equations and improve their overall mathematical reasoning.
Tips for Studying Algebra 1 Online
Studying mathematics online can be a highly effective way to learn, but it requires discipline and focus. With the right approach, online learning can provide flexibility and access to resources that traditional classroom settings might not offer. By organizing study time, using interactive tools, and applying effective study techniques, students can successfully navigate online math courses and strengthen their skills.
Effective Online Study Strategies
- Create a dedicated study space – Find a quiet, distraction-free environment where you can focus on your lessons and assignments.
- Set a study schedule – Consistency is key; create a regular study routine that includes time for reviewing materials and completing practice problems.
- Break lessons into manageable parts – Divide the material into smaller sections to avoid feeling overwhelmed and to make it easier to focus on one concept at a time.
- Use online resources – Take advantage of videos, forums, and interactive tools to reinforce your learning and clarify any doubts.
- Engage with online communities – Join study groups or participate in online discussions to deepen your understanding and collaborate with peers.
Maximizing Online Learning Tools
- Use virtual whiteboards and practice quizzes – Interactive tools can help visualize problems and assess your understanding in real-time.
- Watch tutorial videos – Many online courses provide step-by-step videos that can help break down complex concepts.
- Review feedback regularly – After completing exercises or tests, review your mistakes and feedback to improve your skills.
- Seek help when needed – Don’t hesitate to ask for assistance from tutors, instructors, or classmates if you’re struggling with certain topics.
With a proactive approach, the right resources, and effective time management, students can thrive in an online learning environment and build a solid foundation in math. Combining these strategies with regular practice will ensure continued progress and success throughout the course.
How to Check Your Algebra Answers
Verifying your work is an essential step in the problem-solving process. Ensuring that your solutions are correct helps build confidence and reinforces your understanding of key concepts. By using multiple strategies to double-check your solutions, you can catch errors early and strengthen your problem-solving skills.
Methods for Verifying Solutions
- Substitute your solution back into the original equation – After solving, plug your result back into the equation to check if both sides are equal.
- Use a different approach – Solve the problem using an alternative method to confirm that you reach the same result.
- Check for common errors – Look for mistakes like miscalculations, sign errors, or incorrect operations that may have occurred during the solving process.
- Review the problem setup – Make sure you understood the question correctly and didn’t make assumptions that led to incorrect conclusions.
Additional Tips for Accuracy
- Work step-by-step – Break down the solution into smaller, manageable steps to reduce the likelihood of making a mistake.
- Use estimation – Check if your solution is reasonable by estimating the result before solving the problem in full.
- Ask for feedback – If possible, have someone else review your work to catch any mistakes you may have missed.
By using these strategies, you can improve the accuracy of your solutions and develop stronger mathematical skills. Checking your work not only helps ensure correctness but also enhances your problem-solving abilities over time.
Using Platoweb for Algebra Practice
Online platforms provide valuable opportunities to reinforce and deepen your understanding of mathematical concepts. With interactive exercises and instant feedback, they offer a flexible way to practice problem-solving at your own pace. Utilizing such tools efficiently can significantly enhance your skills, allowing you to track progress and identify areas for improvement.
How Platoweb Helps Improve Math Skills
- Interactive exercises – Engage with hands-on activities that help strengthen your grasp on various mathematical principles.
- Instant feedback – Receive immediate corrections on your answers, which allows for quick identification of mistakes and faster learning.
- Customizable learning pace – Tailor your study sessions to fit your schedule and learning style, progressing through exercises at your own speed.
- Comprehensive content – Access a wide range of practice problems covering all essential topics, ensuring thorough preparation.
Maximizing the Benefits of Online Practice
- Set a study routine – Consistency is key. Make regular practice a habit to reinforce your knowledge.
- Focus on challenging areas – Spend extra time on problems or concepts that you find difficult to fully grasp.
- Track your progress – Monitor your scores and review past mistakes to ensure continuous improvement.
- Take advantage of available resources – Explore tutorials, hints, and additional explanations provided by the platform to clarify difficult concepts.
By making use of online platforms, students can effectively supplement their studies and improve their problem-solving capabilities, gaining confidence and proficiency in their mathematical journey.
Test Preparation Tips for Math Success
Effective test preparation requires a structured approach that focuses on understanding key concepts, practicing regularly, and addressing areas of difficulty. It is important to create a study plan that allows you to review all topics thoroughly while building confidence in solving problems. By organizing your study sessions and using the right strategies, you can enhance your performance and perform well on assessments.
Create a Study Schedule
Start by setting up a study schedule that breaks down the topics you need to review. Ensure that you allocate enough time to cover all material, giving extra focus to more challenging areas. Consistency is essential, so try to stick to your schedule each day.
Practice with Past Tests and Exercises
Reviewing previous tests and practice exercises is an excellent way to prepare for the test. Working through problems you have already encountered helps you identify patterns in the questions and improves your ability to recognize problem types quickly. It also helps build confidence in applying formulas and solving different types of equations.
Focus on Problem Areas
If certain topics or types of problems are more challenging, spend additional time on them. Targeting problem areas allows you to strengthen weak points and gain a better understanding of the material. Don’t hesitate to seek extra help or use online resources to clarify complex concepts.
Use Online Resources and Tools

Make use of online resources, such as video tutorials, practice quizzes, and interactive exercises, to supplement your studying. These tools often offer immediate feedback, helping you learn from your mistakes and improve your problem-solving techniques.
Stay Calm and Rested
In the days leading up to the test, ensure adequate rest to stay alert and focused. Overloading yourself with information at the last minute can lead to burnout. Prioritize sleep and stay calm–your brain needs rest to perform at its best during the exam.
By organizing your study sessions, practicing regularly, and focusing on key areas, you will feel more prepared and confident when it’s time to take the test. Keep a positive mindset, and trust in your preparation!
Getting Help with Math Homework
Struggling with homework can be overwhelming, especially when faced with complex problems or unfamiliar topics. However, there are various ways to seek assistance that can make the process easier and more manageable. By utilizing available resources and approaching the material methodically, you can overcome challenges and complete assignments effectively.
Ask Your Teacher for Clarification
Your teacher is one of the best resources when you’re unsure about a concept. Don’t hesitate to ask questions during class or after school to gain a better understanding of the material. Teachers can explain difficult topics, provide additional examples, and point you toward useful resources.
Use Online Educational Platforms
There are a variety of online platforms that offer step-by-step explanations, video tutorials, and practice problems. These websites often break down complicated problems into simpler steps, allowing you to understand the process better. Many sites also have interactive exercises where you can test your skills.
Form a Study Group
Working with classmates in a study group can be an effective way to tackle homework. Collaborating with others allows you to exchange ideas, explain concepts to one another, and find solutions together. Sometimes, discussing problems with peers can lead to new insights and better understanding.
Use Math Homework Help Apps
There are several math homework help apps that can instantly check your work, offer hints, or provide detailed solutions. These apps allow you to work through problems at your own pace while getting feedback along the way. Many apps also offer 24/7 support, so you can get help whenever you need it.
Visit Your School’s Tutoring Center
If your school offers tutoring services, take advantage of them. Tutors are trained to help students understand challenging concepts and can work with you one-on-one or in small groups. Often, tutors can provide explanations in a way that is easier for you to grasp.
Break Down the Problems
If you’re feeling stuck on a problem, try breaking it down into smaller, more manageable steps. Focus on one part of the equation at a time, and don’t rush through it. Sometimes, approaching a difficult problem slowly and systematically can make it much easier to solve.
Remember, seeking help is a sign of strength, not weakness. Whether you reach out to your teacher, use online tools, or work with others, there are plenty of ways to get the support you need. With the right resources and mindset, you’ll be able to tackle your homework with confidence.
Improving Your Math Skills Over Time
Building strong mathematical abilities requires consistent effort and a structured approach. While learning complex concepts may seem challenging at first, over time, you can enhance your problem-solving skills through focused practice, understanding key principles, and applying strategies that help reinforce your knowledge. Developing proficiency takes time, but with dedication, progress is inevitable.
Practice Regularly
The key to mastering any skill, especially in mathematics, is consistent practice. Set aside dedicated time each day or week to work on math problems, whether from textbooks, worksheets, or online resources. The more you practice, the more familiar you become with different types of problems and the quicker you can spot patterns and solutions.
Understand the Fundamentals
Before diving into more advanced topics, it’s essential to have a solid understanding of the basics. Concepts such as equations, inequalities, and number operations are foundational to more complex ideas. Spend time reviewing these fundamentals to ensure you’re not skipping steps or misapplying concepts in more difficult problems.
Break Problems into Steps
When tackling complex problems, break them down into smaller, more manageable parts. This step-by-step approach helps you stay organized and reduces the chances of making errors. Focus on solving one part at a time, and use what you already know to simplify more challenging sections. Over time, this technique will help you approach problems with more confidence.
Utilize Online Tools and Resources
There are numerous online tools available that can assist with your learning. Interactive websites, video tutorials, and problem solvers can provide detailed explanations and examples to clarify difficult topics. These resources are often available 24/7, allowing you to study at your own pace and revisit concepts whenever needed.
Seek Feedback and Ask Questions
Getting feedback is crucial for improving. Whether through a teacher, tutor, or study group, asking questions and discussing problems helps you identify areas for improvement. If something isn’t clear, ask for further explanations or alternative methods of solving the problem. Engaging with others enhances your understanding and helps reinforce learning.
Stay Patient and Consistent
Improvement doesn’t happen overnight, so it’s important to remain patient and persistent. If a concept feels difficult, take a break and come back to it later with fresh eyes. The more you engage with the material, the more familiar it will become, and gradually, you’ll notice an increase in your ability to solve problems quickly and accurately.
By following these strategies and committing to ongoing practice, you’ll steadily improve your math skills over time. With persistence and the right mindset, complex topics will become easier to navigate, and your confidence in solving problems will continue to grow.