
Preparing for a major math test can be a challenging yet rewarding experience. Understanding the concepts, mastering problem-solving techniques, and applying them efficiently is key to success. In this section, we will provide a comprehensive breakdown of the most common topics, along with practical steps to approach each question methodically. The goal is to help students tackle even the most complex problems with confidence.
By carefully reviewing the material and practicing key skills, students can gain a deeper understanding of the concepts covered. Each solution is presented in a clear, step-by-step format to show the reasoning behind every calculation. This approach ensures that learners are not only able to arrive at the correct solution, but also understand how to reach it.
Effective preparation involves more than just memorizing formulas; it requires a true grasp of the problem-solving process. Throughout this guide, we will emphasize the importance of methodical thinking and breaking down problems into manageable steps. Whether you are revising the core principles or tackling practice questions, this resource is designed to equip you for success.
June 2025 Algebra 2 Regents Overview
As the date of the upcoming examination approaches, it’s crucial to understand what will be tested and how to effectively prepare. This section provides an overview of what to expect, outlining key concepts and offering guidance on the most common problem types. By focusing on core topics, students can streamline their study efforts and improve their performance.
Key Areas of Focus
The test will cover a broad range of mathematical topics. Some of the most important areas to concentrate on include:
- Linear equations and functions
- Quadratic expressions and solving techniques
- Systems of equations and inequalities
- Exponential and logarithmic functions
- Rational expressions and equations
- Probability and statistics fundamentals
How to Prepare Effectively

Preparation is essential for achieving a high score. Consider the following strategies to maximize your readiness:
- Review past exams and practice problems to familiarize yourself with question formats.
- Understand the step-by-step processes for solving each type of problem.
- Use study guides and practice tests to identify areas of weakness.
- Focus on time management to ensure you can complete the entire exam.
By focusing on these key areas and adopting a structured study approach, students will be well-equipped to tackle the challenges of the exam.
What to Expect on the Exam
Understanding the format of the upcoming assessment is essential for effective preparation. This section outlines the structure and content areas you will encounter. Knowing what to expect allows you to focus your study efforts and perform at your best during the test.
The exam will consist of various types of questions designed to assess your understanding of key mathematical concepts. It will include both multiple-choice and open-ended problems, testing your ability to apply learned skills to new situations. The following table summarizes the main sections of the exam and their respective focus areas:
| Section | Content Focus | Question Type |
|---|---|---|
| Part 1 | Basic concepts and formula application | Multiple-choice |
| Part 2 | Problem-solving and calculations | Short answer |
| Part 3 | In-depth application of principles | Extended response |
By becoming familiar with these components and practicing problem-solving techniques, you can ensure that you are fully prepared for the range of challenges the exam will present.
Key Topics for Algebra 2 Regents
To perform well on the upcoming assessment, it is essential to understand the key areas that will be tested. Focusing on the fundamental topics ensures that you are prepared to handle the variety of problems that may arise. Below, we outline the main subjects that are likely to be featured in the exam, highlighting the skills and concepts you need to master.
Functions and Graphs: A significant portion of the test will cover functions and their graphical representations. You should be familiar with the properties of linear, quadratic, and other common functions, including how to graph them and interpret their characteristics.
Systems of Equations: Solving systems of equations, both algebraically and graphically, will be another key component. Understanding how to manipulate and solve these systems is crucial for answering related questions correctly.
Exponential and Logarithmic Equations: Expect to encounter problems involving exponential growth, decay, and the use of logarithmic functions. Be comfortable with the properties and applications of both types of equations.
Polynomials and Rational Expressions: The test will also include questions on polynomial functions and their manipulation, as well as rational expressions. Mastering operations such as factoring and simplifying these expressions is vital.
Probability and Statistics: Basic concepts in probability, such as counting principles and probability distributions, will appear. Additionally, you should be familiar with statistical measures and their application to real-world data.
By focusing on these core topics and practicing related problems, you can build a solid foundation for success on the exam. The skills you gain will not only help you perform well on the test but also provide a deeper understanding of mathematical principles.
How to Approach the Questions
Effective problem-solving begins with understanding the structure and requirements of each question. When facing an assessment, it’s important to approach each problem systematically, breaking it down into manageable steps. This strategy not only helps you stay focused but also ensures that you don’t miss key details.
The first step is to carefully read each question to fully comprehend what is being asked. Highlight key information, such as values, terms, and instructions. If the problem involves multiple parts, make sure to address each one individually, staying organized throughout the process.
Next, determine which mathematical concepts are applicable. Whether it’s solving for a variable, graphing a function, or simplifying an expression, identify the method that best fits the problem. Write out all necessary formulas and note any conditions that might affect the solution.
As you work through the problem, ensure that each step is clearly explained. This not only helps in double-checking your process but also demonstrates a complete understanding of the concept. If the question involves calculations, do them methodically, and review your work as you go.
Finally, after completing the problem, revisit the question to ensure your solution aligns with what was asked. If any part seems unclear, revisit your steps and make adjustments as necessary. By staying organized and thoughtful in your approach, you’ll be able to tackle the questions confidently and accurately.
Step by Step Solutions Explained
Mastering the process of solving mathematical problems requires understanding each phase of the solution, from identifying the problem to reaching the final result. This section provides a breakdown of how to approach problems methodically, offering clear, detailed steps that will guide you through various scenarios. By following a structured approach, you can increase both your accuracy and confidence in solving complex questions.
Identifying Key Information
The first step in any problem is to identify the essential information. Carefully examine the question to determine what is given and what needs to be found. Whether the problem involves an equation, a graph, or a word scenario, organizing the data is crucial. Highlight any numbers, variables, and conditions that directly influence the solution, as these will be key in shaping your approach.
Solving Using the Appropriate Method
Once you have all the necessary details, begin solving by applying the correct method or formula. If the question involves an equation, isolate the variable by performing the appropriate operations, such as adding, subtracting, multiplying, or dividing. For more complex problems, break them down into smaller parts and solve each one individually before combining the results. Always double-check your calculations to ensure accuracy as you proceed.
By following these step-by-step instructions, you will not only arrive at the correct solution but also gain a deeper understanding of the concepts involved. With consistent practice, this approach will become second nature, allowing you to tackle any problem with clarity and precision.
Understanding Common Mistakes
In any complex problem-solving process, it is easy to make small errors that can lead to incorrect results. Recognizing common mistakes is an essential part of learning, as it helps to avoid repeating them and improves overall accuracy. In this section, we will highlight some frequent errors and provide guidance on how to prevent them in your solutions.
Misinterpreting the Question
One of the most common mistakes occurs when the problem itself is not fully understood. Often, important details are overlooked, or the instructions are misread, leading to the wrong approach. To avoid this, carefully read through the problem multiple times, highlighting key information such as what needs to be found, what is given, and any conditions or constraints. Ensuring you grasp the full context before proceeding will set you up for a more successful solution process.
Incorrect Application of Formulas
Another frequent mistake is the misuse or misapplication of formulas. This often happens when a formula is memorized without fully understanding its components. Always review the formula carefully to ensure each term is properly accounted for. Additionally, pay attention to signs and operations, as small mistakes in these areas can lead to significant errors in the final solution.
By being aware of these common pitfalls, you can avoid them and refine your problem-solving techniques. Double-checking your work at each stage of the process is a good habit that will help reduce errors and increase confidence in your results.
Tips for Effective Time Management
Managing your time efficiently is key to performing well on any examination. By planning ahead and structuring your time wisely, you can ensure that you complete every section of the test with accuracy and confidence. This section provides helpful tips for organizing your study sessions and maximizing your focus during the assessment itself.
Prioritize and Plan Ahead

Effective time management begins with setting clear priorities. Start by identifying which topics require more attention and which you are already confident in. Create a study schedule that focuses on the most challenging areas, but also leaves time for revision of material you’re comfortable with. Here are a few strategies to help you stay on track:
- Break down large topics into smaller, manageable parts.
- Set specific goals for each study session, such as completing a set number of problems.
- Use a calendar or planner to mark important deadlines and exam dates.
Maximize Focus During the Exam
When you’re taking the test, time management becomes equally important. Avoid spending too much time on any single question, as this can lead to unnecessary stress. Here are some strategies for managing time effectively during the test:
- Quickly scan through all questions at the start and determine how long to spend on each.
- If you get stuck on a question, move on and return to it later.
- Leave a few minutes at the end to review your answers, ensuring you didn’t miss any critical details.
By implementing these time management strategies, you can improve your efficiency and reduce anxiety, ultimately increasing your chances of success. With careful planning and focused execution, you will be better equipped to handle the exam’s demands.
Reviewing Key Algebra 2 Concepts
Refreshing your understanding of important mathematical principles is essential for performing well in any assessment. In this section, we will revisit key topics that are commonly tested, ensuring that you have a solid grasp of the material. By reinforcing your knowledge of these fundamental concepts, you will be better equipped to approach any question confidently and accurately.
Important Functions and Their Properties
One of the core areas to focus on is understanding various types of functions and their characteristics. These functions form the foundation for many problems and can be used in a wide range of scenarios. Here are some key concepts to review:
- Linear Functions: Be familiar with slope-intercept form and the ability to identify slopes and y-intercepts.
- Quadratic Functions: Review how to solve quadratic equations by factoring, completing the square, or using the quadratic formula.
- Exponential Functions: Understand how to solve problems involving exponential growth or decay, and how to apply logarithms.
Solving Equations and Inequalities
Being able to solve various types of equations and inequalities is critical for success. Practice solving for variables in different contexts, and make sure you’re comfortable working with:
- Linear and quadratic equations.
- Systems of equations, including both substitution and elimination methods.
- Inequalities and understanding how to graph solutions on a number line.
By reviewing these fundamental concepts and practicing problem-solving techniques, you will be better prepared to tackle a wide range of questions. The more familiar you are with the core topics, the easier it will be to recognize patterns and apply the right methods during the assessment.
Important Formulas for the Exam
Having a strong grasp of essential formulas is crucial for tackling problems efficiently and accurately. These formulas serve as tools to simplify complex calculations and help solve problems quickly. In this section, we will cover some of the most important formulas that are frequently tested. Understanding these will not only aid in problem-solving but also improve your overall performance.
Here are some key formulas to remember:
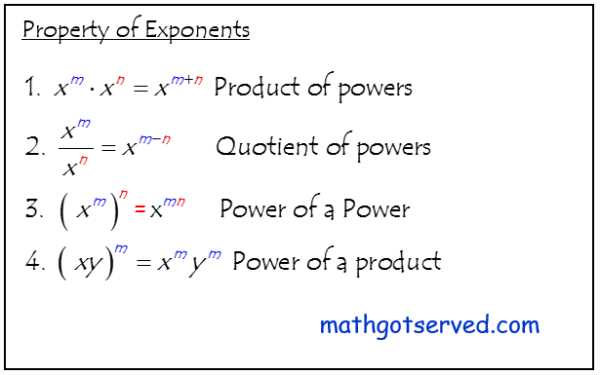
- Quadratic Formula: Used to solve quadratic equations. The formula is x = (-b ± √(b² – 4ac)) / 2a.
- Slope-Intercept Form: The equation of a line is y = mx + b, where m is the slope and b is the y-intercept.
- Distance Formula: To calculate the distance between two points (x₁, y₁) and (x₂, y₂), use d = √[(x₂ – x₁)² + (y₂ – y₁)²].
- Exponential Growth/Decay Formula: For growth or decay problems, use A = P(1 ± r)^t, where A is the final amount, P is the initial amount, r is the rate, and t is time.
- Pythagorean Theorem: In right triangles, a² + b² = c², where a and b are the legs, and c is the hypotenuse.
These formulas are essential for efficiently solving problems in various topics. By reviewing and memorizing these, you’ll be able to approach the exam confidently and tackle each question methodically.
Common Algebra 2 Regents Questions
During assessments, certain types of problems tend to appear frequently, requiring specific problem-solving strategies. In this section, we will explore some of the most common types of questions you may encounter. Understanding these question formats and practicing their solutions will help you prepare effectively and approach the exam with confidence.
Typical Question Types
The following question categories are frequently tested, so it’s essential to review and practice them:
- Quadratic Equations: These questions typically ask you to solve quadratic equations using factoring, the quadratic formula, or completing the square.
- Systems of Equations: You may need to solve for variables in a system of linear equations, either using substitution or elimination methods.
- Word Problems: Often, real-life scenarios are used to test your ability to set up and solve equations based on given information.
- Functions and Graphs: Questions on identifying, interpreting, and graphing different types of functions are common.
- Exponential Growth/Decay: These problems often involve applications of exponential functions, requiring you to calculate growth or decay over time.
- Inequalities: You may encounter problems asking you to solve inequalities and represent the solutions graphically.
Common Problem-Solving Techniques
To tackle these types of questions effectively, it’s important to employ the right strategies. Here are some tips:
- For quadratic equations, always check if factoring is possible before applying the quadratic formula.
- When solving systems of equations, identify the method that works best for the specific problem – substitution is often easier when one equation is solved for a variable.
- Word problems require you to translate real-world scenarios into mathematical expressions, so focus on identifying key variables and relationships.
- For inequalities, remember to reverse the inequality sign when multiplying or dividing by a negative number.
Familiarizing yourself with these common question types and practicing their solutions will ensure you’re prepared to tackle similar challenges on your exam.
How to Check Your Work
Ensuring the accuracy of your solutions is an essential part of the problem-solving process. Even if you think you’ve completed a problem correctly, taking the time to review your steps and results can help catch errors that may have been overlooked. In this section, we will explore effective strategies to check your work and verify that your answers are correct.
Methods for Verifying Solutions
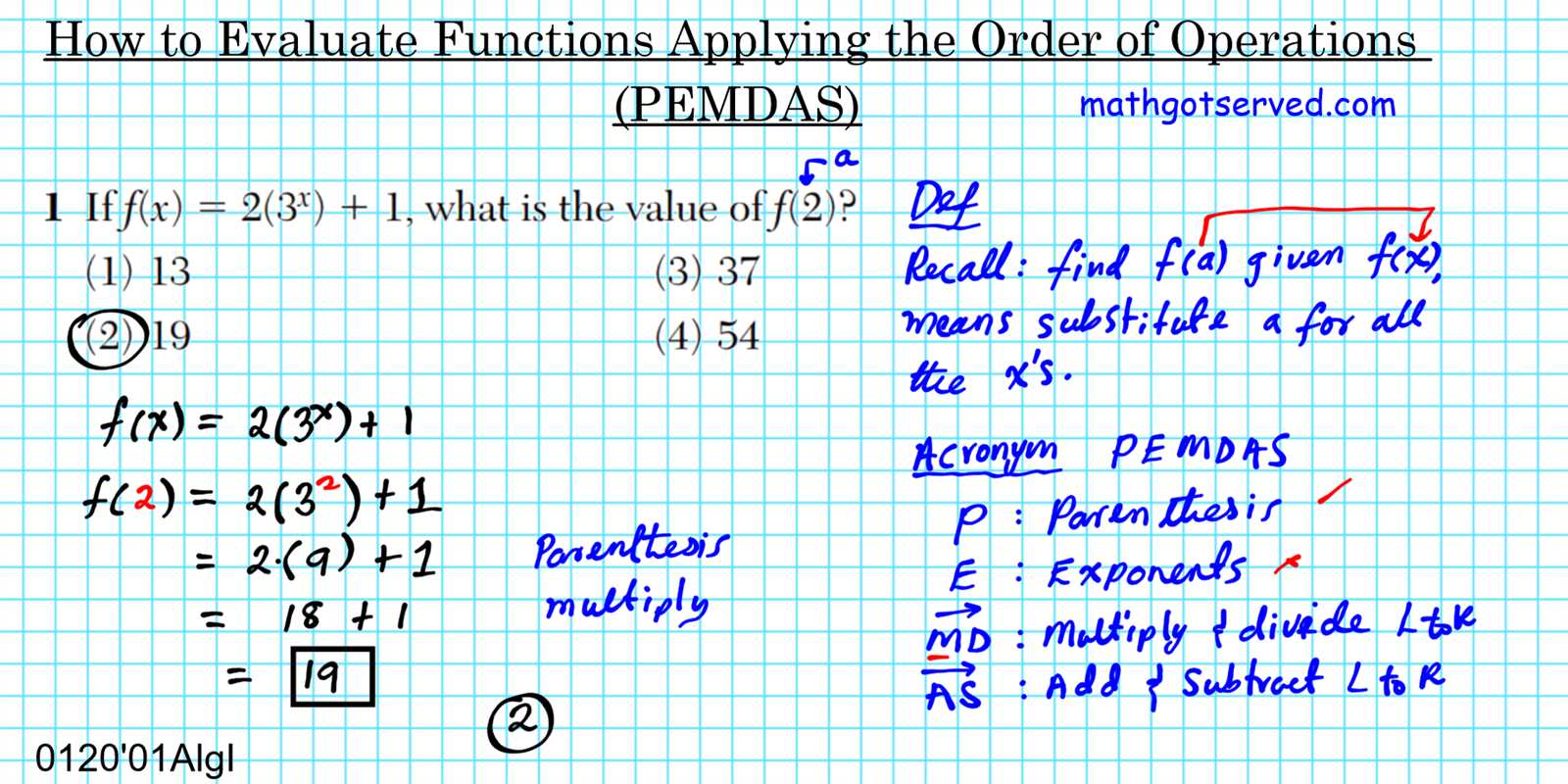
There are several strategies you can use to confirm the accuracy of your solutions. These methods can be applied to various types of problems, ensuring that you are thorough in your review:
- Substitute Back: For many problems, especially equations, you can substitute your solution back into the original equation to check if it satisfies the given conditions.
- Estimate: Before solving, estimate the possible range for your answer. If your result is far outside of this range, it’s a sign to recheck your work.
- Use a Different Method: If possible, try solving the problem using a different approach and compare results. For example, if you used factoring, check with the quadratic formula.
- Cross-Check Units: In problems involving measurements or conversions, ensure that your units are consistent throughout the solution process.
Steps to Follow for a Final Check
After completing your solution, it’s important to take a few extra steps before finalizing your answer:
| Step | Action |
|---|---|
| Step 1 | Review the problem statement to ensure you understand the question correctly. |
| Step 2 | Go through each step of your solution carefully and make sure all calculations are accurate. |
| Step 3 | Verify that the units, if applicable, match the desired outcome. |
| Step 4 | If possible, use a calculator or alternative methods to check the final result. |
| Step 5 | Double-check any work done by hand, particularly when dealing with complex calculations. |
By taking the time to review your work using these strategies, you can significantly reduce the likelihood of making mistakes and increase your confidence in the accuracy of your answers.
Test Taking Strategies for Success
Performing well on an exam requires more than just knowing the material. It involves using effective strategies to manage time, stay focused, and approach each question with confidence. This section provides key tips for navigating the test, ensuring that you maximize your chances of success and avoid common pitfalls.
Preparation Before the Test
Preparing effectively for an exam begins long before the day of the test. Successful preparation lays the foundation for tackling questions with ease and confidence:
- Practice Regularly: Consistent practice with sample questions and past exams helps familiarize you with the test format and builds problem-solving speed.
- Review Key Concepts: Focus on understanding fundamental ideas and formulas that are likely to appear. Make sure you can apply them in different scenarios.
- Organize Your Notes: Neatly organized notes make it easier to study. Break down complex topics into smaller, manageable sections for quick reference.
Effective Strategies During the Test
When it comes time to take the test, how you approach it can have a significant impact on your performance:
- Read Carefully: Always read each question thoroughly before attempting to answer. Pay attention to keywords and any instructions that may affect how you approach the problem.
- Manage Your Time: Allocate a specific amount of time to each section or question, and make sure to pace yourself. If you get stuck, move on and return to difficult questions later.
- Answer Easy Questions First: Tackle questions you know well first to gain confidence and save time for more challenging ones.
- Eliminate Incorrect Choices: When faced with multiple-choice questions, use the process of elimination to narrow down the options and increase your chances of choosing the correct answer.
Staying Calm and Focused
Maintaining composure is just as important as understanding the material. Stress and anxiety can hinder performance, so it’s crucial to remain calm:
- Take Deep Breaths: If you start to feel overwhelmed, take a few deep breaths to refocus your mind and regain control.
- Stay Positive: Keep a positive mindset throughout the test. Remind yourself that you have prepared, and you are capable of succeeding.
- Stay Physically Comfortable: Ensure that you’re sitting comfortably and have everything you need (like a water bottle or extra pens) to avoid distractions.
By incorporating these strategies, you can approach your test with confidence, use your time effectively, and improve your chances of performing at your best.
How to Avoid Calculation Errors
Calculation errors are common during exams, but they can be minimized with a few simple strategies. By staying organized, methodical, and aware of potential pitfalls, you can reduce the likelihood of making mistakes while solving problems. This section highlights tips to help you approach calculations with greater accuracy and confidence.
Double-Check Your Work
One of the easiest ways to catch errors is to review your solutions carefully. By revisiting each step, you can identify mistakes before finalizing your answer:
- Recalculate: After completing a problem, quickly recalculate the key steps or the final result to confirm its accuracy.
- Check Units: Make sure that all units are consistent throughout the problem. Converting between different units or dimensions can lead to errors.
- Verify Key Assumptions: Check that you haven’t overlooked important assumptions or conditions mentioned in the problem.
Break Down Complex Problems
For complicated problems, break the task into smaller, more manageable parts. This approach minimizes the risk of making mistakes due to complexity or haste:
- Work in Steps: Solve each part of the problem step by step, and write down each intermediate result clearly. This makes it easier to spot mistakes later.
- Use Clear Notation: Write your calculations neatly and clearly, keeping each step organized. This reduces the chance of misreading your own work.
- Focus on One Operation at a Time: Don’t rush through calculations by skipping steps or combining multiple operations at once. This increases the likelihood of making errors.
Common Pitfalls to Avoid
There are several common mistakes that students often make during exams. Being aware of these can help you stay vigilant:
- Sign Errors: Be cautious with negative signs, especially when adding or subtracting terms. Double-check whether you’ve used the correct sign at each step.
- Order of Operations: Always follow the correct order of operations (PEMDAS: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction) to avoid mixing up the sequence of calculations.
- Overlooking Zeroes: Pay attention to zeroes when multiplying or dividing. A misplaced zero can drastically change your result.
By taking your time and following these techniques, you can minimize calculation errors and ensure that your solutions are as accurate as possible.
Practicing with Previous Exams
Reviewing past tests is one of the most effective strategies for preparing for any type of assessment. By practicing on previous exams, students gain valuable insights into the format, question types, and the level of difficulty they might encounter. This process not only improves familiarity but also helps develop essential problem-solving skills, ensuring readiness when it comes time for the actual test.
Benefits of Using Past Tests
Working through old exams can provide several advantages:
- Time Management Skills: Past exams allow you to practice completing questions within the time constraints, helping you manage your time more effectively during the actual test.
- Identify Knowledge Gaps: Reviewing old exams can reveal areas where you need to improve your understanding. By identifying these gaps, you can focus your efforts on mastering these concepts before the test.
- Familiarity with Test Structure: Getting used to the format of questions, whether they are multiple choice, short answer, or long-form problems, will help you feel more confident and less stressed during the actual exam.
How to Make the Most of Practice Exams
Simply completing past exams is not enough. To gain the maximum benefit, consider the following tips:
- Simulate Exam Conditions: Try to recreate exam conditions by timing yourself and eliminating distractions. This will help you become accustomed to the pressure and pace of the real test.
- Review Mistakes: After completing each practice test, carefully review your mistakes. Understanding why you made an error will prevent you from repeating it in the future.
- Seek Help When Necessary: If you encounter a question or concept that you don’t fully understand, don’t hesitate to seek clarification from a teacher, tutor, or reliable educational resources.
Where to Find Past Exams
Finding previous exams can be straightforward. Here are a few places to look:
| Source | Availability |
|---|---|
| School Website | Often posts archived exams and practice materials. |
| Textbooks | Many textbooks include sample exams or practice questions at the end of chapters. |
| Online Resources | Websites dedicated to test preparation may have past exams and answer keys available. |
| Study Groups | Collaborating with peers may provide access to shared resources, including old exams. |
By dedicating time to practicing past exams, students can enhance their problem-solving abilities, improve their confidence, and significantly increase their chances of success on the upcoming assessment.
Using Study Guides Effectively
Study guides are a valuable tool for organizing and reviewing the key concepts and material you need to master before any examination. However, simply having a study guide is not enough; it is important to use it strategically. The most effective use of study guides involves a systematic approach that emphasizes active learning, time management, and targeted review.
Key Strategies for Using Study Guides
To get the most out of a study guide, consider the following tips:
- Prioritize Key Topics: Focus on the most important and frequently tested concepts. Look for sections that have been highlighted by your teacher or that are commonly featured in past assessments.
- Break it Down: Divide the material into smaller sections and tackle one at a time. This makes the content less overwhelming and helps reinforce retention.
- Use Active Recall: Test yourself frequently rather than passively reading through the material. Use flashcards, quizzes, or practice problems to actively engage with the concepts.
- Make Notes: While reviewing, jot down notes or summaries of the most important points. These quick notes can serve as a quick reference and help reinforce your understanding.
Maximizing Your Study Time
Efficient use of a study guide requires good time management and focus. Here are some tips to help you optimize your study sessions:
- Create a Schedule: Set aside dedicated time each day for focused study. Break down the material into manageable chunks and allocate specific times for each section.
- Avoid Cramming: Start using the study guide well in advance of the exam date. Cramming at the last minute is ineffective and can lead to unnecessary stress.
- Review Regularly: Instead of studying everything at once, review your guide regularly over the course of several days or weeks. This will help improve retention and prevent burnout.
Leveraging Additional Resources
While a study guide can be comprehensive, it is always beneficial to supplement it with other materials. Consider using the following resources alongside your study guide:
- Textbooks: Review chapters that cover the same topics as the study guide for a deeper understanding.
- Online Resources: Look for videos, tutorials, and practice tests that explain concepts in different ways or provide additional exercises.
- Study Groups: Collaborate with peers to discuss difficult concepts and quiz each other. Explaining material to others can reinforce your own understanding.
By using your study guide strategically, you can ensure that you are well-prepared, confident, and ready for any upcoming assessment.
How to Stay Calm During the Test
Staying calm during an examination is essential for performing at your best. Anxiety and stress can cloud your thinking and hinder your ability to solve problems effectively. It’s important to approach the test with a clear and focused mind. By using simple techniques and strategies, you can reduce stress and stay calm, allowing your preparation to shine through.
Techniques for Managing Stress
Here are some proven methods to help maintain a calm and focused mindset during the test:
- Deep Breathing: Taking slow, deep breaths helps reduce anxiety and clears your mind. Try breathing in for a count of four, holding for four, and exhaling for four. Repeat this a few times to center yourself.
- Positive Affirmations: Remind yourself of your strengths and preparation. Tell yourself that you can handle the task and that you are capable of succeeding.
- Focus on the Present: Concentrate only on the current question or section. Avoid worrying about questions you may have skipped or that seem difficult. Take one step at a time.
Managing Time and Anxiety
Time pressure can also lead to stress, but with effective time management, you can feel more in control:
- Start with the Easiest Questions: If you’re feeling anxious, begin with the questions that seem the most straightforward. This can build confidence and momentum.
- Don’t Rush: Maintain a steady pace throughout the test. Rushing through questions can lead to careless mistakes and increase anxiety.
- Take Breaks: If the test allows for it, take short breaks to clear your mind. Stand up, stretch, or simply close your eyes for a moment. This can help reset your focus.
Preparing Mentally Before the Test
Before the exam day arrives, mental preparation is just as important as academic preparation:
- Get Plenty of Rest: Ensure you get a good night’s sleep before the exam. Being well-rested will help you stay focused and calm during the test.
- Eat Well: A balanced meal can keep your energy levels up and prevent distractions. Avoid heavy, greasy foods that could make you feel sluggish.
- Arrive Early: Give yourself plenty of time to settle in and adjust to the environment. Rushing to the exam room can increase feelings of stress.
By incorporating these strategies into your exam routine, you can stay calm, focused, and ready to tackle any challenge that comes your way.
Preparing in the Final Week
The final week before an exam is crucial for reinforcing your knowledge and ensuring that you are ready for the challenges ahead. At this point, it’s not about learning new material, but rather about reviewing what you already know, honing your skills, and building confidence. Efficient time management and targeted practice can make a significant difference in your performance.
Review Key Topics and Concepts

During the last week, focus on revisiting the most important topics that are frequently tested. This will help you feel more prepared and reduce any uncertainty.
- Review Practice Problems: Go through problems from past exams or practice tests to identify areas where you need improvement. Focus on types of questions you struggled with and practice until you feel confident.
- Condense Notes: Create a one-page summary or a set of flashcards that highlight the key concepts, formulas, and strategies you’ve learned. This will make it easier to quickly refresh your memory.
- Identify Weak Spots: If you notice certain areas are still challenging, spend extra time on them. Don’t be afraid to ask for help from a teacher or tutor if needed.
Stay Balanced and Focused

In the final days leading up to the exam, balance your preparation with relaxation and mental clarity. Overloading yourself with information can lead to burnout and stress.
- Set Realistic Goals: Break your study sessions into manageable chunks. For example, aim to review specific topics each day rather than cramming everything at once.
- Get Enough Rest: Don’t sacrifice sleep for study. A well-rested mind functions more efficiently and helps retain information better.
- Practice Mindfulness: Incorporate techniques such as deep breathing or meditation to reduce anxiety and stay calm under pressure.
By focusing on effective study habits and maintaining balance, you can enter the exam confidently, knowing that you’ve done everything you can to prepare.
Where to Find Additional Resources
In addition to your textbooks and class notes, there are various resources available to further your preparation. Using a combination of online materials, study groups, and practice tools can help reinforce your understanding and improve your skills. Here are some excellent options to consider for extra support.
- Online Educational Platforms: Websites like Khan Academy, Coursera, and edX offer free courses and tutorials on a wide range of subjects. These platforms often provide step-by-step lessons that can help clarify difficult concepts.
- Practice Websites: Sites such as IXL, Mathway, and Wolfram Alpha allow you to practice problems interactively and get instant feedback. These tools can help you identify areas where you need more focus.
- Educational YouTube Channels: YouTube is a treasure trove of educational videos. Channels like PatrickJMT, MathTheBeautiful, and Professor Leonard offer clear explanations of mathematical concepts that you can watch at your own pace.
- Study Groups: Join study groups or online forums where you can collaborate with peers. Platforms like Reddit, Discord, or even local study sessions offer opportunities to discuss problems, share tips, and gain different perspectives.
- Tutoring Services: If you’re looking for more personalized help, consider reaching out to tutoring services, either in-person or online. Websites like Wyzant and Chegg provide access to professional tutors who can guide you through specific challenges.
By utilizing a variety of these resources, you can enhance your preparation and increase your chances of success. Make sure to explore multiple options to find what works best for your learning style.