
In the initial section of this mathematical course, you will explore fundamental concepts that form the backbone of more complex problem-solving strategies. This section lays the groundwork for understanding key principles that are essential for mastering various types of equations and expressions.
Throughout this unit, students will encounter a range of exercises designed to strengthen their analytical skills and improve their ability to approach different mathematical challenges. By focusing on practical methods and step-by-step solutions, learners can develop a deeper understanding of mathematical structures.
Whether you are working with linear equations, simplifying expressions, or tackling real-world problems, this section offers the necessary tools to build a strong foundation. The content is designed to guide you through various problem-solving techniques, ensuring a well-rounded understanding of the material at hand. With the right approach, mastering these early lessons will pave the way for success in more advanced topics.
Mathematical Problem-Solving Solutions
This section provides valuable solutions to a range of key exercises, designed to enhance your understanding of foundational mathematical concepts. By working through the provided explanations and calculations, you’ll gain insight into various problem-solving methods that will help you succeed in more advanced topics.
Step-by-Step Guidance
The exercises in this section are carefully structured to allow you to follow each solution methodically. Here are the main approaches for tackling problems:
- Identify the given information and the goal of the problem.
- Apply appropriate mathematical principles to break down the problem into manageable parts.
- Double-check each step to ensure no errors are made in the calculations.
- Review the solution by testing it against the initial conditions.
Helpful Techniques for Success
As you progress through the problems, keep in mind these important techniques to maximize your learning:
- Practice regularly: Consistent practice ensures that key concepts become second nature.
- Understand the logic: Rather than memorizing steps, focus on understanding why each approach works.
- Review your mistakes: Mistakes provide an excellent learning opportunity. Analyzing errors helps you avoid them in the future.
- Seek patterns: Identifying patterns in solutions makes solving similar problems faster and easier.
With this methodical approach, solving the problems in this section will help you develop a solid foundation for more complex mathematical topics.
Understanding Key Concepts in Chapter 1
The first section of this material introduces fundamental ideas that are essential for mastering more complex mathematical problems. Understanding these concepts is critical, as they form the foundation for later topics and provide the tools needed for effective problem-solving.
Key ideas in this section revolve around basic operations and their applications, offering you the chance to build a solid grasp of essential techniques. By focusing on these concepts, you’ll develop the skills necessary to approach more challenging problems with confidence.
Essential Principles to Focus On
Here are some of the core ideas you should focus on while studying this material:
- Equations and Expressions: Learn how to manipulate and simplify equations to solve problems efficiently.
- Properties of Numbers: Understanding the properties that govern numbers is essential for simplifying and solving various problems.
- Linear Relationships: Recognizing and solving linear relationships is a key step in mastering more advanced techniques.
- Functions and Graphs: Gain a strong understanding of how different functions are represented graphically and how to analyze them.
Approaching Problems with Clarity
As you work through problems, remember the following strategies to ensure a solid understanding of the key concepts:
- Break down complex problems: Start by identifying the variables and the goal of the problem.
- Apply learned principles: Use the properties and methods you’ve learned to tackle each step logically.
- Check your work: Always review your steps to ensure accuracy before finalizing the solution.
- Practice regularly: Repeated practice with different types of problems reinforces key concepts and sharpens your skills.
Mastering these basic principles will lay the groundwork for tackling more advanced topics as you progress in your studies.
Step-by-Step Solutions for Exercises
In this section, we provide detailed explanations for solving various exercises, helping you understand the logic behind each solution. By following the step-by-step breakdown, you will learn how to approach similar problems effectively and efficiently.
Approach Each Problem Methodically
Every problem can be broken down into smaller, manageable steps. Here’s a general approach to solving exercises in this material:
- Identify the goal: Clearly understand what the problem is asking for before starting.
- Organize the information: List all known values and variables before beginning calculations.
- Apply appropriate operations: Use the correct mathematical principles to manipulate the equation or expression.
- Check for consistency: After solving, review your steps to ensure the solution is valid and correct.
Key Tips for Effective Problem Solving
While working through each exercise, keep these helpful strategies in mind:
- Work systematically: Follow each step carefully without skipping any, ensuring accuracy.
- Look for patterns: Identifying patterns in similar problems can make solving them faster.
- Practice regularly: The more you practice, the more familiar and comfortable you will become with solving problems.
- Review common mistakes: Understanding frequent errors can help you avoid them in future exercises.
By following these steps and strategies, you can develop strong problem-solving skills and build confidence in tackling more complex exercises.
How to Solve Key Mathematical Problems
Solving mathematical problems requires a systematic approach that allows you to break down complex tasks into simpler, manageable parts. By understanding the underlying principles and applying the right techniques, you can efficiently find solutions to various types of equations and expressions.
Step-by-Step Approach to Problem Solving
To tackle any mathematical problem effectively, follow these key steps:
- Analyze the problem: Begin by identifying the variables, known values, and what the problem is asking you to find.
- Choose the correct method: Based on the type of equation, decide which mathematical technique or operation is most appropriate.
- Perform calculations: Work through each step of the solution carefully, using the correct operations and ensuring accuracy.
- Review the result: After reaching a solution, check it against the original problem to ensure consistency.
Effective Techniques for Solving Problems
By incorporating these strategies into your approach, you can improve both your understanding and efficiency:
- Work step-by-step: Avoid jumping ahead; each step is crucial for accuracy.
- Check for patterns: In many problems, recognizing recurring patterns can simplify the process.
- Practice different types: Diversify your practice with problems of various complexities to build versatility.
- Validate your solution: Always double-check the final answer and ensure it fits the problem’s requirements.
With consistent practice and a structured approach, you will become more confident and proficient in solving mathematical problems, paving the way for success in future topics.
Common Mistakes to Avoid in Algebra 2
While solving mathematical problems, it’s easy to make certain errors that can derail your progress. Recognizing and avoiding these common mistakes is key to mastering the material and improving your problem-solving skills. By understanding where mistakes tend to occur, you can approach exercises with greater confidence and accuracy.
Below are some of the most frequent pitfalls students encounter, along with tips on how to avoid them:
| Common Mistake | How to Avoid It |
|---|---|
| Skipping steps in calculations | Always write out each step to ensure accuracy and clarity in your work. |
| Misinterpreting the problem | Read the problem carefully, paying attention to what is being asked before starting your solution. |
| Incorrectly applying formulas | Double-check the formula and make sure it is relevant to the problem you’re solving. |
| Forgetting to check your work | Always review your final answer to verify that it meets the requirements of the question. |
| Rushing through the problem | Take your time to analyze each step carefully, ensuring no errors are overlooked. |
By staying aware of these common issues and adopting good habits, you can avoid these mistakes and improve both your understanding and performance in future exercises.
Detailed Explanations for Chapter 1 Problems
This section provides in-depth explanations for the problems covered in the first section of the course. By breaking down each problem step by step, you will gain a clearer understanding of the methods used to find solutions and how to apply them to similar challenges.
Each problem is carefully analyzed, and the approach used to solve it is thoroughly explained to ensure that all key concepts are understood. Whether you are working with equations, expressions, or other mathematical scenarios, these detailed walkthroughs will help you build a strong foundation for future topics.
| Problem Type | Solution Approach |
|---|---|
| Simplifying Expressions | Break the expression down into smaller parts, apply the order of operations, and simplify step by step. |
| Solving Equations | Isolate the variable by performing inverse operations on both sides of the equation. |
| Factoring Quadratic Expressions | Look for common factors or use methods like the quadratic formula to find the roots of the expression. |
| Working with Functions | Evaluate the function by substituting the given values into the function’s expression. |
| Graphing Linear Equations | Identify the slope and y-intercept, then plot points and draw the line. |
By following these detailed steps, you’ll gain a deeper understanding of the mathematical concepts covered in this section. Each explanation is designed to reinforce your learning and ensure that you’re equipped to tackle more complex problems moving forward.
Mastering Algebraic Equations in Chapter 1
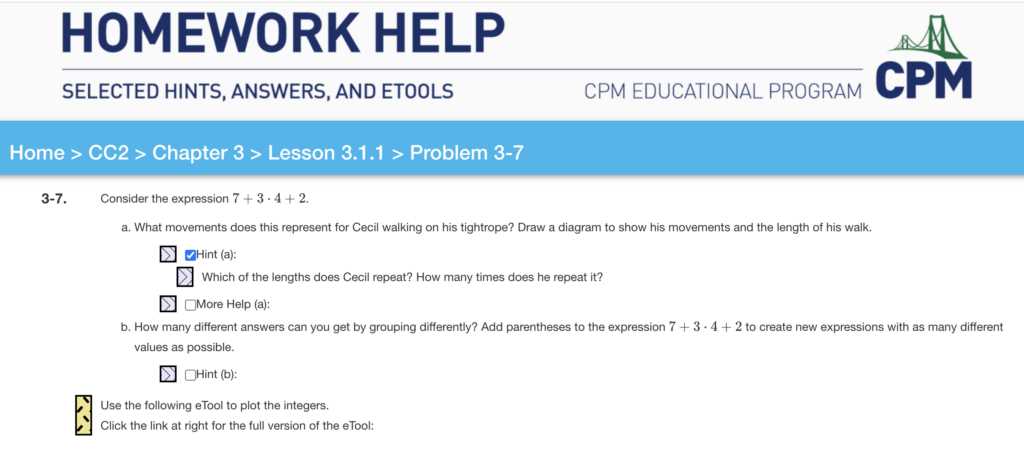
Understanding and solving algebraic equations is a fundamental skill that serves as the foundation for more advanced mathematical concepts. This section focuses on providing you with the tools and techniques necessary to master the process of solving equations efficiently and accurately. By breaking down complex problems into simpler steps, you will gain the confidence to tackle a wide range of mathematical challenges.
Key to mastering equations is recognizing the structure of the problem, selecting the appropriate method, and applying it consistently. Whether you’re dealing with linear equations, systems of equations, or other forms, the strategies outlined here will help you develop a solid approach to solving them.
Step-by-Step Techniques for Solving Equations
To solve algebraic equations effectively, follow these essential steps:
- Identify the equation type: Determine whether you are working with a linear equation, quadratic equation, or another type of algebraic expression.
- Isolate the variable: Use inverse operations to move terms with variables to one side and constants to the other side.
- Simplify the equation: Combine like terms and simplify both sides to make the equation easier to solve.
- Check your solution: Always substitute your solution back into the original equation to verify its accuracy.
Common Techniques for Solving Specific Types
Different types of equations may require unique strategies. Here are some approaches:
- Linear Equations: Solve by isolating the variable and performing basic operations.
- Quadratic Equations: Use factoring, completing the square, or the quadratic formula to find the roots of the equation.
- Systems of Equations: Solve using substitution or elimination methods to find the solution for all variables involved.
By practicing these steps and methods, you will build a deeper understanding of algebraic equations and develop the skills necessary to solve them with ease.
Quick Tips for Algebra 2 Success
Achieving success in advanced mathematical courses requires more than just understanding the basics. It’s about developing effective strategies and staying consistent in your practice. In this section, we’ll explore several tips that will help you tackle problems with confidence and improve your performance in the course.
By following these quick tips, you can maximize your understanding of the material and perform at your best throughout the course. From organizing your study routine to applying problem-solving techniques, these practices will give you a solid foundation for success.
| Tip | How It Helps |
|---|---|
| Practice regularly | Consistent practice helps reinforce concepts and improve problem-solving speed and accuracy. |
| Understand the fundamentals | A strong grasp of basic concepts allows you to approach more complex problems with ease. |
| Break problems into smaller parts | Dividing larger problems into manageable steps makes them easier to solve. |
| Ask questions | Clarifying doubts early helps prevent misunderstandings and keeps you on track. |
| Stay organized | Keeping notes and work organized allows you to track your progress and find patterns in solutions. |
By applying these tips, you can develop the skills and mindset needed to succeed. Stay focused, be persistent, and continually review your work to ensure long-term improvement and mastery of the material.
Breaking Down Complex Algebraic Expressions
Working with intricate mathematical expressions can seem daunting at first, but by breaking them down into simpler components, you can make them much more manageable. The key to mastering these expressions is to systematically apply foundational strategies that simplify each part of the equation. This approach not only helps with understanding but also with solving problems more efficiently.
In this section, we will explore effective techniques to tackle complicated expressions, focusing on how to isolate variables, combine like terms, and apply various operations. These strategies will help you approach even the most complex problems with confidence and accuracy.
Steps to Simplify Complex Expressions
Follow these essential steps to simplify complex mathematical expressions:
- Distribute terms: Apply the distributive property to remove parentheses and combine terms.
- Combine like terms: Group similar variables and constants together to simplify the expression.
- Factor when possible: Look for common factors and factor the expression to make it easier to work with.
- Isolate the variable: Rearrange the expression to isolate the variable on one side of the equation.
- Simplify fractions: If the expression contains fractions, simplify them by finding the greatest common denominator.
Helpful Strategies for Complex Problems
For more challenging problems, try the following techniques:
- Group terms strategically: When working with multi-variable expressions, group terms that have common factors to simplify the process.
- Substitute values: Substituting specific values for variables can sometimes make it easier to see patterns and solve the expression.
- Use exponents: Apply the laws of exponents to simplify expressions involving powers and roots.
By practicing these techniques and staying organized, you will be able to break down complex expressions and solve them step by step, making seemingly difficult problems much easier to tackle.
Efficient Methods for Algebra 2 Problem Solving
Mastering problem-solving techniques is crucial for success in advanced mathematics. The ability to approach complex tasks efficiently can save time and reduce errors. By adopting systematic methods and strategies, you can simplify difficult problems and solve them with confidence. This section will highlight several techniques that can enhance your problem-solving skills and help you tackle challenging exercises effectively.
Whether you’re dealing with equations, inequalities, or other mathematical forms, using efficient methods ensures you handle each step with clarity and precision. The following strategies will guide you through solving problems more quickly and accurately while minimizing the risk of mistakes.
Key Strategies for Efficient Problem Solving

To optimize your problem-solving process, consider applying these strategies:
- Understand the problem: Carefully read and analyze the problem to identify the key components and what is being asked.
- Break down the steps: Divide the problem into smaller, more manageable parts. Solving each part individually can simplify the overall process.
- Choose the right method: Depending on the problem type, select the most effective approach, whether it’s factoring, substitution, or using properties of exponents.
- Check your work: Always review your calculations and solutions to ensure no errors were made during the process.
Advanced Techniques for Challenging Problems
For more difficult problems, these additional techniques may be useful:
- Graphing: Visualizing the problem on a graph can help you understand relationships between variables and identify solutions more easily.
- Utilize substitution: When dealing with systems of equations, substitution can simplify the process by replacing variables step by step.
- Use of factoring: For complex expressions, factoring allows you to rewrite the equation in simpler forms, making it easier to solve.
By incorporating these methods into your problem-solving routine, you’ll be able to approach challenges with greater efficiency, saving time and ensuring accurate results. Mastering these techniques will lead to a more confident and systematic approach to mathematics.
How to Approach Word Problems in Algebra
Word problems can seem overwhelming, but breaking them down into smaller steps can help make them more manageable. These types of problems require translating real-world situations into mathematical expressions, which can then be solved using various techniques. The key is to approach each problem systematically and carefully, ensuring that every detail is accounted for.
In this section, we’ll discuss effective strategies for tackling word problems. By following these methods, you’ll be able to understand the problem, identify relevant information, and solve it efficiently.
Step-by-Step Process for Solving Word Problems
Here are the essential steps for solving word problems:
- Read the problem carefully: Understand the situation and identify the key information. Pay attention to what the problem is asking for and any numbers or relationships mentioned.
- Define the variables: Assign variables to represent unknown quantities. This makes it easier to set up an equation based on the information given.
- Translate words into math: Convert the word problem into an algebraic expression or equation. Use the relationships described in the problem to write mathematical statements.
- Set up an equation: Based on the translated information, create an equation that represents the problem. Ensure all relationships are accurately reflected.
- Solve the equation: Use algebraic methods to solve the equation for the unknown variable.
- Check your answer: Review the solution to ensure it makes sense in the context of the problem. Verify that the numbers and relationships align with the real-world situation.
Common Pitfalls to Avoid
While solving word problems, there are a few common mistakes to watch out for:
- Misinterpreting the problem: Always double-check that you understand what the problem is asking before proceeding.
- Overlooking important details: Pay attention to all the numbers and conditions given in the problem. Missing a small detail can lead to incorrect solutions.
- Not checking the solution: Once you’ve solved the problem, take a moment to review the answer and ensure it fits the context of the original problem.
By following these steps and being mindful of common errors, you will improve your ability to solve word problems with confidence and accuracy.
Key Formulas and Definitions to Remember

Having a solid grasp of essential formulas and concepts is crucial when solving mathematical problems. These key formulas serve as the foundation for various problem-solving techniques, enabling you to tackle a wide range of tasks with ease. In this section, we will highlight some of the most important mathematical principles and equations that you should keep in mind when working through problems.
Understanding and memorizing these formulas not only helps you solve problems faster but also allows you to apply them to different scenarios and equations. Below are some of the critical formulas and definitions that are essential for success in advanced mathematics.
Essential Mathematical Formulas
Here are some formulas that you should know by heart:
- Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a – Used for solving quadratic equations of the form ax² + bx + c = 0.
- Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²) – Used to find the distance between two points in a coordinate plane.
- Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) – Used to determine the slope of a line given two points on the line.
- Pythagorean Theorem: a² + b² = c² – Relates the lengths of the sides of a right triangle, where c is the hypotenuse.
Key Definitions to Remember
In addition to the formulas, it’s important to understand the following concepts:
- Linear Equation: An equation in which the highest exponent of the variable is 1. Example: y = mx + b.
- Exponential Growth: A process where the quantity increases at a constant rate over time, typically represented as y = a(1 + r)ⁿ.
- Factorization: The process of breaking down an expression into factors, such as factoring a polynomial into the product of binomials.
- Function: A relation between a set of inputs and outputs where each input is related to exactly one output.
By mastering these formulas and definitions, you’ll have the tools necessary to solve a wide variety of problems effectively. Regular practice with these concepts will help reinforce your understanding and improve your problem-solving skills.
How to Check Your Algebraic Solutions
Ensuring the accuracy of your mathematical solutions is an essential part of problem-solving. Verifying that your results are correct helps to reinforce your understanding and boosts confidence in your skills. In this section, we will explore several methods to check your solutions and make sure your calculations are accurate before finalizing your answers.
Checking your work involves looking for potential mistakes and ensuring that the solution fits the conditions of the problem. Below are some effective techniques that can help you validate your results.
Methods for Verifying Solutions
Here are some steps you can take to confirm the correctness of your solutions:
- Substitution: Substitute your solution back into the original equation to see if both sides are equal. If they are, your solution is correct.
- Graphing: Graph the equation and see if your solution corresponds to the correct point on the graph. For equations involving two variables, the solution should lie on the graph of the equation.
- Estimation: Use approximate values to estimate your solution and compare it to the exact result. If the two values are close, it suggests the solution is correct.
- Check Units: Ensure that the solution makes sense in the context of the problem. For example, if solving a real-world word problem, check that your result is logically consistent with the problem’s context.
Common Pitfalls to Avoid
While checking your work, be mindful of these common mistakes:
- Sign Errors: Watch out for sign changes during simplification or substitution steps. A small mistake with signs can lead to incorrect answers.
- Algebraic Manipulation Mistakes: Be careful when performing operations such as factoring, expanding, or simplifying expressions. Double-check your steps to avoid errors.
- Overlooking Multiple Solutions: Some equations, particularly quadratic ones, may have more than one solution. Make sure you’ve accounted for all possible solutions.
- Skipping Steps: Always work through every step of the solution process. Skipping even small steps can lead to missing key details in the solution.
By using these methods and avoiding common mistakes, you’ll be able to confidently check and confirm the accuracy of your solutions, improving both your mathematical skills and your problem-solving abilities.
Common Algebra 2 Strategies for Beginners
Starting with advanced mathematical concepts can feel overwhelming, but with the right strategies, it becomes much easier to navigate through. Developing a strong foundation in problem-solving techniques is crucial for mastering the more complex topics that arise later. In this section, we will explore effective methods to approach problems and simplify your understanding of challenging material.
By employing the right strategies, you can break down complex problems into manageable steps and build confidence as you progress. Below are several helpful tips and approaches for beginners to excel in their studies.
Helpful Strategies for Success
Here are some key strategies to help you tackle problems in this subject:
- Understand the Basics: Before diving into more difficult problems, make sure you have a strong grasp of basic concepts. This includes operations with numbers, solving simple equations, and recognizing patterns.
- Work Step-by-Step: Break down each problem into smaller, more manageable steps. This helps prevent feeling overwhelmed and allows you to focus on one part of the problem at a time.
- Use Visuals: Graphs, charts, and diagrams can be extremely helpful for visualizing equations and solutions. Drawing out a problem can give you a clearer understanding of its structure.
- Practice Regularly: The more you practice, the more familiar you become with common problem types. Set aside time for daily or weekly practice to reinforce your understanding.
- Ask for Help: Don’t be afraid to ask questions when you get stuck. Teachers, tutors, and online resources can offer valuable guidance and alternative ways of solving problems.
Common Mistakes to Avoid
While you focus on improving your skills, keep an eye out for these common pitfalls:
- Skipping Steps: Avoid rushing through problems. Skipping steps, even small ones, can lead to errors. Always work through every part of the problem carefully.
- Misinterpreting the Question: Read the problem carefully before solving. Often, beginners overlook key information that can guide them toward the correct solution.
- Not Checking Work: Double-check your final answer. Sometimes, a simple mistake can make the difference between a correct and incorrect solution.
By consistently applying these strategies and avoiding common mistakes, you’ll be well on your way to mastering advanced concepts and solving complex problems in no time.
Essential Skills for Solving Algebra 2 Problems
To succeed in solving complex mathematical problems, certain foundational skills are crucial. These skills help you approach challenges methodically and ensure accuracy in your calculations and solutions. Mastering these techniques not only simplifies the problem-solving process but also builds your confidence in tackling advanced problems with ease.
In this section, we will cover the most important skills every student needs to develop in order to excel. By refining these abilities, you will find yourself more prepared to face a variety of problem types and strategies effectively.
Key Skills for Effective Problem Solving
Here are the essential skills to focus on as you work through problems:
- Critical Thinking: The ability to analyze a problem and determine the best method for solving it is foundational. This involves understanding the underlying principles and recognizing which techniques apply to a given situation.
- Factoring and Simplifying Expressions: Being able to simplify and factor equations is a core skill that simplifies more complicated problems. Understanding how to break down expressions into simpler components will speed up your problem-solving process.
- Working with Functions: Knowing how to interpret and manipulate functions is key for solving a wide range of problems. This skill allows you to work with equations that describe relationships between variables.
- Managing Variables: Proficiency in handling variables is crucial. You should be comfortable isolating variables, substituting values, and solving for unknowns in various types of equations.
Tips for Mastering Problem-Solving Skills
While developing these skills, it’s important to keep in mind some additional tips that can further enhance your problem-solving abilities:
- Practice Regularly: The more you practice, the better you get. Consistent practice with different problem types will help you internalize the methods and strategies you need.
- Review Mistakes: After solving a problem, always review your work. Identifying where mistakes were made helps improve understanding and prevents the same errors from recurring.
- Learn from Examples: Look at solved examples to understand the step-by-step approach. This can help you visualize how to approach similar problems in the future.
By developing these key skills and applying them regularly, you’ll be able to solve problems more efficiently and confidently. These techniques serve as the building blocks for mastering more advanced concepts as you progress.
Improving Your Problem-Solving Speed
Enhancing your speed in solving mathematical problems is a vital skill, especially when dealing with complex tasks or time-sensitive tests. The faster and more efficiently you can apply the right methods, the more confident you will become in your abilities. This section explores techniques that can help you boost your problem-solving speed while maintaining accuracy.
By focusing on key strategies, practicing specific methods, and eliminating common hurdles, you can significantly improve the time it takes to complete problems. Speed is not just about rushing; it’s about becoming more efficient and strategic in your approach to each problem.
Effective Strategies for Faster Problem Solving
Here are some strategies that can help you solve problems more quickly:
- Understand the Basics: Having a solid grasp of foundational concepts will allow you to recognize patterns and apply shortcuts effectively. This foundational knowledge will make it easier to identify the most efficient method for each problem.
- Break Problems into Smaller Steps: Large, complex problems can be overwhelming. Breaking them down into smaller, manageable steps will help you solve them more quickly and reduce the chances of mistakes.
- Use Mental Math: For simple calculations, try to use mental math whenever possible. This eliminates the need to write down every step and saves valuable time.
- Familiarize Yourself with Common Methods: Practice solving problems using the most common methods and techniques. The more familiar you are with these approaches, the quicker you’ll be able to identify and apply them when necessary.
Tips for Practicing Speed
Here are some additional tips to improve your speed through regular practice:
- Set Time Limits: When practicing, set a time limit for each problem. This encourages you to solve the problem as efficiently as possible and helps you learn to manage time effectively.
- Track Your Progress: Keep track of how long it takes you to solve problems and note areas where you’re slow. Focus on improving those areas through targeted practice.
- Work on Different Problem Types: Exposure to a variety of problem types will help you build speed by making it easier to recognize which approach works best for different scenarios.
By following these strategies and consistently practicing, you can improve your problem-solving speed. Remember, the goal is not just to be fast but to be accurate while solving problems in less time. Speed will naturally improve as you become more familiar with the process and develop confidence in your skills.
Analyzing the Structure of Algebraic Equations
Understanding the framework of mathematical equations is a crucial step in solving them efficiently. By breaking down the structure of these equations, you can identify key elements and relationships that will guide you toward the correct solution. This section will help you focus on how to analyze and interpret equations more effectively.
Each equation is composed of variables, constants, and operations, and understanding how these parts interact is essential for solving the equation. By identifying patterns in how terms are arranged, you can develop strategies that allow you to approach problems with more precision and confidence.
Identifying Key Components
To analyze an equation thoroughly, start by recognizing its key components:
- Variables: These are the unknown values represented by symbols, often denoted by letters like x or y. Identifying them helps you understand what the equation is solving for.
- Constants: Constants are fixed values within the equation, typically numbers. Recognizing constants allows you to focus on how they interact with the variables.
- Operators: These symbols (+, -, *, /) show the operations performed between variables and constants. Understanding the role of each operator is essential in determining how the terms of the equation relate to one another.
Examining the Relationships
Once you have identified the components of the equation, focus on how they relate:
- Order of Operations: Understanding the order in which operations should be performed (PEMDAS/BODMAS) is essential for simplifying and solving equations correctly.
- Balancing the Equation: Analyzing the equation requires keeping both sides balanced. If one side changes, the other side must be adjusted in the same way to maintain equality.
- Isolating Variables: In many cases, solving for a variable involves isolating it on one side of the equation. Recognizing the most efficient way to do this can significantly reduce solving time.
By thoroughly analyzing the structure of mathematical equations, you can approach problems more systematically. This will help you identify patterns, use the most effective methods, and ultimately solve the equations with greater ease and accuracy.
Review of Key Topics in the First Section
The first section introduces essential mathematical concepts and provides a foundation for more complex problem-solving techniques. It focuses on the fundamental ideas that help develop a deeper understanding of mathematical structures and how they are applied in various contexts. This review will summarize the main topics covered and offer insights into how each concept builds upon the other for solving more advanced problems.
At the core of this section, the focus is on foundational techniques such as simplifying expressions, solving linear equations, and understanding the relationships between different mathematical elements. Mastering these topics will prepare you to tackle more intricate tasks with confidence and efficiency.
Key Concepts Covered
The following concepts form the basis of this section:
- Understanding Variables and Constants: Identifying and working with unknown values and fixed quantities.
- Operations on Expressions: Simplifying and manipulating algebraic expressions using appropriate mathematical operations.
- Equations and Solutions: Solving linear equations, balancing both sides, and interpreting the solutions.
- Graphing Relationships: Using graphs to visually represent relationships between variables and understand trends and behaviors.
Building Blocks for Success
As you progress through these topics, each new concept adds a layer of complexity, requiring a solid grasp of previous material. The review focuses on reinforcing your understanding of the following:
- Effective Problem-Solving Strategies: Identifying the best approaches for various types of equations and problems.
- Critical Thinking and Pattern Recognition: Developing the ability to identify patterns and relationships between mathematical elements, which helps in making predictions and solving equations efficiently.
- Practice and Application: Reinforcing concepts through practice problems to improve speed and accuracy in solving equations.
By reviewing these core topics, you will be better prepared to approach more advanced mathematical challenges and build a strong foundation for future learning.