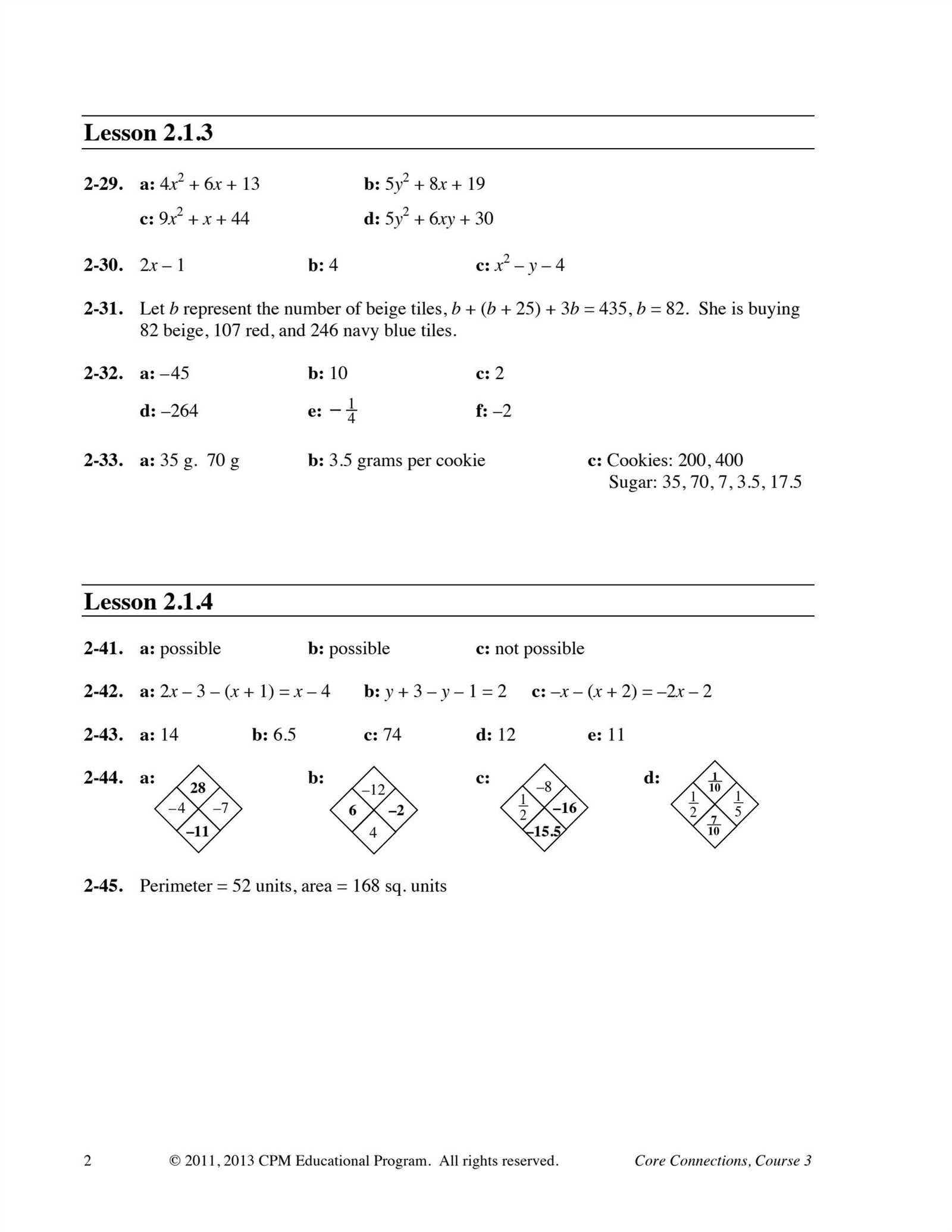
Understanding foundational mathematical principles is crucial for students looking to excel in problem-solving and critical thinking. As learners navigate complex exercises, having the right strategies and methods can significantly improve both comprehension and performance. This section aims to provide clear solutions, step-by-step explanations, and effective techniques for tackling challenging topics in mathematics.
By focusing on essential skills, such as simplifying expressions, solving equations, and interpreting word problems, students can build a solid foundation. The following sections will guide you through different types of exercises and demonstrate how to approach each one logically. With consistent practice, mastering these techniques will lead to greater confidence and success in mathematical tasks.
Core Connections Algebra 1 Answers Overview
This section provides a detailed look at how to approach and solve common problems found in introductory mathematics courses. Understanding the structure of exercises, recognizing patterns, and applying key strategies are essential for mastering concepts and achieving success in solving complex tasks. Here, we will explore essential methods and tools to guide students through their learning journey.
Students often face difficulties with the organization and application of mathematical principles. With the right approach, however, these challenges can be overcome. By focusing on breaking down problems into manageable steps and using logical reasoning, learners can effectively tackle a wide variety of problems.
- Identifying the main concepts and operations involved
- Recognizing common problem types and structures
- Breaking down multi-step problems into simpler parts
- Understanding how different methods can be applied to solve similar issues
- Building confidence through consistent practice and problem-solving
Ultimately, mastering these techniques will lead to greater proficiency in solving math exercises and understanding how mathematical concepts relate to real-world situations. By practicing these methods regularly, students can significantly improve their skills and become more efficient problem solvers.
Understanding Algebra 1 Key Concepts
Mastering the foundational principles of mathematics is essential for solving complex problems. A deep understanding of core topics allows students to approach exercises logically and efficiently. This section covers the most important concepts that students need to grasp in order to excel in their studies, from basic equations to more advanced problem-solving techniques.
Fundamentals of Mathematical Expressions
One of the key elements in mastering mathematics is understanding how to manipulate and simplify expressions. This includes identifying variables, constants, and coefficients, and learning how to combine them correctly. Whether it’s linear, quadratic, or polynomial expressions, recognizing their structure is essential to solving problems efficiently.
Equations and Inequalities
Solving equations and inequalities is another critical skill. By isolating variables and applying inverse operations, students can solve for unknowns in a variety of contexts. It’s important to understand the relationship between different types of equations and how they are solved differently, whether it’s through balancing equations or graphing inequalities.
Step-by-Step Algebra 1 Answer Guide
To solve mathematical problems effectively, it’s crucial to break down each task into smaller, manageable steps. This approach helps identify the key components of the problem and ensures that no steps are missed. By following a structured process, students can methodically approach each problem and gain a deeper understanding of the underlying concepts.
Step 1: Identify the Problem Type
Before beginning, it’s essential to recognize the type of problem you’re dealing with. Whether it’s a simple equation or a more complex expression, identifying the correct method to apply will help guide your solution process.
Step 2: Isolate Variables
Once the problem type is identified, the next step is to isolate the unknowns. Use inverse operations to move constants or coefficients away from the variable to simplify the equation.
| Operation | Effect on Equation |
|---|---|
| Adding/Subtracting | Move constants to one side |
| Multiplying/Dividing | Isolate the variable |
| Applying Inverse Operations | Simplify equation step-by-step |
Step 3: Solve and Check

After isolating the variable, solve the equation and substitute the value back into the original problem to verify the correctness of your solution. This final check ensures that your calculations are accurate and that no mistakes were made during the process.
Common Mistakes in Algebra 1
When tackling mathematical problems, it’s easy to make mistakes that can derail the entire solution process. Many of these errors stem from misunderstanding the problem or applying the wrong technique. By identifying and addressing these common pitfalls, students can improve their problem-solving skills and avoid frustration.
Misunderstanding the Order of Operations
One of the most frequent mistakes is not following the proper order of operations. It’s essential to handle parentheses, exponents, multiplication and division, and addition and subtraction in the correct sequence to arrive at the right result. Ignoring this can lead to significant errors, especially in more complex expressions.
Incorrectly Solving for Variables
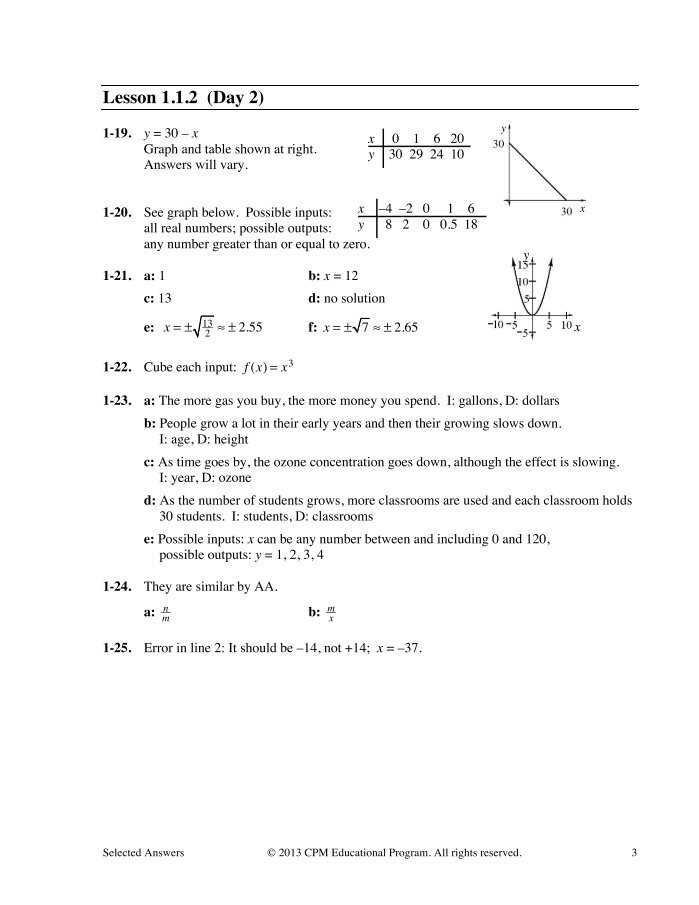
Another common issue occurs when students incorrectly isolate or solve for variables. This can happen when terms are not properly moved across the equal sign or when inverse operations are used incorrectly. Always double-check your steps to ensure the variable is isolated correctly before solving.
Mastering Variables and Equations
Understanding how to work with unknown quantities and solve equations is a fundamental skill in mathematics. Variables represent unknown values, and equations establish relationships between them. Mastering how to manipulate and solve these relationships is key to building a solid mathematical foundation. By gaining proficiency in these areas, students can solve a wide variety of problems with confidence and accuracy.
Step 1: Identifying Variables

Variables typically represent unknown values that we need to solve for. Recognizing how to identify and label variables correctly is the first step in solving an equation. Understanding their role within an equation will help determine the correct approach for solving it.
Step 2: Solving Equations
Solving equations involves isolating the variable to find its value. This process typically requires applying inverse operations to move constants and coefficients, simplifying the equation step by step. Below is a basic outline of the process for solving a simple equation:
| Step | Action | Example |
|---|---|---|
| 1 | Isolate the variable term | 2x + 3 = 11 → 2x = 8 |
| 2 | Apply inverse operations | 2x = 8 → x = 4 |
| 3 | Check the solution | 2(4) + 3 = 11 → 8 + 3 = 11 |
By following these steps and practicing regularly, students can confidently solve equations and manipulate variables with ease.
Tips for Tackling Word Problems
Word problems can often be the most challenging type of exercise because they require translating real-world scenarios into mathematical expressions. However, with a systematic approach, these problems can become more manageable. The key to success is understanding the question, breaking it down into smaller parts, and then applying the appropriate mathematical techniques to solve it.
Step 1: Read and Understand the Problem
Before attempting to solve a word problem, it’s important to carefully read through the entire problem to ensure that you understand what is being asked. Focus on the key details and identify what information is given and what needs to be found. Often, highlighting important numbers or phrases can help clarify the problem.
Step 2: Translate the Words into Equations
Once the problem is understood, the next step is to convert the word problem into a mathematical equation. This might involve identifying the variables, constants, and operations that need to be used. Here’s an example of how to translate a word problem:
| Word Problem | Mathematical Equation |
|---|---|
| John has 5 more apples than Sarah. Together, they have 15 apples. How many apples does each person have? | x + (x + 5) = 15 |
By setting up the equation properly, you can now solve for the unknowns systematically.
Step 3: Solve and Verify
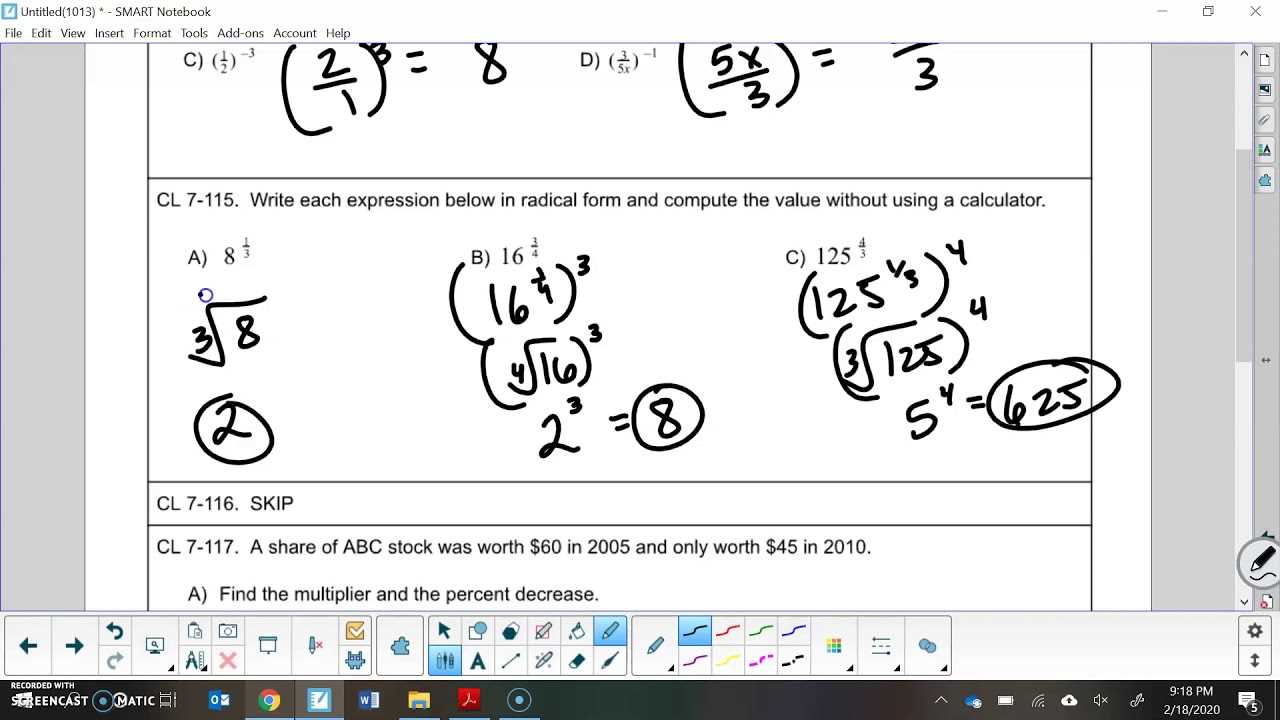
After setting up the equation, solve for the unknown variables. Once a solution is found, it’s important to plug the values back into the original word problem to verify the accuracy of the solution. This ensures that the answer makes sense within the context of the problem.
Strategies for Algebra 1 Success
Achieving success in mathematics requires more than just solving equations; it involves developing effective strategies that help students understand key concepts, avoid common pitfalls, and build confidence. By following a systematic approach, learners can improve their problem-solving abilities and excel in more complex tasks. This section outlines key strategies that will help students master important skills and perform well in their studies.
One of the most important strategies is consistent practice. Regularly solving problems helps reinforce concepts and makes techniques feel more intuitive. Additionally, breaking down problems into smaller, more manageable steps can help simplify complex exercises and ensure no key elements are overlooked.
Another effective strategy is seeking help when needed. Don’t hesitate to ask for clarification or assistance from teachers, tutors, or peers. Sometimes a different perspective can provide insight into a problem, making it easier to understand and solve. Collaboration and discussing challenging concepts with others can deepen understanding and foster a stronger grasp of mathematical principles.
Lastly, staying organized is crucial. Keeping notes, diagrams, and example problems neatly arranged can serve as valuable resources when reviewing material or working through exercises. Being organized helps track progress and quickly locate key concepts when revisiting previous lessons or tackling new problems.
How to Check Your Solutions
Verifying your solutions is a crucial part of problem-solving. It ensures that the answer you arrived at is correct and consistent with the given problem. Whether you’re solving equations, word problems, or other mathematical tasks, checking your solutions helps catch mistakes and reinforces understanding of the concepts. This section outlines effective methods to verify your answers.
Step 1: Substitute the Solution Back
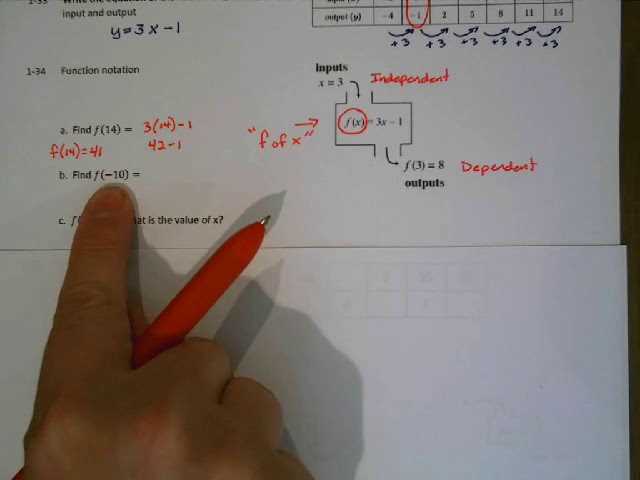
The most straightforward method to check your work is to substitute the solution back into the original equation or problem. This allows you to confirm whether the solution satisfies all parts of the problem.
- For equations: Substitute the value of the variable into the original equation and check if both sides are equal.
- For word problems: Re-read the problem with your solution in place to ensure it makes sense in the context of the question.
Step 2: Use Reverse Operations
For more complex problems, you can use reverse operations to verify your work. Reversing the operations used to solve the problem should lead you back to the original expression or equation.
- Start with your final result and work backward step by step.
- Ensure that each operation aligns with the inverse of the steps taken during the solution process.
By carefully reviewing your work and applying these verification techniques, you can ensure the accuracy of your solutions and avoid common errors.
Essential Algebra 1 Skills to Develop
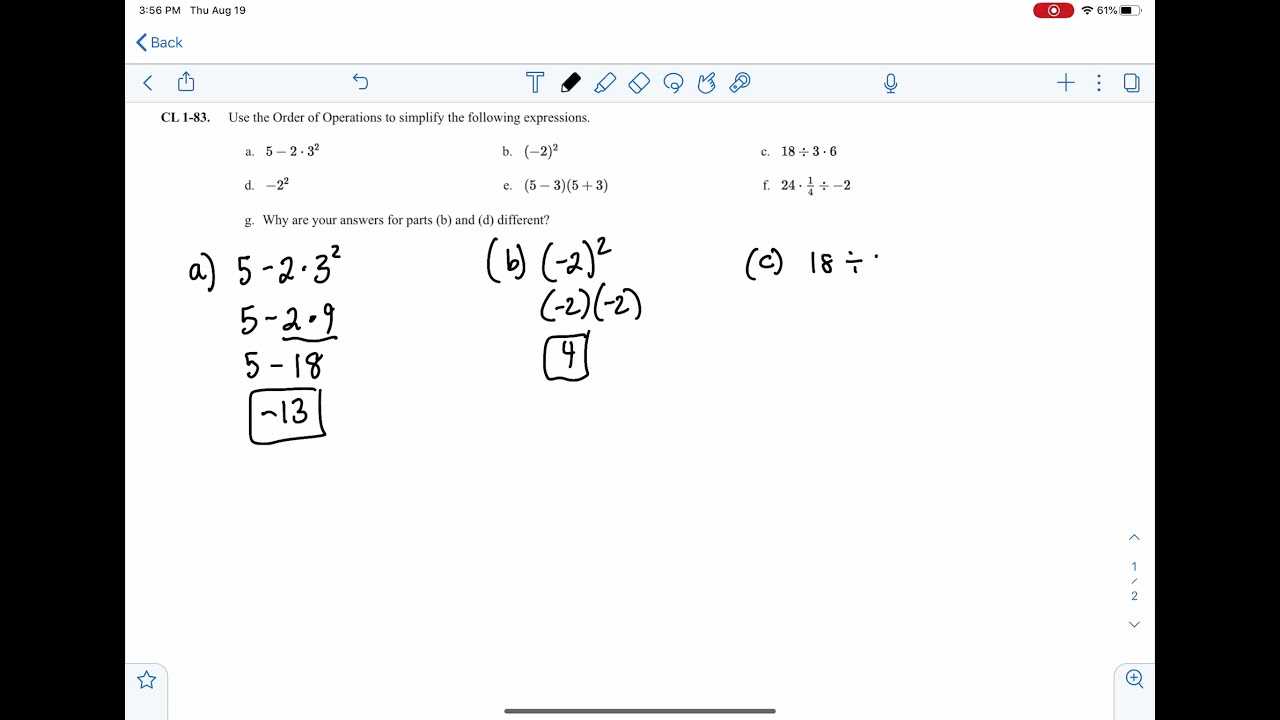
To excel in mathematics, particularly when solving equations and working with variables, it’s important to focus on building a strong foundation of essential skills. These skills not only help in solving specific problems but also lay the groundwork for more advanced topics. Mastering these key abilities will make solving mathematical tasks quicker and more intuitive.
1. Simplifying Expressions

One of the most fundamental skills is simplifying expressions. This involves combining like terms, reducing fractions, and applying the distributive property. A solid understanding of how to simplify expressions will make solving more complex equations much easier and faster.
2. Solving Linear Equations

Another critical skill is solving linear equations. This includes isolating the variable, applying inverse operations, and working with both one-variable and multi-variable equations. Becoming proficient at solving these types of problems builds confidence and prepares you for more complex equations in the future.
By consistently practicing these basic skills, you’ll develop a stronger mathematical mindset and be better equipped to solve a variety of problems with confidence.
Practice Questions for Algebra 1
Regular practice is essential for mastering key mathematical concepts. Working through different types of problems helps reinforce your understanding and improve problem-solving skills. In this section, you’ll find a series of practice questions that target various areas of mathematics, from solving equations to working with expressions. These exercises will help you test your knowledge and identify areas where you might need further practice.
Basic Equation Solving
Start with these simple problems to build confidence in solving linear equations:
| Problem | Solution |
|---|---|
| 2x + 5 = 15 | x = 5 |
| 3x – 7 = 11 | x = 6 |
| 5x + 4 = 19 | x = 3 |
Working with Expressions

Next, try these problems that involve simplifying and combining like terms:
| Problem | Solution |
|---|---|
| 4x + 3x – 2 | 7x – 2 |
| 2(3x + 4) | 6x + 8 |
| 5x – 3(x + 2) | 2x – 6 |
By solving these problems, you’ll strengthen your ability to simplify expressions and solve basic equations quickly and accurately.
Using Resources to Improve Scores
To achieve better results in mathematics, it’s essential to make use of various resources that can aid in understanding and practice. These resources can range from textbooks and online tools to tutoring sessions and study groups. By utilizing these materials effectively, students can reinforce their knowledge, clarify difficult concepts, and enhance their problem-solving skills. Taking advantage of available resources can make a significant difference in overall performance.
One of the most helpful resources is practice exercises, which provide an opportunity to apply learned concepts in different contexts. Online platforms, such as interactive quizzes and problem solvers, offer immediate feedback, helping students identify mistakes and improve quickly. Additionally, seeking assistance from teachers or tutors can provide personalized support and targeted guidance in areas where students struggle the most.
Another valuable tool is collaborative study. Joining study groups or participating in discussions allows students to share strategies, explain concepts to one another, and tackle problems collectively. This collaborative learning can deepen understanding and encourage students to think critically about mathematical problems.
By actively using these resources, students can improve their confidence, grasp challenging material, and ultimately enhance their scores in mathematical assessments.
Algebra 1 Answer Key Breakdown
Understanding the structure of solution keys is essential for properly evaluating your work and improving your skills. An answer key not only provides the correct solutions but also highlights the steps taken to reach those solutions. By reviewing the breakdown of each answer, students can identify where they went wrong and learn how to approach problems more effectively in the future.
The key to successfully using an answer key is to carefully analyze each solution. This involves going beyond just checking the final answer and paying attention to the steps, operations, and logic used in the process. Here’s how to effectively break down the answer key:
Steps to Review an Answer Key
- Compare Each Step: Check if the method used to solve the problem aligns with what was taught. This helps confirm whether you followed the right process.
- Identify Mistakes: If the solution differs from your result, examine where the error occurred. Did you make a calculation mistake, or did you overlook a step?
- Understand the Method: Focus on the reasoning behind each operation. Knowing why certain steps are taken reinforces your understanding of the concept.
- Use the Key as a Learning Tool: Don’t just check the answers–use the key to enhance your learning. By understanding the solution process, you can apply it to similar problems.
Common Errors to Watch for in an Answer Key
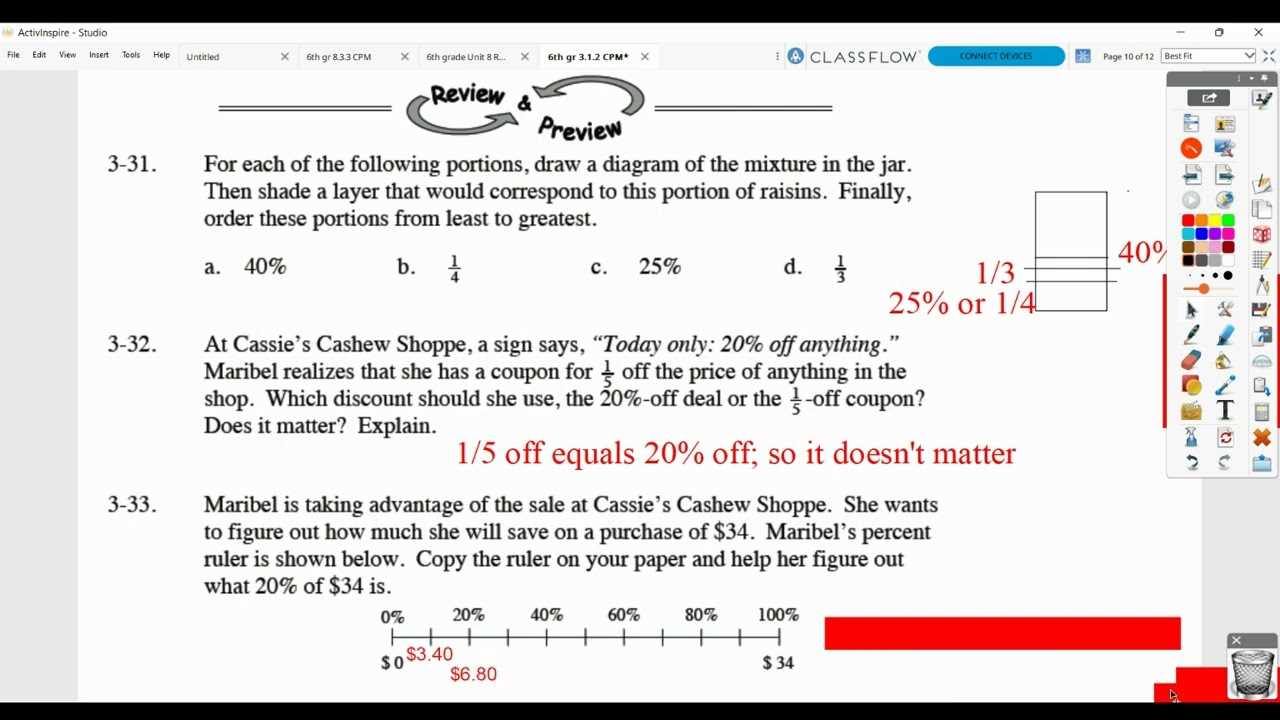
- Not applying the correct order of operations.
- Missing or incorrect distribution of terms.
- Incorrectly solving for variables or not isolating them properly.
By reviewing an answer key thoroughly, you can better understand your mistakes and improve your problem-solving process, leading to stronger skills and higher success in future challenges.
Common Algebraic Formulas You Need
To solve mathematical problems efficiently, it’s crucial to have a solid grasp of the essential formulas that frequently appear in equations. These formulas serve as tools to simplify complex problems and make calculations easier. Whether you’re solving for unknowns, working with systems, or simplifying expressions, knowing these fundamental relationships can significantly improve your problem-solving abilities.
1. The Distributive Property
The distributive property is one of the most widely used formulas. It allows you to simplify expressions by multiplying a number outside the parentheses by each term inside. The formula is:
a(b + c) = ab + ac
This property is essential for expanding and simplifying expressions, especially when working with polynomials.
2. The Quadratic Formula
The quadratic formula is used to solve quadratic equations, or equations in the form of ax² + bx + c = 0. It provides the solutions for the variable x and is expressed as:
x = (-b ± √(b² – 4ac)) / 2a
This formula is invaluable when factoring is not possible, and it ensures that you can always find solutions for quadratic equations, whether they are real or complex.
Mastering these and other algebraic formulas will enable you to approach problems with more confidence and solve them more efficiently. Having a mental reference to these formulas allows you to focus on applying them correctly and solving equations faster.
How to Approach Algebra 1 Tests
When preparing for math exams, it’s important to have a strategy that maximizes your efficiency and accuracy. A well-thought-out approach not only helps you manage your time but also ensures that you thoroughly understand the material being tested. By following a systematic method, you can tackle even the most challenging problems with confidence.
Step 1: Review Key Concepts
Before the test, make sure to review the most important concepts and formulas that you’re likely to encounter. Focus on areas where you feel less confident, and ensure you understand how to apply key strategies.
- Practice solving equations and simplifying expressions.
- Familiarize yourself with the common types of problems that appear on past tests.
- Understand the properties of operations and their applications in problem-solving.
Step 2: Time Management During the Test

Efficiently managing your time during the test is crucial. Here are some tips to stay on track:
- Scan the entire test: Quickly glance through all questions before starting to identify easier problems and allocate time accordingly.
- Start with the known: Answer questions you feel most confident about first to build momentum.
- Don’t spend too long on one problem: If you get stuck, move on and return to the challenging question later.
By reviewing the material beforehand and practicing time management during the test, you can approach your math exams more effectively and increase your chances of success.
How Algebra 1 Answers Help Learning
Reviewing the solutions to mathematical problems plays a key role in reinforcing understanding and improving problem-solving skills. By analyzing the steps leading to the correct result, students can identify patterns, recognize errors, and learn how to approach similar problems in the future. This process is essential for deepening comprehension and solidifying the foundation needed for more advanced topics.
When you study solutions, it’s important to focus not just on the final answer but also on the method used to reach it. Understanding why certain steps are taken helps students develop critical thinking and problem-solving strategies. Furthermore, when students make mistakes, seeing the correct approach highlights the areas where they may have gone wrong, allowing them to adjust their thinking for future problems.
By continuously reviewing and reflecting on the provided solutions, learners can improve their overall mathematical abilities. This not only boosts confidence but also encourages a more active learning process, where students feel equipped to solve a wide variety of problems on their own.