In this part of the learning process, students engage with a set of practice exercises designed to enhance their problem-solving abilities. These tasks provide a structured way to review and reinforce the fundamental ideas required to succeed in mathematics. Through this method, learners not only test their understanding but also refine their approach to tackling mathematical challenges.
As you work through the exercises, the focus is on honing skills and identifying areas that need improvement. It’s essential to approach each problem with the intent to master the underlying concepts, which will become vital as you progress through more advanced topics. Regular practice allows for a deeper grasp of the material and the development of strategies for solving complex equations.
By reviewing the solutions, students gain valuable insights into the correct methodologies and thought processes. This feedback helps to correct mistakes and clarify doubts, ultimately contributing to a stronger mathematical foundation. The more you practice and engage with these exercises, the more confident and proficient you’ll become in tackling a wide range of problems.
Algebra Nation Section 1 Test Yourself Answers
In this part of the curriculum, learners are provided with a series of exercises aimed at evaluating their comprehension of the core principles. The goal is to reinforce understanding and highlight areas that may require further attention. Each exercise challenges students to apply their knowledge in practical ways, offering them the opportunity to self-assess their skills and improve over time.
To fully benefit from these practice activities, it’s crucial to approach them systematically. Below are key strategies to consider when reviewing the solutions:
- Review each step carefully: Examine the process behind each solution to understand how it was reached. This helps in mastering the method used to solve similar problems.
- Identify common errors: By analyzing mistakes, you can prevent them from occurring again in future tasks. Understanding where and why errors happen is crucial for growth.
- Focus on the underlying concepts: Each problem is designed to test specific ideas. Be sure to review the theory behind each question to strengthen your overall comprehension.
Additionally, comparing your responses with the provided solutions is a valuable way to spot areas for improvement. This comparison not only reveals any gaps in your knowledge but also encourages deeper engagement with the material.
As you continue to practice, make sure to challenge yourself with progressively harder exercises. This will help solidify your understanding and increase your confidence in tackling more complex problems.
Understanding the Algebra Nation Test
This section provides an overview of the exercises designed to evaluate your mathematical understanding. These tasks are structured to help you assess how well you have grasped the key concepts. The goal is not just to complete problems, but to refine your approach to solving various types of questions efficiently.
The main purpose of these exercises is to provide a deeper understanding of how mathematical principles are applied in real scenarios. They allow students to gauge their progress and identify areas that may need further attention or practice. Here’s how to make the most of these opportunities:
- Familiarize with the structure: Each activity is crafted to target specific skills. Understanding the format helps you approach them with more confidence.
- Analyze problem types: Different types of questions test different aspects of your skills. Take note of which problem types are challenging and focus on improving those areas.
- Practice critical thinking: These tasks are designed to develop your analytical skills. Always aim to understand the reasoning behind each step, rather than just memorizing solutions.
By recognizing patterns and strategies, you will gradually improve your ability to tackle more complex tasks. The exercises are an essential part of your mathematical development, reinforcing both basic and advanced techniques in a structured manner.
How to Approach Section 1 Questions
When tackling mathematical exercises, it’s essential to approach each problem strategically to ensure accurate and efficient solutions. By breaking down each question into manageable steps, you can avoid feeling overwhelmed and increase your chances of success. The key is not just finding the correct answer, but understanding the reasoning behind each solution.
Understand the Problem
Before attempting to solve any question, take a moment to carefully read and analyze it. Identify the key information and determine what is being asked. Understanding the problem’s context and the requirements will guide your approach and help you stay focused on relevant concepts.
Plan Your Approach
Once you’ve grasped the problem, decide on the best method to solve it. Consider the tools and formulas you might need. Make sure to work through the problem step by step, keeping track of intermediate results. This methodical approach not only helps prevent errors but also ensures that you’re applying the correct principles throughout.
By practicing these strategies, you’ll develop a more confident and organized approach to solving problems. Always review your work and verify your solutions to further improve your skills.
Key Concepts in Algebra Section 1
This part of the curriculum focuses on the foundational principles that are essential for understanding and solving mathematical problems. Mastering these concepts is crucial for building a strong base, enabling students to tackle increasingly complex topics with confidence. The key ideas covered here serve as the building blocks for more advanced techniques and applications.
The core concepts introduced include fundamental operations, relationships between variables, and the structure of equations. A clear grasp of these topics is necessary not only for completing exercises but for applying problem-solving strategies in a wide variety of contexts.
By focusing on these fundamental principles, students can improve their problem-solving abilities, laying the groundwork for success in subsequent areas of study. It’s important to take the time to fully understand these concepts before moving forward, as they are integral to more advanced mathematical reasoning.
Step-by-Step Guide to Solving Problems
When faced with a mathematical challenge, it’s important to approach the problem methodically to ensure accuracy and clarity. Breaking down each task into smaller, manageable steps not only makes the solution more achievable but also helps in developing a deeper understanding of the underlying concepts. This structured approach is key to solving even the most complex problems with confidence.
Step 1: Analyze the Problem
Start by thoroughly reading the question. Identify the key information and determine what is being asked. Highlight or underline important numbers, variables, or operations. Understanding what the problem requires is the first step in determining how to approach it.
Step 2: Plan Your Approach

After understanding the problem, decide on the method or formula that best fits the situation. Consider the relationships between the variables and how they influence each other. Break the problem into smaller parts if needed and choose the appropriate strategy to move forward.
By following this step-by-step guide, you can approach each problem with a clearer mindset and greater precision. Over time, this method will help you become more efficient and accurate in solving a wide range of mathematical challenges.
Common Mistakes in Section 1 Exercises
While working through mathematical exercises, it’s common to make errors that can hinder progress. Recognizing and understanding these mistakes is crucial for improvement. Identifying patterns in the types of errors made can help avoid them in the future, leading to more accurate and efficient problem-solving.
Some of the most frequent mistakes include misinterpreting the problem, skipping crucial steps, and applying incorrect formulas. It’s easy to rush through calculations without double-checking, which often leads to simple yet avoidable errors. Additionally, not fully understanding the relationships between variables can lead to flawed reasoning and incorrect solutions.
To minimize mistakes, it’s important to take your time and ensure each step is followed carefully. Reviewing your work before finalizing your answer can help catch overlooked errors and solidify your understanding of the material.
Effective Strategies for Answering Questions
When facing any kind of problem, developing a systematic approach is essential for providing clear and correct solutions. By applying well-organized strategies, you can ensure that each question is answered efficiently and accurately. These strategies not only improve your problem-solving skills but also boost confidence during the learning process.
Plan Before You Solve
Start by reading the problem carefully and breaking it down into smaller components. Organize the information before jumping to the solution. Here are a few tips to help with planning:
- Identify key details: Look for important numbers, relationships, or operations that will guide your solution.
- Determine the method: Choose the appropriate strategy or formula based on the type of problem you’re solving.
- Outline your steps: Write down a rough plan for solving the problem step by step, so you don’t miss anything important.
Check and Review Your Work
Once you’ve solved the problem, always review your solution before finalizing it. Cross-check your calculations, verify your logic, and ensure that the final result makes sense. This extra step helps catch any minor mistakes and ensures a thorough understanding of the material.
By following these strategies, you can approach each problem with clarity, improving both your accuracy and confidence in solving similar tasks in the future.
Tips for Time Management in Tests
Effective time management during exams is essential for achieving the best possible results. By organizing your approach and allocating time wisely, you can ensure that you have enough time to address each problem thoroughly while avoiding the stress of rushing. Developing strategies for managing time helps maximize efficiency and minimizes errors caused by pressure.
Here are some practical tips for managing your time during assessments:
| Strategy | Description |
|---|---|
| Plan Ahead | Before you begin, quickly glance through the entire exam to understand the types of questions and how much time to spend on each section. |
| Set Time Limits | Assign specific time limits to each problem or section based on its difficulty. Stick to these limits to avoid spending too much time on any one question. |
| Start with Easy Questions | Begin with the questions you feel most confident about. This allows you to build momentum and ensures you score easy points before moving to more difficult problems. |
| Skip and Return | If you get stuck on a question, move on and return to it later. This keeps you from losing valuable time and helps you stay on track. |
| Review Your Work | Save a few minutes at the end to review your answers, making sure you’ve solved everything correctly and haven’t missed any questions. |
By implementing these time management strategies, you can approach each exam with greater confidence and efficiency, ensuring that you make the most of the time you have available.
How to Check Your Algebra Answers
Verifying your work is a crucial step in ensuring that your solutions are correct and that you haven’t overlooked any errors. Taking the time to carefully check each step can make the difference between a correct result and a simple mistake. By applying specific strategies, you can effectively identify potential mistakes and improve the accuracy of your results.
Here are a few methods you can use to verify your calculations:
- Review Each Step: Go back through your work to ensure each step follows logically from the previous one. Pay attention to small details such as signs and operations that may have been missed or misapplied.
- Substitute the Solution: If possible, substitute your final result back into the original equation or problem to see if it holds true. This can be a quick way to check your work.
- Double-Check Calculations: Recalculate key values, especially those that are critical to the solution. Sometimes a simple miscalculation can lead to a wrong answer.
- Cross-Verify with Another Method: If applicable, try solving the problem using a different approach. If both methods yield the same result, it increases the likelihood that your solution is correct.
By implementing these verification techniques, you can boost your confidence in your solutions and catch any mistakes before finalizing your work.
Improving Accuracy with Practice
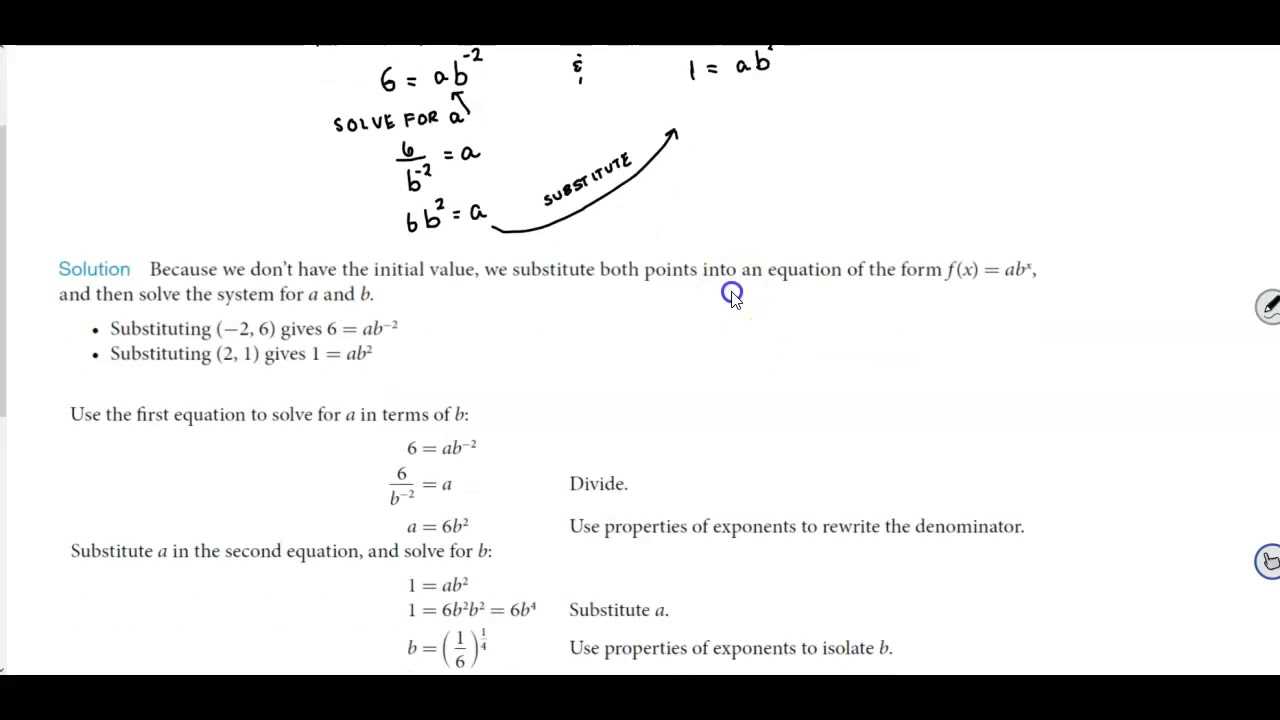
Consistent practice is the key to enhancing precision and mastering mathematical concepts. The more you engage with problems, the better you become at recognizing patterns, avoiding common mistakes, and applying the right techniques. By regularly solving problems, you develop the skills necessary to approach challenges with greater confidence and accuracy.
Build a Strong Foundation
Before diving into complex problems, make sure you have a solid grasp of the fundamental concepts. Strong foundations allow you to tackle more difficult tasks without getting overwhelmed. Start by practicing simple problems and gradually increase the complexity as you become more comfortable.
Review and Reflect on Mistakes
It’s not enough to just complete exercises; reviewing your mistakes is crucial to improving your accuracy. After solving each problem, take time to analyze where you went wrong and what led to the error. This reflection process helps you learn from your mistakes and prevents similar issues in the future.
With dedicated practice and self-reflection, you’ll notice steady improvements in your accuracy, allowing you to solve problems more efficiently and confidently.
Resources for Algebra Nation Practice
Having access to the right resources is essential for strengthening your understanding and improving your problem-solving skills. A variety of tools and platforms can help you practice effectively, providing both challenges and support as you work through different types of problems. These resources are designed to complement your learning and guide you through each stage of mastering key concepts.
Here are some useful materials to enhance your practice:
- Interactive Online Platforms: Many websites offer interactive exercises and quizzes that allow you to practice problems in real-time. These platforms often provide instant feedback, helping you identify mistakes and refine your approach.
- Video Tutorials: Step-by-step video explanations can break down complex concepts, showing you how to solve various problems. Watching these tutorials can clarify difficult topics and give you alternative strategies for tackling challenges.
- Practice Workbooks: Printable or digital workbooks are another great resource. These offer a wide variety of practice problems, often organized by difficulty, so you can gradually progress and test your skills in different areas.
- Study Groups: Collaborating with peers can be a valuable way to reinforce your learning. Joining a study group or working with a tutor allows you to share insights and tackle difficult problems together.
By utilizing these tools, you can build a more well-rounded understanding and increase your proficiency with different types of exercises. Consistent practice using these resources will help you gain confidence and improve your overall performance.
Benefits of Reviewing Section 1 Answers
Revisiting your solutions is an important practice that helps solidify your understanding and improve your performance. By carefully reviewing the steps and results of each problem, you can identify areas of weakness and strengthen your overall approach. This process not only ensures that you’ve completed each task correctly but also enhances your ability to solve similar problems in the future.
Here are some key benefits of revisiting your work:
- Improved Accuracy: Re-checking your solutions helps catch simple mistakes that may have been overlooked during the initial process. Ensuring your answers are accurate boosts your confidence and reduces the risk of errors in the future.
- Better Understanding: Reviewing your approach allows you to see if you fully understand the concepts behind each problem. This reflection can deepen your grasp of key ideas and help you apply them more effectively in different scenarios.
- Increased Retention: Going over your work again reinforces the information and strategies you’ve learned. Repetition is one of the best ways to commit knowledge to memory, making it easier to recall when needed.
- Identification of Patterns: When you review multiple problems, you begin to notice patterns or common strategies that can be applied to solve similar tasks. Recognizing these patterns can make future problem-solving more efficient.
By regularly reviewing your solutions, you not only ensure that your answers are correct, but you also cultivate a deeper understanding and enhance your ability to tackle future challenges with greater precision.
How to Use Algebra Nation Effectively
To make the most out of this learning platform, it’s important to approach it with a strategy that aligns with your goals. This tool offers a variety of exercises and resources designed to help you master key mathematical concepts. By using it efficiently, you can not only reinforce what you’ve learned but also challenge yourself to improve in areas where you may be struggling.
Here are some tips on how to use this platform effectively:
- Set Clear Goals: Before diving into the material, define what you want to achieve. Whether it’s mastering specific concepts, improving your speed, or boosting your overall understanding, having a clear focus will help you stay on track and motivated.
- Take Advantage of Practice Problems: Consistent practice is the key to improvement. Regularly solving problems on the platform will help you solidify your knowledge and increase your problem-solving speed. Start with easier tasks and gradually move to more complex ones as you progress.
- Use Available Resources: This platform provides more than just practice problems. Explore video lessons, tutorials, and hints for additional support. These resources can offer deeper insights into challenging topics and provide different perspectives on how to solve problems.
- Review Your Work: After completing each exercise, take time to go over your solutions and the reasoning behind them. If you made mistakes, understand where you went wrong and make adjustments for future problems. This process helps reinforce learning and improves your accuracy.
- Track Your Progress: Many platforms provide tracking tools that allow you to monitor your improvement over time. Use these tools to identify which areas need more focus and celebrate milestones as you continue to progress.
By following these steps, you can maximize the benefits of the platform and work more effectively towards mastering the content. Consistent use and a focused approach will help you build confidence and achieve better results in the long run.
Understanding Test Yourself Feedback
Feedback is an essential part of the learning process, offering insights into your performance and areas for improvement. When engaging with the platform, the feedback you receive after completing exercises can help identify strengths and highlight areas where you need more practice. Understanding how to interpret and act on this feedback is crucial for advancing your skills.
The feedback typically comes in the form of corrections, explanations, and guidance on how to improve. By reviewing this information carefully, you can better understand your mistakes, correct misconceptions, and apply more effective strategies in future exercises. Below is a breakdown of how to interpret common types of feedback you may encounter:
| Type of Feedback | Description | Action to Take |
|---|---|---|
| Correct Answer | Indicates that your solution was accurate and well-reasoned. | Review the steps to reinforce your understanding and replicate this approach in future problems. |
| Partial Credit | Shows that you made some progress but missed a key step or made a small mistake. | Identify the step you missed and rework the problem to avoid making the same error next time. |
| Incorrect Answer | Highlights a mistake or misunderstanding in your approach. | Revisit the concept involved, review tutorials or resources, and attempt similar problems to build confidence. |
| Hint Provided | Offers a suggestion or clue to help you work through the problem. | Consider the hint carefully and use it to adjust your strategy. Attempt the problem again with the new perspective. |
By consistently reviewing and acting on this feedback, you’ll improve your problem-solving skills and deepen your understanding of the material. Take the time to reflect on the suggestions provided and use them to refine your approach with each exercise.
Why Section 1 is Crucial for Success

The initial part of any learning process serves as the foundation for all subsequent knowledge. It is in this phase that you build the core skills necessary for tackling more complex problems. Mastering the basics is essential, as they provide the tools and understanding required for success in more advanced topics. Without a strong grasp of the fundamental concepts, progressing to more challenging material becomes much more difficult.
By focusing on the first set of exercises and concepts, you lay the groundwork for a deeper understanding of the subject. This phase is not only about memorizing facts but also about developing problem-solving strategies and critical thinking skills that will be used throughout your learning journey.
Moreover, this stage acts as a confidence booster. As you practice and refine your skills, your ability to approach more complex challenges improves. The initial success you experience here serves as motivation to continue progressing, reinforcing the importance of fully mastering this first phase before moving on.
Key Formulae for Algebra Section 1
In the initial stages of any mathematical journey, understanding and applying fundamental equations is crucial. These basic formulas serve as the building blocks for solving a wide range of problems. Mastering them provides the foundation for tackling more complex concepts and equations in later stages.
Below are some essential formulas that are frequently used in early exercises:
| Formula Name | Formula | Application |
|---|---|---|
| Linear Equation | y = mx + b | Used to represent straight lines, where m is the slope and b is the y-intercept. |
| Quadratic Equation | ax² + bx + c = 0 | Represents parabolic curves and is solved using factoring, completing the square, or the quadratic formula. |
| Slope Formula | m = (y2 – y1) / (x2 – x1) | Calculates the slope between two points on a coordinate plane. |
| Point-Slope Form | y – y1 = m(x – x1) | Useful for writing the equation of a line when a point and slope are known. |
These formulas are essential for working through initial problems, and understanding their application will aid in solving a variety of exercises efficiently. As you progress, these foundational formulas will continue to be valuable tools in more advanced topics.
How to Stay Motivated During Study
Staying focused and motivated during study sessions can be challenging, especially when faced with complex material or a lengthy study routine. However, developing effective strategies can significantly improve your persistence and concentration. By setting clear goals and breaking tasks into manageable segments, you can create a sense of accomplishment that fuels continued effort.
Here are some key techniques to help maintain motivation:
- Set Specific, Achievable Goals: Break down your study material into smaller, more manageable chunks. This makes it easier to track progress and gives you a sense of achievement with each completed task.
- Create a Positive Environment: Ensure your study space is organized, comfortable, and free from distractions. A clean and quiet environment fosters concentration and reduces stress.
- Reward Yourself: Set milestones for your study goals and treat yourself when you reach them. Whether it’s a short break, a snack, or an activity you enjoy, rewards can help you stay motivated.
- Stay Active: Incorporating physical activity into your routine can improve focus and energy. Short walks or stretching exercises can refresh your mind and reduce mental fatigue.
- Visualize Success: Picture the results of your hard work. Whether it’s mastering a challenging topic or achieving your long-term academic goals, keeping your end vision in mind can push you forward.
By applying these strategies, you can stay motivated and continue making progress in your studies, regardless of how difficult or tedious the material may seem. Consistency, paired with positive reinforcement, leads to lasting success in any learning journey.
Mastering Skills with Section 1
Building a solid foundation in mathematical problem-solving requires consistent practice and understanding of fundamental concepts. By focusing on essential techniques, learners can strengthen their abilities and tackle more complex problems with confidence. This section offers a focused approach to mastering key skills through targeted exercises and step-by-step guidance.
To effectively improve your problem-solving abilities, it’s important to approach each topic systematically. Here are some strategies to help you master the core skills:
- Understand Key Concepts: Before diving into problems, ensure you fully grasp the underlying concepts. This includes identifying key principles, formulas, and methods that will be applied in different scenarios.
- Practice Regularly: Consistent practice is essential to reinforce learning. Regularly working through exercises allows you to identify patterns, enhance recall, and improve accuracy.
- Work Step-by-Step: Break down each problem into smaller, manageable steps. This makes it easier to understand the process and helps to avoid mistakes.
- Use Available Resources: Take advantage of all available resources, including online tutorials, problem sets, and interactive tools, to deepen your understanding and refine your skills.
- Review Mistakes: After completing each exercise, take time to review any errors. Understanding why a particular approach didn’t work will help you avoid similar mistakes in the future and strengthen your reasoning skills.
By following these strategies and committing to regular practice, you can build mastery over time and approach challenges with a greater level of confidence. Success in mastering these skills is not about memorizing steps, but rather developing the ability to think critically and apply learned methods effectively.