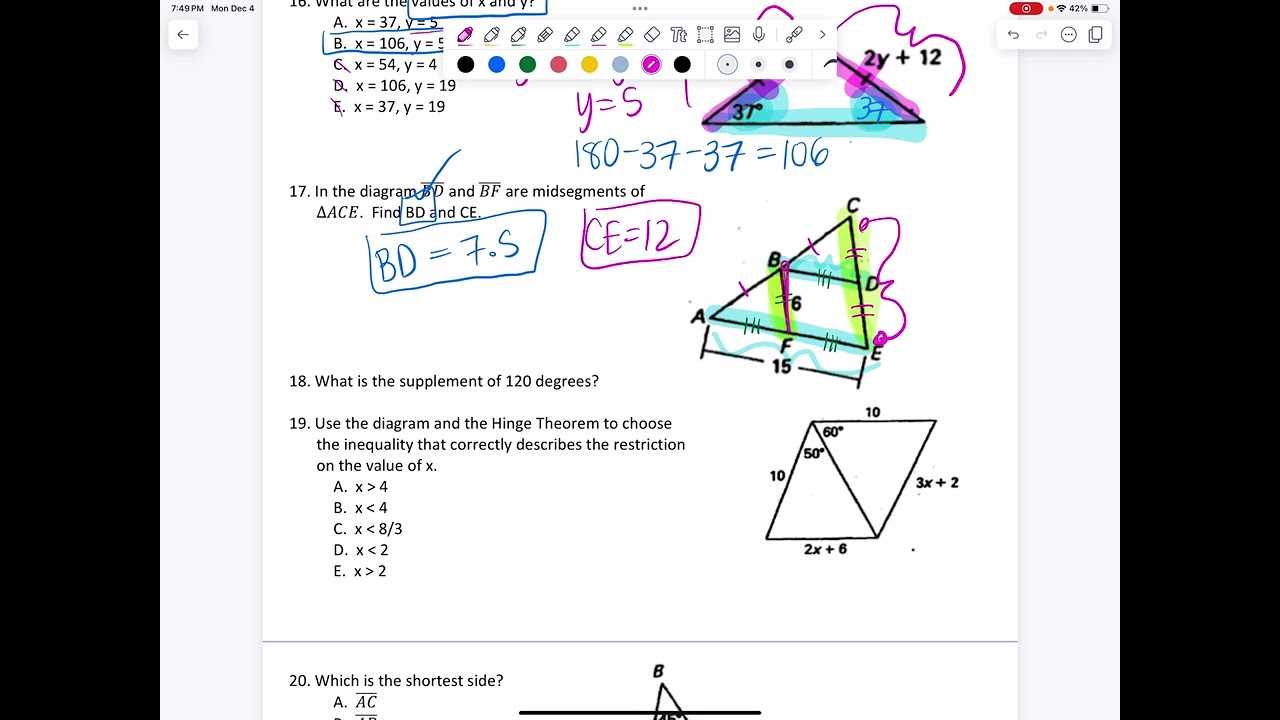
Preparing for a major test in mathematics can feel overwhelming, but breaking it down into manageable steps can make the process more approachable. This guide will help you focus on the most critical topics and give you the tools to tackle the toughest challenges. By understanding the key principles and practicing common types of problems, you’ll build the confidence needed to succeed.
Whether you’re reviewing shapes, angles, or algebraic concepts, each section provides insight into solving problems efficiently. Learning how to identify patterns, apply formulas, and check your work are vital skills that will serve you well during the assessment. This guide is designed to walk you through these essential ideas with clarity and practical examples, ensuring you’re ready for the challenge ahead.
Mathematical Concepts and Problem Solving Guide
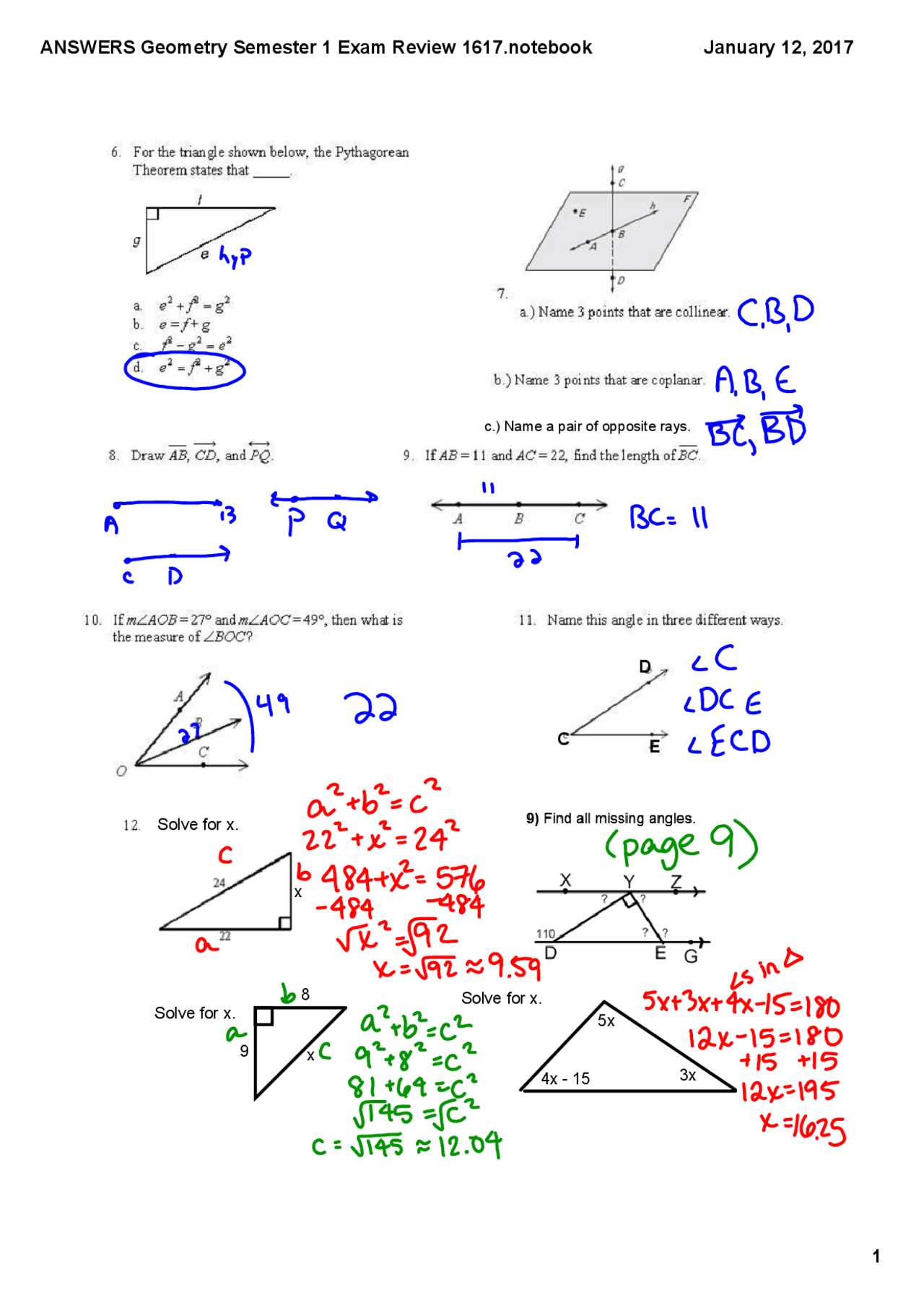
This section is designed to provide solutions and explanations for the most commonly tested problems, helping you understand the approach needed to achieve the correct results. By carefully reviewing the solutions, you’ll gain insight into the thought process behind each step, ensuring a deeper understanding of how to tackle similar challenges.
Identifying Key Patterns and Strategies
One of the most important aspects of solving problems is recognizing patterns and applying the right strategies. Whether you’re dealing with shapes, proportions, or equations, each problem has its unique approach. Practicing these patterns will help you quickly identify the method you need, making problem-solving more efficient during the test.
Step-by-Step Problem Breakdown
Breaking down each question into smaller, more manageable parts is essential for success. By focusing on one step at a time and cross-checking your results, you reduce the chances of making mistakes. This process allows for a clearer path to the solution, ensuring no detail is overlooked and enhancing your accuracy.
Overview of Semester 1 Geometry Topics
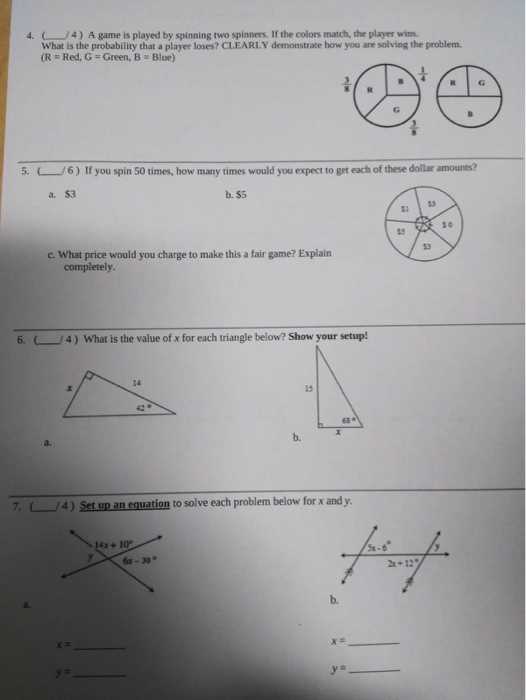
This section covers the core concepts and techniques that form the foundation of the material typically assessed in the first part of the academic year. Understanding these fundamental ideas is crucial for building the necessary skills to solve more complex problems. Topics include everything from shapes and their properties to the relationships between angles and measurements.
Among the key concepts, students will explore linear equations, congruence, similarity, and area and volume calculations. These areas require both analytical thinking and the ability to apply specific formulas to real-world scenarios. Mastery of these topics ensures a solid understanding of the basic structure of the subject.
Additionally, this section introduces the idea of logical reasoning and proof techniques, which are vital for progressing in advanced mathematical fields. Developing a clear approach to solving problems and recognizing patterns will greatly enhance performance and understanding.
Key Concepts to Focus On
To achieve success in the first portion of the course, it’s essential to concentrate on mastering the foundational principles that will support more advanced topics later. These concepts form the building blocks for all future problem-solving and ensure that you have the necessary tools to approach complex questions with confidence.
Understanding Shapes and Their Properties
One of the primary areas to focus on is the study of various shapes and their defining characteristics. This includes understanding the properties of triangles, quadrilaterals, and circles, as well as how to calculate area, perimeter, and other measurements. Recognizing these properties and how they relate to each other is key to solving related problems efficiently.
Angles, Lines, and Their Interactions
Another critical area is the relationship between angles, lines, and intersecting figures. Being able to identify different types of angles, such as complementary and supplementary, and understanding how they interact with parallel and perpendicular lines is essential. These principles often serve as the foundation for more complex geometric reasoning and proofs.
How to Use the Answer Key
Having access to solutions can be a valuable tool when preparing for assessments. However, it’s essential to approach the provided solutions thoughtfully. Instead of simply looking up the results, use them as a guide to understand the underlying methods and thought processes that led to the correct answers.
Step-by-Step Verification
Start by attempting the problem on your own before consulting the provided solutions. Once you’ve worked through the problem, compare your method with the solution to identify any discrepancies. Pay close attention to the reasoning behind each step, as this will help you understand how to approach similar challenges in the future.
Learn from Mistakes
If you find that your solution differs from the one provided, review the steps you took and pinpoint where you went wrong. Understanding your mistakes is crucial for improving your problem-solving skills. The goal is not just to reach the right answer but to fully grasp the reasoning behind each step.
Common Mathematical Mistakes to Avoid
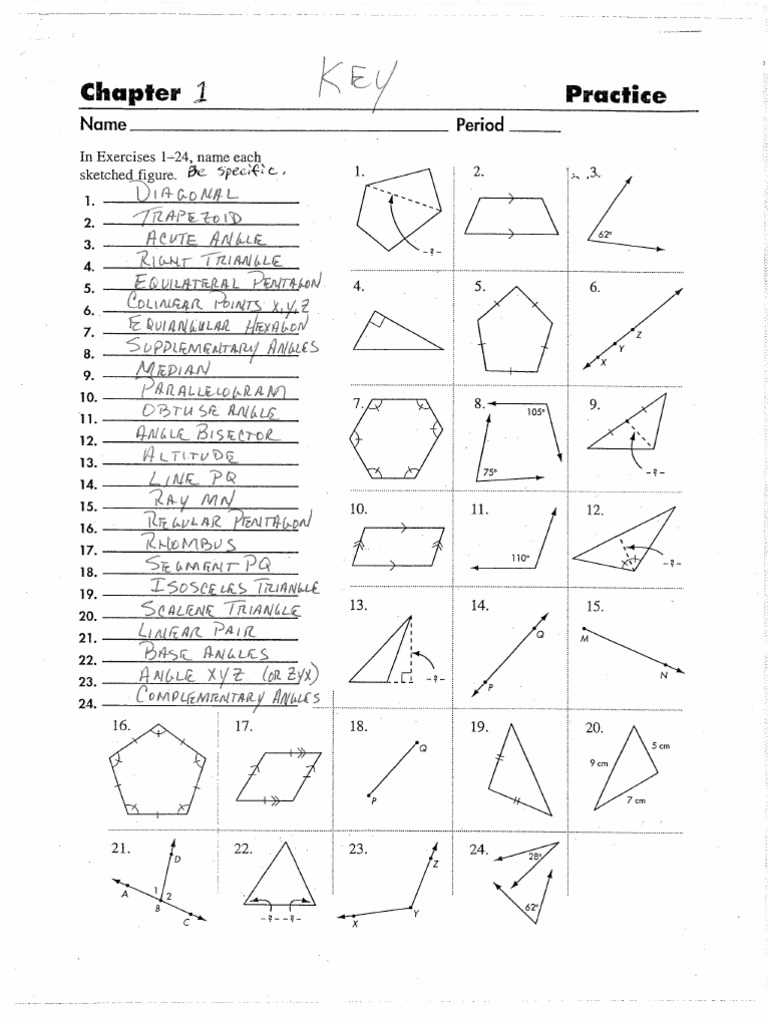
When working through mathematical problems, it’s easy to overlook small details that can lead to significant errors. Identifying and understanding these common mistakes can make a big difference in improving accuracy and confidence. Recognizing potential pitfalls ahead of time helps you approach each problem methodically and avoid unnecessary setbacks.
Misunderstanding the Relationship Between Angles
One frequent mistake is incorrectly interpreting the relationships between different types of angles, such as complementary or supplementary angles. Failing to identify parallel lines or the correct angle measurements can result in incorrect calculations and answers. Always double-check angle relationships to ensure proper application of rules.
Forgetting to Apply Units of Measurement
Another common error is neglecting to include or convert units of measurement, especially when calculating area, volume, or other dimensional properties. Always ensure that units are consistent throughout the problem and that you’re applying the correct conversion factors where necessary to avoid mismatched results.
Reviewing Basic Geometric Shapes
Understanding the fundamental shapes and their properties is essential for tackling a wide range of problems. These basic figures form the core of more complex tasks and are used in many different scenarios. Whether you’re working with polygons, circles, or other simple forms, recognizing their key characteristics and measurements will help you solve related problems more effectively.
Among the most important shapes to focus on are triangles, squares, rectangles, and circles. Each shape has specific rules for calculating area, perimeter, and other properties. Mastering these principles allows for a clear approach to problems that involve these figures, whether they appear alone or in combination with other shapes.
Important Theorems for the Exam
Understanding key principles and theorems is crucial for solving complex problems efficiently. These foundational concepts are widely applied across various types of problems and will help you make logical deductions in tests. Familiarizing yourself with the most important theorems ensures you’re prepared for any question that may arise during assessments.
Below are some of the core theorems that every student should review:
- Pythagorean Theorem: This theorem is essential for finding the length of sides in right-angled triangles. The relationship between the sides is expressed as a² + b² = c².
- Parallel Postulate: Understanding how parallel lines interact with transversal lines is key to solving problems involving angles and their relationships.
- Triangle Sum Theorem: The sum of the interior angles of any triangle is always 180°.
- Congruence Theorems (SSS, SAS, ASA): These theorems help determine when two triangles are congruent based on side and angle measurements.
- Circle Theorems: Various theorems involving the properties of tangents, chords, and arcs, which are essential for solving problems related to circular shapes.
By revisiting these theorems and understanding their applications, you’ll be better equipped to approach a wide range of problems with confidence and precision.
Understanding Angles and Their Properties
Angles play a vital role in a wide range of problems, from basic measurements to more complex proofs and constructions. A clear understanding of their types and how they interact is essential for solving many challenges. Recognizing the different classifications and relationships between angles enables more efficient problem-solving and application of relevant formulas.
Key concepts include identifying complementary, supplementary, and vertical angles, as well as understanding how angles relate to parallel lines and transversals. Mastering these fundamental ideas allows for easier identification of angle relationships, which are frequently used in both practical and theoretical scenarios.
Practice Problems for Success
Consistent practice is essential for mastering any subject. Solving a variety of problems helps reinforce concepts and improves your ability to tackle different types of challenges. By working through problems that test your understanding, you build confidence and become more efficient in applying mathematical principles.
Basic Shape and Measurement Problems
Start with simple problems that involve calculating areas, perimeters, and other measurements for common shapes like rectangles, triangles, and circles. These types of questions are fundamental and provide a solid foundation for more complex tasks.
| Problem | Solution |
|---|---|
| Find the area of a rectangle with length 8 cm and width 5 cm. | Area = length × width = 8 × 5 = 40 cm² |
| What is the circumference of a circle with radius 7 cm? | Circumference = 2πr = 2 × π × 7 ≈ 43.98 cm |
Angle and Line Relationship Problems
Next, focus on problems that involve angle relationships, such as complementary and supplementary angles, or those involving parallel lines and transversals. These problems help you understand the connections between angles and how to apply the correct rules.
| Problem | Solution |
|---|---|
| If two angles are complementary and one measures 30°, what is the other angle? | Complementary angles sum to 90°. Therefore, 90° – 30° = 60°. |
| Two parallel lines are cut by a transversal. One angle measures 40°. What is the measure of the alternate interior angle? | Alternate interior angles are congruent. Therefore, the alternate interior angle also measures 40°. |
Tips for Solving Word Problems
Word problems can often seem intimidating due to their complex scenarios and lengthy descriptions. However, breaking down the problem into smaller, more manageable parts can make it easier to understand and solve. By carefully analyzing the information provided and following a structured approach, you can find the solution step by step.
Start by reading the problem carefully, identifying the key information, and noting down the question being asked. Highlight the numbers, relationships, and any conditions mentioned. Once you have a clear understanding of the given data, translate it into mathematical terms and proceed with the appropriate operations.
Here are some helpful strategies for solving word problems:
- Identify the question: Focus on what the problem is asking and determine the unknown values.
- Extract important information: Pull out the relevant details from the text, such as measurements, relationships, and constraints.
- Draw diagrams: Whenever possible, create a visual representation of the problem to make the relationships between elements clearer.
- Choose the right formula: Match the problem to the appropriate formula or equation that will help you solve for the unknown.
- Check your work: After calculating the answer, review the problem to ensure that your solution makes sense and fits the context.
By practicing these techniques, you can improve your problem-solving skills and approach word problems with greater confidence and efficiency.
How to Approach Proof Questions
Proof questions require a logical and structured approach to demonstrate the validity of a statement based on given information. The key to solving these problems lies in organizing your thoughts clearly, building from established facts, and following a step-by-step process to arrive at the conclusion. While the process can be challenging, breaking it down into manageable steps ensures clarity and accuracy in your reasoning.
Follow these steps to effectively tackle proof-based problems:
- Understand the statement: Carefully read the problem and identify what is being asked to prove. Highlight key terms and any given information.
- Identify known facts: List out any known properties, theorems, or postulates that might help in the proof. These will be the building blocks of your reasoning.
- Plan your approach: Think about how to connect the given information to the statement you need to prove. Consider using direct proof, indirect proof, or proof by contradiction, depending on the situation.
- Write out the proof step-by-step: Start from the known facts and work logically through the problem. Ensure that each step follows logically from the previous one and is supported by a theorem, definition, or postulate.
- Conclude the proof: After reaching the desired conclusion, summarize the proof to show that you have demonstrated the statement is true based on the given information and logical reasoning.
With practice, approaching proof questions will become a more natural and systematic process, leading to clearer and more accurate solutions.
Time Management for Assessments
Effective time management is crucial for performing well in any assessment. Knowing how to allocate your time across different sections of the test can make a significant difference in your ability to answer all questions thoughtfully and thoroughly. Without a clear plan, you may end up rushing through some parts while spending too much time on others.
To avoid time-related stress and optimize performance, it is important to prioritize tasks, maintain a steady pace, and stay organized throughout the assessment. Below is a table with tips on managing your time effectively during a test:
| Time Management Tip | Details |
|---|---|
| Understand the test format | Before starting, quickly review the sections to understand their weight and structure. This helps you allocate time appropriately. |
| Set time limits per section | Divide the total test time by the number of sections to set time limits. Stick to these to avoid spending too much time on any one part. |
| Start with easy questions | Begin with the questions you are most confident in to build momentum and save time for more complex tasks later. |
| Don’t linger on tough problems | If you get stuck, move on to the next question and return later. This ensures you make progress and don’t lose valuable time. |
| Leave time to review | Always reserve a few minutes at the end to review your answers, checking for any mistakes or missed details. |
By following these strategies, you can manage your time efficiently, reduce stress, and improve your performance during any assessment.
How Geometry Relates to Real Life
Understanding the principles of shapes, sizes, and spatial relationships extends beyond academic exercises and plays a crucial role in daily life. From architecture to technology, these concepts are applied in countless ways to solve real-world problems. Recognizing how these ideas connect to the world around us helps to appreciate their significance and practical value.
Here are a few examples of how these principles influence our daily experiences:
- Architecture and Construction: The design of buildings, bridges, and other structures relies heavily on concepts like angles, symmetry, and measurements to ensure safety, stability, and aesthetic appeal.
- Art and Design: Artists use these principles to create visually pleasing compositions, understanding balance, proportion, and perspective to achieve the desired effect.
- Technology and Engineering: In fields like robotics, computer graphics, and product design, spatial reasoning helps to develop and refine products and software.
- Navigation and Mapping: Travel and navigation tools, such as GPS, use geometric concepts to accurately represent locations, distances, and directions.
- Everyday Tasks: Whether it’s planning a garden, designing a room layout, or crafting a DIY project, understanding spatial relationships is key to making informed decisions and achieving desired results.
By recognizing how these abstract concepts are integrated into practical applications, we can better appreciate their importance and improve our problem-solving abilities in real-world scenarios.
Strategies for Memorizing Key Formulas
Memorizing essential equations and relationships is an important part of mastering concepts in any subject. While it may seem challenging at first, using effective strategies can make the process more manageable and help you retain the information for longer periods. Understanding how to memorize and recall formulas with ease is crucial for performing well in assessments and applying knowledge practically.
Effective Memorization Techniques
Here are some proven methods for retaining key equations:
- Use Mnemonics: Create memorable phrases or acronyms to associate with each formula. This mental shortcut helps you recall complex information quickly.
- Practice Regularly: Repetition is a powerful tool for memorization. Writing formulas down multiple times or testing yourself consistently can help reinforce memory.
- Understand the Formula: Rather than simply memorizing the formula, take the time to understand what each component represents and how it is derived. This deeper understanding can make recall easier.
- Visual Aids: Create visual representations of formulas, such as diagrams or charts, that connect the formula to real-world examples. This approach makes abstract ideas more tangible.
- Flashcards: Use flashcards to quiz yourself on key formulas. This method allows for active recall and reinforces your memory.
Organizing Formulas for Easy Access
To optimize your study process, it’s also helpful to organize your formulas systematically:
- Group Similar Formulas: Organize equations based on topics or categories (e.g., area, volume, angles). This creates a logical flow that aids in recalling related formulas together.
- Create a Formula Sheet: Having a dedicated sheet with key formulas can be useful for quick reference during practice sessions. Regularly review this sheet to reinforce your memory.
- Use Apps or Digital Tools: Digital tools and apps offer interactive ways to quiz yourself on formulas, track your progress, and reinforce your learning.
By combining these techniques, you can improve your ability to recall and apply important equations with ease, making the learning process more efficient and less stressful.
Common Pitfalls in Geometry Exams
When tackling assessments in this subject, there are several common mistakes that many students make. These errors often arise from a lack of attention to detail or misunderstanding the core principles. Recognizing these pitfalls in advance can help you avoid them and improve your performance. In this section, we will explore some of the most frequent challenges and how to overcome them.
Misinterpreting the Problem
One of the most common issues students face is misinterpreting the question. This can lead to unnecessary confusion and result in incorrect solutions. Here are some key things to keep in mind:
- Read Carefully: Ensure you fully understand the wording of the problem before starting. Look for important details such as specific measurements or conditions.
- Identify What’s Being Asked: Often, problems contain extra information that isn’t necessary for solving the problem. Focus on what’s being asked and what information is required.
- Check Units: Pay attention to units of measurement, as using the wrong ones can lead to errors in calculations.
Skipping Steps or Rushing Through the Process
Many students tend to skip steps in the problem-solving process, especially when they feel confident or are running out of time. However, this can lead to incomplete or incorrect solutions. Here’s how to avoid rushing:
- Show Your Work: Always write down the steps you take, even if you can do some calculations mentally. This helps you avoid mistakes and allows you to check your reasoning.
- Take Your Time: While it’s important to manage your time, rushing through problems increases the risk of making careless mistakes. Ensure you give yourself enough time for each section.
- Use Process of Elimination: If you are unsure about a step, try eliminating impossible answers or options. This strategy can help guide your decision-making.
By being aware of these common pitfalls and adopting strategies to address them, you can approach each task with greater confidence and precision. Proper preparation and a methodical approach will significantly reduce the likelihood of these errors affecting your performance.
How to Stay Calm During the Test
Test anxiety is a common challenge that can affect performance, even for well-prepared students. The pressure to perform well can lead to stress, which in turn can impair focus and memory recall. In this section, we will explore effective strategies to maintain composure during a high-pressure assessment and ensure that you approach each question with clarity and confidence.
Practice Deep Breathing
One of the most effective ways to calm your nerves is through deep breathing exercises. Taking a few slow, deep breaths can reduce stress and help you focus on the task at hand. When you feel anxiety building:
- Inhale deeply for a count of four, hold for four seconds, and exhale for a count of four. Repeat this process several times to reduce tension and clear your mind.
- Focus on your breathing: This simple technique will help shift your attention away from any distractions or feelings of panic.
Break the Test Into Manageable Parts
Instead of viewing the entire assessment as one overwhelming task, break it down into smaller, more manageable sections. Tackling smaller pieces one at a time can make the test seem less intimidating:
- Start with familiar material: Begin with the questions you feel most confident about to build momentum and boost your confidence.
- Move on to more challenging questions: Once you’ve completed the easier sections, focus on the harder ones. Don’t dwell too long on any one question if you get stuck–mark it and move on.
Maintain a Positive Mindset
A positive attitude can significantly impact your performance. Even if a question seems difficult or confusing, remind yourself that you have prepared for this moment. Use affirmations like:
- “I am capable of solving this problem.” Repeating positive statements can help reduce negative thoughts and enhance your focus.
- Visualize success: Imagine yourself completing the test calmly and confidently. Visualization is a powerful tool for reducing anxiety and building self-assurance.
By adopting these strategies, you can reduce stress and maintain a calm, focused mindset throughout the assessment. Remember, staying calm allows your brain to work more effectively, helping you to think clearly and perform at your best.