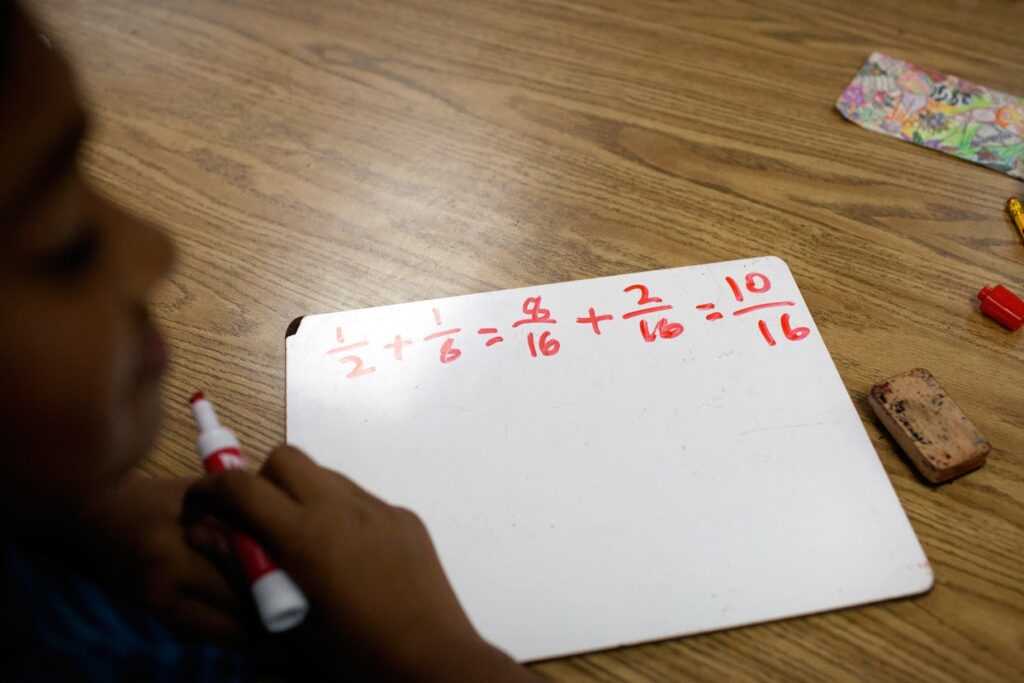
Mathematics can be a challenging subject, but mastering its core concepts is essential for building a strong foundation. Whether you’re struggling with basic calculations or complex equations, understanding the principles behind each problem is the first step toward success. This section provides clear solutions and strategies to help you tackle various mathematical topics effectively.
By breaking down problems into manageable steps, you’ll gain confidence in solving equations, working with functions, and applying mathematical concepts to real-world scenarios. The goal is to provide the tools and methods necessary to navigate through assignments, tests, and practical applications with ease. With regular practice, anyone can improve their math skills and achieve mastery.
Developing problem-solving techniques and learning to recognize patterns are crucial skills that will serve you well not only in the classroom but also in everyday life. As you progress, remember that understanding the “why” behind each solution is just as important as reaching the correct answer. This guide will help you build both your reasoning and computation skills.
Big Ideas Math Integrated Mathematics 1 Answers
In any learning process, understanding key principles and applying them to different types of problems is crucial for mastery. This section is designed to help students navigate through various topics by providing clear explanations and step-by-step methods. By focusing on the logic behind each solution, learners can enhance their comprehension and boost problem-solving skills.
To effectively tackle exercises and assignments, it’s important to follow a structured approach. Here are some strategies to help simplify complex tasks:
- Break down the problem into smaller, more manageable parts.
- Identify the essential concepts and focus on understanding their applications.
- Practice similar problems to reinforce your skills and gain confidence.
- Check your work regularly to ensure accuracy and correct any mistakes along the way.
Mastering the topics in this section will provide students with a deeper understanding of essential concepts, including:
- Solving algebraic equations
- Graphing functions and interpreting data
- Working with geometric shapes and measurements
- Analyzing word problems and applying mathematical reasoning
Each exercise will be accompanied by clear, detailed steps to guide learners through the process, ensuring that no concept is left unexplained. By practicing consistently and following the outlined strategies, students will find themselves more equipped to solve a wide range of problems confidently and accurately.
Understanding the Key Concepts

Grasping the foundational principles behind each topic is essential for success in any subject. When working through various mathematical challenges, it is important to first understand the key ideas that form the basis for solving problems. These concepts are not only building blocks for more advanced topics but also tools for applying knowledge to practical situations.
Breaking Down Complex Topics
Each mathematical concept is a piece of a larger puzzle. It is essential to understand how smaller principles connect to create a complete picture. By approaching problems step by step, learners can identify patterns and relationships between concepts, making it easier to tackle more complex problems. A solid understanding of these core topics ensures a deeper grasp of the subject and enhances problem-solving ability.
Real-World Applications
Once the foundational principles are understood, the next step is to recognize their real-world applications. Many mathematical concepts are used to solve problems in daily life, from budgeting and measurements to analyzing data. Understanding how to apply these concepts in practical scenarios not only strengthens theoretical knowledge but also provides motivation and context for learning.
Step-by-Step Solutions for Problems
Breaking down complex problems into manageable steps is a powerful method for achieving clarity and accuracy. By approaching each challenge systematically, students can better understand the reasoning behind each solution and build confidence in their abilities. This process also allows for careful checking of each step, reducing errors and improving overall problem-solving skills.
Here is a general approach to solving problems:
- Identify the problem: Read the problem carefully and determine what is being asked. Highlight key information and organize it.
- Plan the solution: Choose an appropriate method or strategy to solve the problem. This may involve using formulas, equations, or logical reasoning.
- Perform the steps: Work through the calculations or steps methodically, ensuring that each part is understood before moving on.
- Review the solution: Double-check each step to ensure there are no mistakes, and verify the final answer makes sense in the context of the problem.
By following these steps, students can approach any problem with a clear plan and improve their ability to solve challenges with accuracy and confidence. Regular practice with this method helps reinforce key concepts and enhances problem-solving skills across different topics.
Tips for Solving Algebraic Equations
Solving algebraic equations involves finding the value of unknown variables that make the equation true. Understanding how to manipulate and simplify expressions is key to solving problems efficiently. By following a structured approach and applying specific strategies, anyone can enhance their ability to work through algebraic challenges with confidence.
Isolate the Variable
The first step in solving an equation is to isolate the variable on one side of the equation. This can be done by performing inverse operations, such as addition or subtraction, to eliminate constants or coefficients from one side. Once the variable is isolated, the equation becomes simpler to solve.
Balance Both Sides
It is crucial to maintain balance in the equation by performing the same operation on both sides. Whether you’re adding, subtracting, multiplying, or dividing, always ensure that whatever change you make to one side is also made to the other. This principle of equality helps preserve the integrity of the equation and ensures a correct solution.
How to Tackle Word Problems Effectively
Word problems can often seem overwhelming due to the need to interpret a narrative and convert it into mathematical expressions. However, with the right approach, they become much more manageable. The key is to break down the problem, understand what is being asked, and identify the necessary steps to reach a solution. By following a structured method, you can make sense of even the most complex problems.
The first step is to carefully read the problem and highlight important information such as quantities, relationships, and keywords that indicate specific mathematical operations. Next, translate this information into an equation or set of equations that represent the situation. Finally, solve the equation and interpret the solution in the context of the original problem.
Practicing word problems regularly improves your ability to quickly recognize patterns and apply the correct strategies. By focusing on the logic behind the problem and staying organized, you can confidently approach and solve word problems with ease.
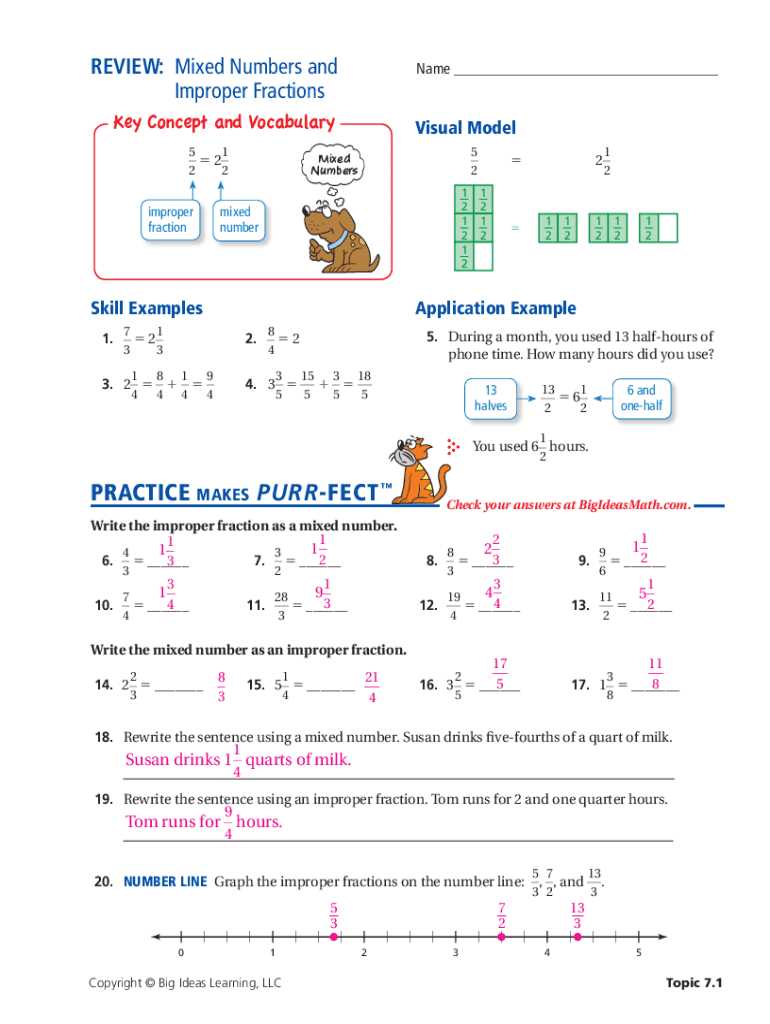
Mastering Fractions and Decimals
Understanding fractions and decimals is essential for building a strong foundation in numerical problem-solving. These two forms of numbers are used in a wide range of applications, from basic arithmetic to complex calculations. By mastering how to work with them, you’ll gain the ability to handle diverse problems with confidence and accuracy.
The key to mastering fractions is understanding the relationship between the numerator and denominator. Practice simplifying fractions, converting between fractions and mixed numbers, and finding common denominators to make operations easier. With decimals, focus on converting between fractions and decimal form, as well as performing addition, subtraction, multiplication, and division with decimal numbers.
Converting between fractions and decimals is a skill that opens the door to various calculations. When converting a fraction to a decimal, simply divide the numerator by the denominator. For decimals, it’s helpful to understand how to move the decimal point to convert between forms or round to the desired precision.
Consistent practice and understanding of these concepts are vital for building fluency. Once you can confidently work with both fractions and decimals, you’ll be able to apply them seamlessly in a variety of mathematical contexts.
Geometry Basics in Integrated Mathematics
Geometry is the study of shapes, sizes, and the properties of space. It plays a crucial role in understanding the physical world and is essential for solving many real-world problems. By mastering the fundamental concepts of geometry, students can develop the skills necessary to tackle more advanced topics and apply them effectively in various fields.
One of the first concepts to understand is the relationship between points, lines, angles, and planes. These basic elements form the foundation of more complex geometric structures. Understanding how to measure and work with different shapes, such as triangles, quadrilaterals, and circles, is essential for solving geometric problems.
Additionally, mastering geometric principles like the Pythagorean theorem, the properties of parallel and perpendicular lines, and calculating the area and volume of different figures will greatly enhance problem-solving abilities. By applying these techniques step by step, students can develop a solid understanding of geometry that will support their learning in other areas of mathematics and beyond.
Important Formulas to Remember
Formulas are essential tools in solving a wide range of mathematical problems. By memorizing key equations, students can approach challenges more efficiently and effectively. Whether you’re working with basic operations or more complex concepts, knowing the right formulas is crucial for finding quick and accurate solutions.
Some of the most important formulas to keep in mind include those for calculating areas, volumes, and basic algebraic equations. These formulas provide the foundation for solving problems in various topics such as geometry, algebra, and statistics.
For example, the formula for the area of a rectangle A = l × w (where l is the length and w is the width) or the Pythagorean theorem a² + b² = c² for right-angled triangles are fundamental. Similarly, knowing how to calculate the volume of a cylinder V = πr²h or the area of a circle A = πr² can help solve problems related to space and measurement.
By regularly practicing and applying these formulas, students will improve their ability to solve problems across different areas with confidence and ease.
Common Mistakes and How to Avoid Them
In any field of study, errors are part of the learning process. However, some mistakes are more common than others and can be avoided with careful attention and practice. By recognizing these pitfalls early and understanding how to correct them, students can improve their skills and approach problems with greater accuracy.
One of the most frequent mistakes is overlooking key details in word problems. It’s easy to misinterpret what’s being asked or skip over important information. To avoid this, always read the problem thoroughly and highlight the key elements before attempting to solve it.
Another common error is misapplying formulas or mathematical operations. For example, when working with fractions, students often forget to find a common denominator before adding or subtracting. To prevent such mistakes, review the steps for each operation and practice regularly to reinforce understanding.
Lastly, be mindful of signs in algebraic expressions. It’s easy to forget to distribute negative signs or reverse them when solving equations. Double-check your work, especially when working with multiple terms, to ensure all signs are correctly handled.
By recognizing these common mistakes and applying strategies to avoid them, students can greatly improve their problem-solving accuracy and efficiency.
Using Online Resources for Extra Help
In today’s digital age, online resources provide an excellent way to reinforce learning and gain additional support. Whether you’re struggling with a specific topic or looking to review key concepts, the internet offers a wealth of tools and platforms to help students succeed. From video tutorials to interactive exercises, online resources can make complex concepts easier to understand.
Websites like educational platforms, forums, and tutorial videos can break down challenging topics into smaller, more manageable parts. Many of these resources provide step-by-step explanations, examples, and even quizzes to test your understanding. Some sites also offer live tutoring sessions or discussion boards where you can ask questions and get feedback from experts or fellow learners.
In addition to websites, there are apps and software designed specifically for solving problems and practicing different concepts. These tools often include visual aids like graphs or dynamic simulations, which can help visualize abstract ideas and improve problem-solving skills.
By utilizing these online tools, students can get the extra practice they need and access different teaching methods that may help them grasp difficult concepts more effectively.
How to Prepare for Tests
Preparing effectively for an exam requires more than just reviewing notes; it involves a strategic approach that helps reinforce understanding and boost confidence. By organizing study time, practicing regularly, and focusing on key concepts, students can approach assessments with a clear and calm mindset. Below are several strategies to help you prepare thoroughly and perform well on tests.
One of the most effective ways to study is by breaking down the material into manageable sections. Focus on mastering one concept at a time, and ensure you understand it before moving on. Regular practice and problem-solving are key, as they help solidify your grasp of the material and highlight areas where you might need more review.
Here are some tips to help guide your preparation:
| Preparation Tips | Explanation |
|---|---|
| Start Early | Give yourself plenty of time to review all topics, so you aren’t overwhelmed as the test date approaches. |
| Practice Regularly | Consistent practice helps reinforce concepts and exposes you to a variety of problems. |
| Work on Weak Areas | Identify the topics where you struggle the most and focus additional time on them. |
| Use Study Resources | Leverage textbooks, online platforms, and practice tests to further enhance your understanding. |
| Get Enough Sleep | A well-rested mind retains information better and performs more efficiently under stress. |
By implementing these strategies, you can create a comprehensive study plan that prepares you to tackle any test confidently. Stay focused, and remember that consistent effort leads to success!
Benefits of Practicing Math Regularly
Consistent practice is one of the most powerful ways to strengthen problem-solving skills and improve overall understanding of concepts. By dedicating time each day to solving problems and reviewing key topics, students can build confidence and master difficult subjects. Regular practice not only enhances performance but also provides lasting benefits that extend beyond academics.
Here are some of the key advantages of practicing frequently:
- Improved Retention: Regular practice helps reinforce what you’ve learned, making it easier to remember and apply concepts when needed.
- Faster Problem Solving: The more you practice, the quicker and more efficiently you can solve problems, even under time pressure.
- Increased Confidence: As you become more familiar with various types of problems, your self-assurance grows, reducing anxiety during tests.
- Stronger Analytical Skills: Practice develops your ability to break down complex problems and think critically about solutions.
- Better Exam Performance: Regular engagement with exercises leads to improved exam results as it helps identify gaps in knowledge and refine techniques.
By incorporating practice into your daily routine, you’ll not only excel in exams but also cultivate lifelong skills that will serve you well in various aspects of life. Consistency is the key to mastering any subject and achieving long-term success.
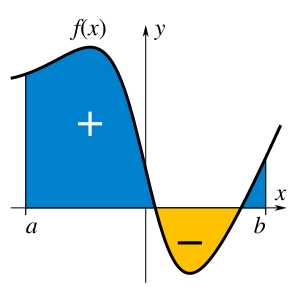
Understanding Functions and Graphs
Grasping the relationship between equations and their graphical representations is crucial for solving problems in various areas. Functions allow us to model real-world situations and interpret patterns by expressing relationships between variables. Understanding how to manipulate these relationships and visualize them through graphs is an essential skill in problem-solving and analysis.
When working with functions and graphs, it’s important to recognize the key features of a graph and how they correspond to the function’s behavior. Below are some important concepts to keep in mind:
- Domain and Range: The domain refers to the set of possible input values, while the range represents the output values. These define the scope of a function.
- Intercepts: The points where the graph intersects the x-axis (x-intercepts) and y-axis (y-intercepts) provide critical information about the function.
- Slope: The slope indicates the steepness of the graph. It is determined by the rate of change of the function, often described as “rise over run.”
- Behavior at Infinity: Understanding how a graph behaves as it moves towards positive or negative infinity is important for analyzing limits and trends.
- Key Functions: Familiarizing yourself with basic functions, such as linear, quadratic, and exponential, will allow you to recognize and interpret their graphs more easily.
By analyzing the graph of a function, you can gain insights into its behavior, predict future values, and solve real-world problems. Practicing the plotting of various types of functions will sharpen your ability to recognize patterns and improve your understanding of complex concepts.
Strategies for Improving Problem-Solving Skills
Enhancing your ability to solve complex problems involves more than just understanding the concepts–it requires developing a strategic approach that encourages critical thinking and persistence. By incorporating effective techniques into your study routine, you can tackle challenges with greater confidence and efficiency. Improving problem-solving skills is a gradual process that benefits from consistent practice and a focus on key strategies.
Here are some strategies to help strengthen your problem-solving abilities:
- Understand the Problem: Before jumping into a solution, take time to read the problem carefully. Make sure you understand what is being asked and identify the known and unknown variables.
- Break It Down: Complex problems often contain smaller, manageable parts. Divide the problem into steps and solve each one individually to avoid feeling overwhelmed.
- Visualize the Situation: Drawing diagrams, charts, or graphs can help you see relationships more clearly. Visual representations make it easier to identify patterns and understand the structure of the problem.
- Choose the Right Approach: Evaluate different methods for solving the problem. Whether it’s working backwards, using algebraic manipulation, or applying a known formula, choosing the right approach is crucial.
- Practice Regularly: The more you practice, the better you’ll become at recognizing common problem types and applying appropriate strategies. Repeated practice builds intuition and sharpens your problem-solving skills.
- Learn from Mistakes: Don’t be discouraged by errors. Mistakes are valuable learning opportunities. Review where you went wrong and adjust your approach for next time.
- Stay Organized: Keep your work neat and orderly. Proper organization helps you track your progress and ensures that no steps are missed in the problem-solving process.
By applying these strategies, you will not only improve your problem-solving skills but also develop a more systematic approach to challenges. Over time, these techniques will become second nature, allowing you to tackle even the most complex tasks with ease.
Real-Life Applications of Integrated Math
Understanding how mathematical concepts apply to real-world situations is essential for students. The skills learned in problem-solving, calculations, and logical thinking are not just academic; they have practical uses in everyday life. These concepts can help solve real-life problems across various fields, making them valuable tools for both personal and professional scenarios.
From managing personal finances to designing structures, the practical application of mathematical knowledge is everywhere. In this section, we explore some common areas where these principles are used effectively.
Personal Finance and Budgeting
One of the most direct applications of mathematical concepts is in personal finance. From budgeting to saving and investing, the ability to understand and manipulate numbers is crucial for making informed financial decisions. Mathematical skills help individuals plan their expenses, track spending, and calculate interest rates for loans or savings accounts.
Career and Professional Fields
Many professions require a solid understanding of mathematical principles. Engineers, architects, scientists, and even digital marketers rely on various forms of calculation and problem-solving to optimize their work. Whether designing a bridge or analyzing data trends, these professionals apply mathematical techniques to create solutions in their respective fields.
| Profession | Mathematical Application |
|---|---|
| Engineer | Design and structural analysis, materials calculations |
| Architect | Blueprint design, measurements, structural integrity |
| Scientist | Data analysis, experimentation, model building |
| Digital Marketer | Data analysis, calculating ROI, trend forecasting |
By applying mathematical concepts in real-life contexts, individuals not only enhance their problem-solving abilities but also gain the confidence to tackle practical challenges. These applications reinforce the relevance of mathematical learning and its direct connection to the world around us.
Tools and Apps to Assist Learning
In today’s digital age, numerous tools and applications can support learners in understanding complex concepts and improving their problem-solving abilities. These resources are designed to provide interactive exercises, tutorials, and instant feedback, allowing students to reinforce their skills and track their progress. By using the right tools, students can enhance their learning experience and better prepare for exams and assignments.
Below are some useful tools and apps that can assist with understanding various mathematical concepts and improving overall academic performance:
- Wolfram Alpha: A computational engine that provides step-by-step solutions to a wide range of problems, from algebra to advanced calculus. It’s particularly helpful for visualizing functions and solving equations.
- GeoGebra: An interactive platform for learning and teaching mathematics. GeoGebra is especially useful for geometry, algebra, and calculus, allowing users to create graphs and visual representations of mathematical concepts.
- Khan Academy: A free online learning resource offering lessons in a wide variety of subjects. The site features video tutorials, exercises, and quizzes that can help reinforce key mathematical concepts.
- Photomath: An app that allows students to take pictures of handwritten or printed math problems and provides step-by-step solutions. It’s perfect for practicing and understanding specific problems.
- Desmos: An advanced graphing calculator that helps students visualize equations and data. It’s widely used for plotting graphs and analyzing functions.
These apps and tools can provide students with an interactive and flexible way to engage with their studies. By incorporating these resources into their routine, learners can develop a deeper understanding of the subject matter and improve their performance.
Reviewing Key Lessons Before Exams
Effective review before exams plays a crucial role in reinforcing important concepts and boosting confidence. By revisiting core topics, students can identify areas of weakness, refine their problem-solving strategies, and ensure they are fully prepared for any challenge. A structured approach to review allows for better retention and understanding, ultimately leading to improved performance during exams.
Steps to Review Key Lessons Effectively
- Organize Study Sessions: Break down the material into manageable sections and allocate time for each topic. Use a study schedule to ensure all key concepts are covered.
- Focus on Weak Areas: Identify areas where you struggle and spend extra time reviewing those sections. Practice problems and seek clarification on difficult concepts.
- Use Practice Tests: Simulate exam conditions by taking practice tests. This helps familiarize you with the exam format and allows you to assess your understanding.
- Review Past Mistakes: Go over any previous assignments or quizzes where mistakes were made. Understanding why you made those errors will help avoid repeating them.
Additional Tips for Efficient Review
- Summarize Key Concepts: Write down concise summaries of each topic, highlighting the most important formulas, theorems, or procedures.
- Teach Someone Else: Teaching a concept to someone else can reinforce your own understanding. Explain key ideas aloud to a study partner or even to yourself.
- Stay Positive and Relaxed: Keep a positive attitude and stay calm. Stress can impair your ability to retain information, so ensure you have adequate rest and take regular breaks during your review sessions.
By following these strategies, students can maximize their study sessions and approach their exams with a clear and confident mindset. Regular review of the material helps to consolidate knowledge, ultimately leading to better exam results.