Understanding the core principles of your studies is essential for excelling in any subject. For students aiming to strengthen their skills, it is important to focus on both theoretical knowledge and practical problem-solving techniques. Working through problems with accurate solutions can significantly enhance comprehension and confidence.
By exploring the right resources, learners can find valuable tools that guide them step-by-step, helping to clarify doubts and correct errors. Practicing with these resources encourages deeper learning and prepares students for both exams and real-world applications.
Utilizing these helpful references can make the difference between struggling with concepts and achieving mastery. With the right approach, students can build a strong foundation that will serve them well in future challenges. Success in solving problems lies in consistent practice, understanding patterns, and learning from mistakes. Embrace the process, and your skills will naturally improve over time.
Understanding Grade 10 Concepts
Grasping the foundational ideas behind your studies is crucial for academic success. When working through problems, it’s not just about finding the right solution, but also understanding the methods and reasoning that lead to that solution. This deeper understanding helps to improve problem-solving abilities and builds the confidence needed for tackling more complex challenges.
Key Topics in the Curriculum
The curriculum for this level is designed to challenge students, pushing them to apply learned concepts in new and practical ways. Topics covered range from simple arithmetic to more advanced ideas like algebra and geometry. Understanding the interconnections between these subjects is vital for gaining proficiency and solving problems effectively.
Approaching Complex Problems
Breaking down difficult tasks into smaller, manageable steps can make complex problems easier to solve. Recognizing patterns, identifying what is given and what is asked, and using previous knowledge all contribute to finding the best approach. Practicing these techniques ensures smoother progression through challenging topics.
| Topic | Key Concepts | Common Challenges |
|---|---|---|
| Algebra | Solving equations, simplifying expressions | Variable manipulation, understanding functions |
| Geometry | Angles, shapes, areas | Visualizing properties, applying formulas |
| Statistics | Data analysis, probability | Interpreting graphs, calculating averages |
By gaining a solid grasp of these subjects, students can improve their skills and approach new problems with greater ease. Mastery of core concepts will help build a strong foundation for future learning and exams.
Why Use an Answer Key
Utilizing a resource that provides correct solutions can be an essential tool for improving your understanding and performance. It serves as a guide to verify your approach, check your work, and understand the reasoning behind each solution. This process not only helps with accuracy but also strengthens your problem-solving skills.
Here are a few reasons why having access to a reliable solution guide can be beneficial:
- Improved Learning: Reviewing correct solutions helps you identify mistakes and learn the proper methods for solving similar problems.
- Increased Confidence: Knowing that you are on the right track boosts your confidence and encourages further practice.
- Efficient Study: Instead of struggling with every problem, you can focus on areas that need improvement while confirming the ones you’ve already mastered.
- Better Time Management: Quickly checking your work saves time, allowing for more practice in other areas that require attention.
Incorporating these tools into your study routine enables you to work more efficiently and effectively. It also fosters a deeper understanding of key concepts, leading to stronger performance in exams and real-world applications.
Step-by-Step Problem Solving Guide

Solving problems effectively requires a structured approach that breaks down complex tasks into smaller, manageable steps. This method helps you stay organized, identify patterns, and apply the right techniques to find the correct solution. Whether you’re facing a simple calculation or a more challenging puzzle, following a clear process ensures better results and fewer mistakes.
Here’s a general approach you can follow to tackle problems with confidence:
- Understand the Problem: Read through the problem carefully and identify what is given and what is being asked. Clarify any unclear terms or concepts.
- Devise a Plan: Think about what strategies or methods are needed to solve the problem. This could involve applying known formulas or techniques.
- Execute the Plan: Work through the problem step by step, applying the methods and calculations as planned. Stay organized to avoid skipping important details.
- Review and Check: Once you’ve reached a solution, review your work to ensure it makes sense and that no errors were made during the process. Double-check your calculations and logic.
By following this approach, you can increase your efficiency and accuracy, leading to better problem-solving skills. With consistent practice, this structured method will become second nature, helping you excel in both assignments and exams.
Key Topics Covered in Grade 10 Math
Throughout the learning journey, students encounter a range of topics that are designed to strengthen their understanding of fundamental concepts. These subjects not only build on prior knowledge but also introduce new ways to approach and solve problems. Mastering these areas helps students develop the skills needed for more advanced studies and practical applications.
The following are some of the key areas typically covered at this level:
- Algebra: Solving equations, working with variables, and understanding functions. Students learn how to manipulate algebraic expressions and solve linear equations.
- Geometry: Study of shapes, angles, and properties of figures. This includes calculating areas, perimeters, and understanding theorems related to lines and angles.
- Trigonometry: Understanding the relationship between angles and sides of triangles. Students explore sine, cosine, and tangent functions to solve real-world problems.
- Statistics: Collecting and analyzing data, calculating averages, and interpreting graphs. This topic introduces probability and its applications in everyday situations.
- Polynomials: Working with terms and expressions that include powers of variables. This includes addition, subtraction, and factoring of polynomials.
These subjects provide the foundation for more complex topics in later years. Focusing on these areas helps learners improve their critical thinking skills and prepares them for real-world problem solving.
Importance of Practice for Success
Consistency and repetition are essential when it comes to mastering any skill. The more you engage with problems and challenges, the stronger your ability to solve them becomes. Practice helps reinforce concepts, improve accuracy, and increase confidence. Over time, it enables learners to approach tasks with ease, finding solutions more quickly and efficiently.
Building Strong Foundations
Regular practice ensures that core concepts are well understood and retained. By revisiting problems and attempting different approaches, students can uncover gaps in their knowledge and address them before they become larger obstacles. This builds a solid foundation for more advanced topics and improves overall performance.
Boosting Problem-Solving Skills
Problem-solving requires not just knowledge, but the ability to think critically and creatively. Through continuous practice, students learn to recognize patterns, choose the right strategies, and apply their understanding to new situations. This ongoing process sharpens both their analytical and logical thinking skills.
| Benefit | How Practice Helps |
|---|---|
| Confidence | Regular practice increases familiarity with different problems, boosting self-assurance in solving them. |
| Efficiency | Frequent engagement allows for faster problem-solving, reducing time spent on each task. |
| Concept Retention | Repeating exercises helps reinforce understanding and ensures key ideas are retained long-term. |
In the long run, consistent practice not only enhances immediate performance but also builds the skills necessary for continued success in future challenges. A steady approach ensures that learners remain prepared and capable, no matter the complexity of the task at hand.
Tips for Effective Study
Mastering any subject requires more than just reading through the material. It’s about developing a methodical approach that helps you retain information, apply concepts, and build your problem-solving skills. A well-structured study plan ensures that you understand the material deeply and can recall it when needed.
Create a Study Schedule
One of the most effective ways to stay on track is by organizing your time. A study schedule allows you to allocate time for each topic, ensuring that you cover everything without feeling overwhelmed. Consistency is key – regular short study sessions are often more effective than cramming at the last minute.
Focus on Understanding, Not Memorizing
Instead of simply memorizing formulas or steps, focus on understanding the reasoning behind them. This deeper comprehension allows you to apply concepts to new problems with greater ease. Take time to explore different methods for solving problems, and challenge yourself to explain the logic behind your solutions.
Additional Tips for Success:
- Practice Regularly: Consistent practice helps solidify concepts and improves your ability to solve problems accurately.
- Break Down Complex Problems: Don’t get discouraged by difficult questions. Break them into smaller, manageable parts and solve each one step by step.
- Stay Organized: Keep your notes, assignments, and resources organized so that you can easily review them when needed.
- Ask for Help: If you’re stuck on a concept, don’t hesitate to seek assistance from a teacher or peer.
By incorporating these strategies into your study routine, you’ll not only improve your performance but also build confidence in your abilities. Effective study is about developing good habits that will support your learning journey in the long term.
Common Mistakes to Avoid in Math
In any subject, it’s easy to make errors, but recognizing and understanding these mistakes is key to improving your skills. Many common missteps in solving problems are not related to a lack of knowledge but to careless errors or overlooked details. By identifying these pitfalls, you can correct them and enhance your problem-solving abilities.
Here are some of the most frequent mistakes students encounter and how to avoid them:
Rushing Through Problems
When under time pressure, students often rush through questions, leading to small but significant mistakes. It’s crucial to take your time and carefully read through each problem. Skipping steps or misinterpreting the question can result in incorrect solutions. Make sure to double-check your work before finalizing your answer.
Misapplying Formulas
It’s easy to mistakenly use the wrong formula for a given problem, especially when the concepts are similar. To prevent this, always read the problem thoroughly and ensure you are applying the correct formula for the task at hand. If in doubt, take a moment to review your notes or the problem itself to confirm the best approach.
Forgetting to Simplify
After completing calculations, students sometimes forget to simplify their results. Leaving answers in their raw form can make them more difficult to interpret or compare with others. Always check that your final answer is in its simplest form, especially when working with fractions, exponents, or radical expressions.
Additional Tips for Avoiding Mistakes:
- Show Your Work: Writing out every step helps you spot errors and ensures that you don’t miss important calculations.
- Understand the Problem: Ensure you clearly understand what the problem is asking before jumping to solve it. Misreading the question is a common issue.
- Check Units and Labels: Always check that you’re using the correct units, particularly in word problems or real-life applications. Mixing up units can lead to incorrect answers.
- Stay Organized: Keeping your work neat and well-organized makes it easier to follow your reasoning and identify any errors you might have overlooked.
Avoiding these common mistakes will not only improve your performance but also help you approach problems with greater confidence and accuracy.
How to Use the Answer Key Effectively
Having access to a solution guide can be incredibly helpful when learning complex topics. However, it’s important to use this resource properly to truly benefit from it. Instead of simply copying answers, a more effective approach is to use the guide to deepen your understanding and improve your problem-solving skills.
Check Your Work, Not Just the Final Answer
One of the best ways to use a solution guide is to check your process rather than just the final result. After completing a problem, compare your steps with those in the guide. If your answer differs, review where you might have made a mistake and try to identify the step that went wrong. This will help you learn from your errors and avoid repeating them in the future.
Understand the Method, Don’t Just Copy
It’s easy to be tempted to look at the solution and copy the steps directly, but this won’t help you learn. Instead, focus on understanding how the solution was reached. Take note of the strategies used and the reasoning behind them. By doing this, you’ll be better equipped to apply similar approaches to different problems.
Here are some tips for using a solution guide effectively:
- Don’t Rush: Take your time to analyze each step in the solution guide. Make sure you understand how each part of the problem is solved before moving on.
- Use as a Learning Tool: Treat the solution guide as a teaching resource. Use it to clarify concepts you didn’t fully understand when first solving the problem.
- Cross-Check Multiple Sources: If the solution guide doesn’t explain a step clearly, try looking at other examples or resources to get a better understanding.
- Review After Each Attempt: Always go back to the solution guide after completing a set of problems. This helps reinforce what you’ve learned and shows you areas that still need improvement.
When used properly, a solution guide can be a powerful tool to improve your skills and knowledge, helping you solve problems more confidently and accurately in the future.
Understanding the Structure of Learning Resources
When tackling any subject, it’s essential to understand the structure of the learning material you’re working with. These resources are typically organized in a way that builds upon previous knowledge, ensuring that you grasp foundational concepts before moving on to more complex topics. Understanding this structure allows you to approach the content systematically and efficiently.
Overview of Learning Resource Layout
Most educational materials are divided into distinct sections, each focusing on a specific area of study. These sections are carefully designed to introduce new concepts progressively. The structure is meant to help you connect ideas, build your skills step by step, and practice what you’ve learned.
- Introduction: The opening section provides an overview of the key concepts, objectives, and skills you are expected to master.
- Theory and Concepts: This part explains the core principles and formulas you will need to understand. It often includes examples and visual aids.
- Practice Problems: This section allows you to apply what you’ve learned through exercises that test your understanding and ability to solve related problems.
- Review and Summary: The final section recaps the material, often with additional tips or a summary of important points to reinforce your learning.
How to Navigate the Material Effectively
Knowing the structure helps you navigate the resource in a more organized manner. Here are a few tips for making the most of the layout:
- Focus on Mastery: Don’t rush through sections. Ensure that you fully understand each concept before moving on to the next.
- Use the Practice Problems Wisely: Use the exercises not just to check your knowledge, but to reinforce it. Practice problems often highlight areas where you need more work.
- Review Regularly: Regularly revisit earlier sections to ensure the material stays fresh in your mind.
- Seek Clarification: If you don’t understand a concept or step, seek clarification from additional resources, teachers, or peers.
By understanding the structure of your learning material and utilizing it effectively, you can improve your retention, enhance your problem-solving abilities, and build a strong foundation for mastering complex topics.
Focus Areas for Better Performance
Improving performance in any subject requires careful attention to certain areas that directly impact your understanding and ability to solve problems. By concentrating your efforts on these key focus areas, you can enhance both your skills and confidence. Identifying these critical aspects helps ensure that you are not only memorizing information but truly mastering the content.
Strengthen Core Concepts

It’s essential to develop a strong grasp of the fundamental principles that underpin the subject. Without a solid foundation, advancing to more complex material can become increasingly challenging. Focus on understanding the logic behind each concept, as this will make problem-solving easier and more intuitive.
- Master the Basics: Take the time to thoroughly understand core principles before moving to more advanced topics.
- Recognize Patterns: Identifying recurring patterns in problems will help you apply the right techniques faster and with more accuracy.
- Build a Conceptual Framework: Understand how different concepts are connected, creating a mental map of the material that you can refer to when solving problems.
Improve Problem-Solving Skills
Problem-solving is at the heart of mastering any subject. It’s not just about knowing the answers but about knowing how to approach and tackle challenges. Practicing regularly will help you become more efficient at recognizing the correct methods and applying them accurately.
- Work Through Examples: Review and solve various examples to familiarize yourself with different problem types and techniques.
- Analyze Mistakes: When you make an error, take the time to understand why it happened and how you can avoid it in the future.
- Develop a Step-by-Step Approach: Break down problems into smaller, manageable steps to ensure accuracy and avoid rushing through solutions.
Stay Organized and Consistent
Maintaining an organized study routine can significantly impact your overall performance. Regular review and consistent practice are vital in retaining information and developing proficiency in solving problems. Keep track of your progress and address any gaps in understanding promptly.
- Set a Study Schedule: Dedicate specific time slots for practice and review to ensure you stay on track with your learning goals.
- Review Regularly: Consistent review helps reinforce material and prevents forgetting key concepts.
- Track Your Progress: Keep a record of completed exercises and areas of difficulty to monitor improvement over time.
By focusing on these key areas–strengthening your core knowledge, enhancing problem-solving techniques, and staying organized–you can significantly boost your performance and achieve greater success in your studies.
Preparing for Exams with Practice Solutions
Effective preparation for any exam involves understanding the material deeply and practicing solving problems. Using practice solutions can be an invaluable tool in this process, allowing you to verify your understanding and correct mistakes. By carefully reviewing these solutions, you can reinforce your skills, identify areas of weakness, and approach the exam with greater confidence.
How to Utilize Practice Solutions

To get the most out of practice resources, it’s important to use them strategically. Simply reviewing the correct solutions is not enough; active engagement with the material is key. Follow these steps to ensure your preparation is effective:
- Attempt Problems First: Always try solving problems on your own before checking the solutions. This helps you identify where you need improvement.
- Compare Your Solutions: After solving, compare your answers to the provided solutions. Understand the steps taken and see if you missed any important details.
- Focus on Mistakes: Pay close attention to the errors you make. Understand why your approach didn’t work and how you can improve it.
- Review Step-by-Step: Break down each problem into smaller steps and ensure you understand each phase of the solution. This will improve your problem-solving process.
Maximizing Your Review Sessions
Maximize your review sessions by focusing on high-yield problems and areas that you find most challenging. Effective use of practice solutions also includes revisiting concepts that are difficult for you, even if the solutions seem easy. This will help you build confidence and prevent confusion during the actual exam.
| Step | Action | Benefit |
|---|---|---|
| 1 | Attempt the problems independently | Develops confidence and reinforces knowledge |
| 2 | Check your solutions | Identifies areas for improvement and corrects misunderstandings |
| 3 | Analyze your mistakes | Prevents recurring errors and deepens understanding |
| 4 | Revisit challenging concepts | Strengthens weaker areas and boosts overall performance |
By following these strategies, you can use practice solutions to effectively prepare for your exams. The goal is not just to get the right answers but to understand the underlying principles, improving both your knowledge and exam-taking skills.
How to Check Your Work Accurately
Checking your work thoroughly is a critical part of the problem-solving process. It ensures that no mistakes were made and helps to reinforce your understanding of the concepts. Whether you’re solving a set of equations or tackling complex problems, a systematic approach to reviewing your steps can lead to more accurate results. Below are strategies for effectively checking your solutions to avoid common errors.
Step-by-Step Review Process
To verify your work, follow a methodical process that includes the following steps:
- Start with the Final Answer: Double-check the final result. Ask yourself if it makes sense in the context of the problem and whether it fits within the expected range or pattern.
- Revisit Each Step: Go through your calculations or logic one step at a time. Confirm that each step follows logically from the previous one and that no shortcuts or mistakes were made.
- Check for Consistency: Ensure that your units, symbols, and operations are consistent throughout the problem. Inconsistencies can lead to incorrect results.
- Compare with Known Methods: Cross-reference your approach with standard methods or procedures for solving similar problems. This helps to identify any missed or incorrect techniques.
Common Pitfalls to Avoid

While reviewing your work, be mindful of these common pitfalls:
- Overlooking Small Details: Even small errors, such as forgetting a negative sign or misplacing a decimal, can have a significant impact on the result.
- Relying Solely on a Calculator: A calculator can help, but it’s important to understand the process behind the solution. Blindly trusting a calculator can cause you to miss conceptual errors.
- Skipping Steps: Skipping intermediate steps may save time initially, but it makes it harder to trace errors. Always show your work.
- Not Taking Breaks: Reviewing work when you’re fatigued can lead to overlooking mistakes. Step away for a moment and return with fresh eyes.
By following these strategies and being mindful of common errors, you can ensure that your solutions are accurate and well-understood. Consistent and careful checking will improve your confidence and ability to tackle future problems with precision.
Utilizing Online Resources for Help
In today’s digital age, there are countless online resources available to support learners in understanding complex concepts. These platforms provide instant access to tutorials, explanations, and practice problems that can help clarify difficult topics. By leveraging the power of the internet, students can enhance their learning experience and fill in any gaps in their knowledge. Below are some ways to effectively use online tools to strengthen your understanding.
Types of Online Resources
There are various online platforms designed to assist with different aspects of learning. Some of the most helpful types of resources include:
- Tutorial Websites: Websites like Khan Academy, Coursera, and edX offer free video lessons on a wide range of subjects. These tutorials often break down complex topics into digestible segments, making it easier for students to grasp key ideas.
- Online Forums: Websites such as StackExchange or Reddit provide communities where learners can ask questions, share ideas, and receive guidance from experts and fellow students. Engaging in these forums can help clarify doubts and offer different perspectives on a problem.
- Practice Problem Websites: Platforms like IXL, Brilliant.org, and Wolfram Alpha provide a wealth of practice questions and step-by-step solutions. These can be invaluable for reinforcing concepts and testing your skills.
- Interactive Learning Tools: Some websites offer interactive exercises that let you solve problems in real-time, helping to reinforce your understanding through immediate feedback and explanations.
Tips for Using Online Resources Effectively
While online resources are helpful, using them strategically will maximize their benefits. Here are some tips to make the most of these tools:
- Stay Organized: Keep track of which resources you’ve used and which topics you’ve covered. This will help you avoid confusion and ensure you don’t skip essential material.
- Practice Regularly: Consistency is key. Make a habit of using online resources daily to reinforce your learning and stay ahead in your studies.
- Engage with the Community: Don’t just passively watch tutorials or read articles–participate in discussions, ask questions, and help others when possible. Active learning can deepen your understanding.
- Set Clear Goals: Focus on specific topics or areas of difficulty, and use online resources to target these areas. Having a clear goal will keep your learning process focused and efficient.
By effectively utilizing online tools and platforms, you can deepen your understanding and improve your problem-solving skills. These resources offer a wealth of information at your fingertips and can be a great supplement to traditional studying methods.
Test Preparation Tips

Preparing effectively for an upcoming evaluation requires both strategic planning and disciplined study. By focusing on key concepts, practicing regularly, and utilizing available resources, students can improve their performance and feel more confident when facing the exam. Here are some helpful tips to ensure you’re fully prepared for the test ahead.
Establish a Study Routine
Creating a structured study schedule can help ensure that you cover all the necessary material without feeling overwhelmed. Consistent practice over time is much more effective than cramming at the last minute. Consider the following strategies:
- Divide Topics into Manageable Sections: Break the material into smaller, more digestible chunks. Focusing on one topic at a time makes it easier to retain information.
- Set Specific Goals for Each Session: Each study session should have a clear goal. For example, aim to master one particular concept, like solving linear equations or working with geometric shapes.
- Review Regularly: Don’t just move on once you’ve learned something new. Regular review helps reinforce your understanding and ensures that the material stays fresh in your mind.
Use Practice Tests and Resources
Practice tests are a great way to simulate the exam environment and assess your readiness. Additionally, other learning resources can provide further support. Here are some recommendations:
- Take Timed Practice Tests: Simulate the test conditions by setting a timer and completing practice tests within the allotted time. This will help you manage time more effectively during the actual exam.
- Review Mistakes Thoroughly: After completing a practice test, carefully go over your mistakes. Understanding why you got a question wrong is crucial for improving your performance.
- Use Online Resources: Many websites offer interactive exercises, practice problems, and explanations. Make use of these platforms to reinforce your understanding of difficult concepts.
By following these tips, you can approach your upcoming test with a focused mindset and the confidence that comes from thorough preparation. Staying organized and practicing regularly are essential to achieving the best possible results.
Tracking Your Progress in Math
Monitoring your growth and development in any subject is a vital part of the learning process. By consistently evaluating your understanding, you can identify areas of strength and pinpoint topics that require further attention. Tracking progress allows you to adjust your study strategies, stay motivated, and see tangible improvements over time.
Set Clear Milestones
Setting achievable goals is a powerful way to stay on track. These milestones act as checkpoints to measure how well you’re progressing. Consider the following when setting your goals:
- Break Down Large Goals: Instead of focusing on the overall subject, break it down into smaller, manageable tasks. This can include mastering specific concepts, such as working with fractions or understanding algebraic expressions.
- Set Weekly Targets: Focus on what you want to accomplish each week. This can include completing a set of practice problems, reviewing notes, or tackling more complex topics.
- Celebrate Small Achievements: When you reach a milestone, take a moment to recognize your success. This helps maintain motivation and encourages continued effort.
Utilize Self-Assessments and Feedback
Regular self-assessment allows you to evaluate your own understanding, while feedback from others provides valuable insight into areas for improvement. Consider using the following methods:
- Take Practice Tests: Use practice tests to gauge your understanding of key concepts. Reviewing your results will highlight both your strengths and areas that need more attention.
- Seek Feedback from Peers or Teachers: Ask for feedback from someone knowledgeable to gain insight into how you can improve. Constructive criticism can help you refine your approach.
- Track Mistakes and Progress: Keep a record of errors and mistakes. By revisiting these mistakes and understanding them, you can avoid repeating them in the future and track how much you’ve improved.
Tracking your progress is an ongoing process. It helps build confidence and ensures that you’re consistently improving. Whether through regular self-assessments or feedback, monitoring your growth allows for more effective learning and better results.
Building Confidence in Problem Solving
Developing a strong sense of confidence in solving problems is essential for success in any field. By mastering techniques and strategies, you can approach challenges with a positive mindset and greater ease. Building confidence involves understanding concepts thoroughly, practicing regularly, and recognizing your progress over time.
Start with Simple Problems
When you begin solving problems, it’s important to start with simpler tasks. This allows you to build a strong foundation and gain confidence before moving on to more complex challenges. Consider these steps:
- Master Basic Concepts: Ensure you fully understand the fundamental ideas behind the problems. A solid grasp of basics makes tackling harder tasks much easier.
- Progress Gradually: Begin with easy examples and slowly increase the difficulty level. This helps avoid feelings of overwhelm and builds a sense of achievement.
- Repeat to Reinforce: Practice similar problems multiple times. Repetition builds muscle memory and boosts your belief in your abilities.
Embrace Mistakes as Learning Opportunities
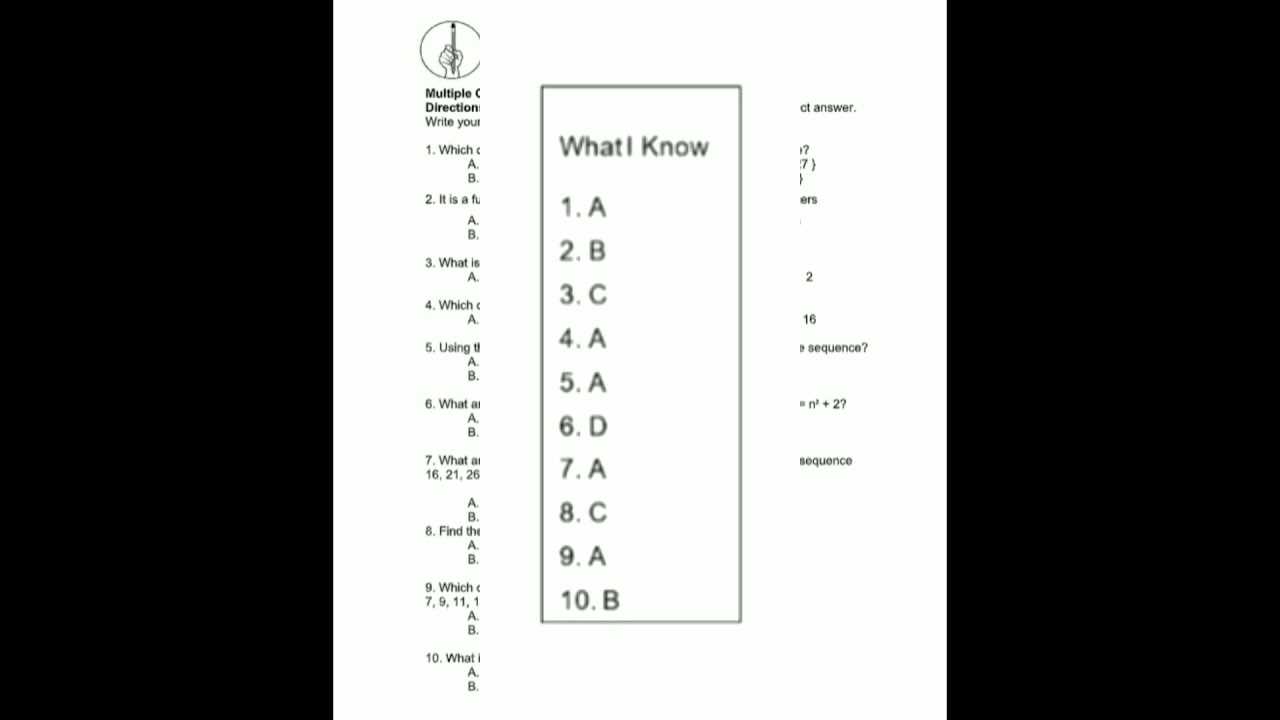
Confidence grows when you view mistakes as part of the learning process rather than failures. Every error is an opportunity to understand a concept more deeply and refine your approach. Here’s how to make the most of mistakes:
- Review Mistakes: After making an error, go back and understand where you went wrong. Analyzing mistakes helps prevent them from happening again.
- Learn from Feedback: Whether from peers or instructors, feedback provides valuable insights on how to improve. Constructive criticism is an essential tool for growth.
- Stay Positive: Keep a positive attitude when faced with setbacks. A resilient mindset encourages perseverance and fosters growth.
By consistently practicing and reflecting on your progress, your confidence will naturally increase. Building problem-solving confidence is a journey that requires patience and persistence, but the results are well worth the effort.