
Preparing for an advanced mathematics test requires a strategic approach to mastering complex concepts and applying problem-solving techniques. A detailed analysis of past assessments can provide valuable insights into the types of questions and the best ways to approach them. In this section, we will break down the key questions from a previous year’s test and walk you through each one step by step.
Understanding the structure of the test is essential for effective preparation. Whether you’re dealing with multiple-choice problems or open-ended solutions, it’s important to familiarize yourself with the types of challenges that may arise. This guide will offer clear explanations and solutions, helping you navigate through each section with confidence.
By reviewing detailed solutions, you can enhance your problem-solving skills and gain a deeper understanding of the subject matter. This article serves as a helpful resource for anyone looking to strengthen their grasp on advanced mathematical principles and improve performance in future assessments.
2008 AP Calculus AB Exam Answers
In this section, we will explore solutions to a set of complex mathematical problems presented in a prior year’s assessment. By carefully analyzing each question and breaking it down into manageable steps, you can develop a better understanding of how to approach similar challenges in the future. Whether it’s a question focused on derivatives, integrals, or real-world applications, this guide provides you with practical strategies for tackling tough problems.
Understanding Problem Types
Each section of the test typically contains a variety of problem types, ranging from theoretical questions to those requiring calculations. It’s crucial to recognize the structure of these problems, as each demands different strategies. For example, solving word problems requires translating the situation into a mathematical model before applying the appropriate methods. Being able to identify the core issue and determine the best approach is key to success.
Step-by-Step Solutions
Here, we provide clear and concise steps for each question, guiding you through the reasoning process and showing how to reach the correct conclusion. With careful attention to detail, you can improve your problem-solving speed and accuracy. By practicing these steps, you’ll not only enhance your understanding of the material but also gain confidence in handling similar challenges in the future.
Overview of the 2008 Exam Format
The structure of a standardized test is designed to assess a student’s understanding of key concepts and their ability to apply problem-solving techniques. In this section, we will look at how the assessment was organized, highlighting the different sections and types of questions included. Understanding the format is essential for effective preparation and can help in managing time during the actual test.
Typically, the test is divided into two main parts: multiple-choice questions and free-response problems. Each part has its own unique format and requirements, testing various skills such as critical thinking, computation, and the ability to demonstrate reasoning in written form. By familiarizing yourself with the types of questions and their layout, you can approach the test with a strategic mindset.
Key Topics Covered in the Exam
To perform well on a high-level mathematics test, it’s essential to understand the main concepts and topics that are commonly covered. The assessment focuses on a wide range of mathematical principles, from basic to advanced techniques. Mastery of these topics is crucial for achieving a high score and demonstrating a solid grasp of the material.
Core Areas of Focus
- Functions and their properties
- Limits and continuity
- Derivatives and their applications
- Integration techniques and applications
- Analysis of real-world problems using mathematical models
Specific Concepts Explored
- Understanding the concept of instantaneous rate of change
- Interpreting graphs and analyzing slope behavior
- Utilizing the Fundamental Theorem of Calculus
- Applying techniques for approximating areas under curves
- Exploring differential equations and their solutions
By familiarizing yourself with these topics, you can approach each section of the test with confidence and a clear understanding of the material. Practicing these concepts will help you apply the correct methods when faced with similar questions on the test.
Understanding Multiple-Choice Questions
Multiple-choice questions are designed to test a student’s ability to quickly recall information and apply specific concepts to a given problem. These questions usually present a problem followed by a set of possible answers, requiring you to identify the correct solution. To excel in this section, it’s important to approach each question methodically and eliminate incorrect options based on logical reasoning.
Strategies for Success
- Read the question carefully before reviewing the choices.
- Look for keywords or phrases that hint at the correct approach.
- Eliminate clearly incorrect options to improve your odds.
- If unsure, try to solve the problem mentally before selecting an answer.
- Check for patterns in similar questions to recognize common traps.
Common Pitfalls to Avoid
- Don’t rush through questions–take your time to read carefully.
- Don’t assume answers are in a particular order; look at all options.
- Avoid second-guessing your initial choice without a good reason.
- Don’t skip over questions that seem difficult–work through them logically.
By following these strategies and avoiding common mistakes, you can enhance your performance in the multiple-choice section and boost your overall score. Practicing with similar problems can also improve your confidence and help you develop a solid approach to each question.
How to Tackle Free Response Questions
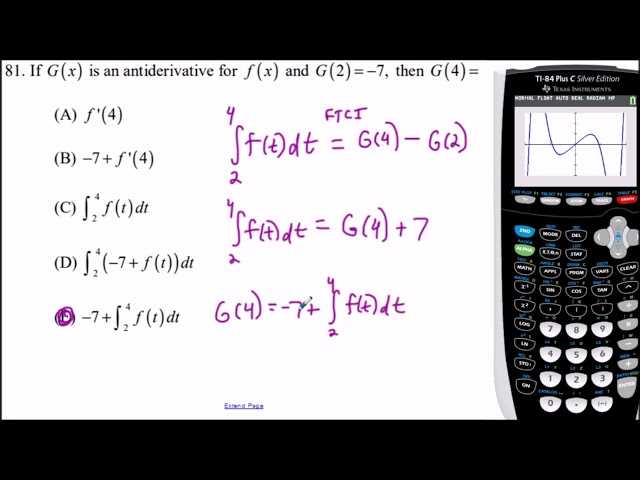
Free response questions are designed to assess your ability to apply mathematical principles and clearly communicate your thought process. Unlike multiple-choice questions, these require you to show all steps involved in solving the problem. A well-organized response not only demonstrates your understanding but also maximizes the points you can earn.
When approaching these questions, it’s essential to break down the problem into smaller, manageable parts. Start by understanding what is being asked, then outline the steps necessary to solve the problem. Always show your work clearly and logically, as partial credit can often be awarded for correct methods even if the final answer is incorrect.
- Read the problem carefully and identify key information.
- Write down any formulas or equations that may be useful.
- Work through the problem step by step, showing all calculations and reasoning.
- Check your work for accuracy before submitting your response.
By maintaining clarity and organization in your responses, you increase the likelihood of earning full credit for each part of the problem. Practicing free response questions can help you refine your approach and improve your performance on similar tasks in the future.
Solutions for the 2008 Exam Questions
This section provides detailed solutions to the problems presented in the prior year’s assessment. By carefully examining the steps involved in each solution, you can gain a deeper understanding of the techniques and strategies used to solve complex problems. Each solution is broken down step by step to help you grasp the methods applied and improve your problem-solving skills.
For each problem, we outline the necessary formulas and explain how to apply them in the context of the given question. Whether it’s a question involving rates of change, areas under curves, or optimization, understanding the reasoning behind the solution is key to mastering similar problems in the future. By working through these solutions, you can improve your ability to solve problems quickly and accurately.
Take the time to review each step in detail, and make sure you understand why each approach is used. This will allow you to apply these techniques effectively on similar questions in the future, ensuring success in upcoming assessments.
Step-by-Step Guide to Question 1
This section walks you through the solution to the first problem from the assessment, breaking it down into clear and manageable steps. Understanding the methodology behind solving such a problem will give you the tools to approach similar challenges with confidence. We will go through the key concepts and techniques required to find the correct solution, ensuring each step is well understood.
Step 1: Understand the Problem
Carefully read the question and identify the key information provided. This may include variables, functions, or conditions that you will need to incorporate into your solution. Take note of any specific instructions or constraints given in the problem.
Step 2: Set Up the Solution
Determine the appropriate approach to solving the problem. This could involve applying a specific formula, setting up an equation, or choosing the correct method for integration or differentiation. Be sure to write down all necessary formulas and relevant information.
Step 3: Solve Step-by-Step
Work through the problem methodically. Show all calculations and explain your reasoning at each step. By clearly documenting your process, you demonstrate a complete understanding of the material, even if you make a minor error along the way.
Step 4: Check Your Work
After completing the solution, revisit the problem to ensure that all steps are correct and the final result makes sense in the context of the question. Checking your work can help you catch any mistakes and improve your accuracy for future problems.
By following these steps, you can improve your problem-solving skills and feel more prepared for similar questions in future assessments.
Step-by-Step Guide to Question 2

This section provides a detailed breakdown of how to approach the second problem from the assessment. The goal is to walk through the process step by step, demonstrating the logical progression and methods used to arrive at the solution. By carefully following each stage, you’ll build a solid foundation for tackling similar questions in the future.
To begin, it’s essential to understand the type of problem you’re dealing with and the specific concepts it requires. Once that’s clear, we can proceed with the solution in a structured manner.
| Step | Description |
|---|---|
| Step 1: Analyze the Given Information | Identify all known variables and functions provided in the problem. Pay attention to any conditions or relationships that need to be used in solving the question. |
| Step 2: Select the Appropriate Method | Based on the problem type, choose the best mathematical method to solve it. This could involve integration, differentiation, or applying a specific theorem. Write down the formulas or principles you’ll use. |
| Step 3: Work Through the Calculations | Execute the necessary calculations carefully. Break them into smaller parts and show each step to avoid errors. Use proper notation and include all intermediate results. |
| Step 4: Interpret the Result | After completing the calculation, interpret your result in the context of the problem. Verify if the outcome is reasonable and corresponds with the question’s conditions. |
| Step 5: Verify Your Solution | Double-check each step to ensure accuracy. This includes revisiting any formulas or steps where errors could occur. If possible, consider alternative methods to validate your result. |
By following this structured approach, you’ll gain a deeper understanding of the material and increase your ability to solve similar problems accurately and efficiently in the future.
Step-by-Step Guide to Question 3
This section provides a thorough walkthrough of the third question from the assessment, guiding you through each step of the solution process. The goal is to break down the problem logically, illustrating how to approach complex concepts and applying the appropriate techniques. By the end of this guide, you should have a clear understanding of how to solve similar problems in future assessments.
Step 1: Analyze the Given Information
Begin by reviewing all the information provided in the question. Identify key variables, functions, and any constraints or conditions that are part of the problem. Understanding these elements will help you determine the best approach for solving the problem.
Step 2: Select the Correct Approach
Based on the analysis, choose the most effective method to tackle the problem. This might involve applying a specific mathematical rule or using a particular technique such as optimization, finding limits, or solving an equation. Make sure to write down the relevant formulas or equations you’ll need for the solution.
It’s important to break the problem into smaller, manageable steps to ensure accuracy at each stage. Carefully work through each part of the problem, showing all intermediate steps and clearly explaining the reasoning behind each action. This will not only improve your understanding but also help maximize the points you can earn for the solution.
Step-by-Step Guide to Question 4
This section breaks down the fourth question from the assessment into clear, manageable steps. By following each phase of the solution process, you will be able to master the key techniques needed to address similar problems. This guide emphasizes logical thinking and methodical problem-solving skills that are crucial for success in such assessments.
The first step is to carefully examine the question, identify the core concepts involved, and determine which strategies to employ. By following a structured approach, you can effectively work through the problem without feeling overwhelmed.
Step 1: Identify Key Information
Start by analyzing the problem statement. Take note of important details such as variables, functions, and any specific conditions. These elements will help you choose the right method and tools for solving the problem. Make sure you understand exactly what the question is asking for.
Step 2: Organize Your Approach
Once you’ve understood the question, break the problem into smaller, manageable parts. Consider the most appropriate mathematical method, whether it’s differentiation, integration, or algebraic manipulation. Here’s how to organize your approach:
- Write down all known information clearly.
- Identify the formula or technique you need to use.
- Set up the problem step by step.
Step 3: Solve the Problem
With a clear plan in place, start solving the problem. Apply the chosen methods carefully, showing all intermediate steps. It’s important to keep track of your calculations and to follow each logical step to reach the final result. Make sure to include units or context if the problem requires them.
Step 4: Verify Your Solution
After completing the solution, double-check your work. Ensure all calculations are correct and that the final answer is consistent with the problem requirements. If possible, verify your result through an alternative method or by using a different approach to check for consistency.
By following these steps, you will be well-equipped to tackle similar problems in the future, gaining both confidence and proficiency with each new question.
Analyzing the Results of Your Assessment

After completing a challenging test, it’s essential to thoroughly analyze your results. This step helps you understand both your strengths and areas where improvement is needed. By breaking down your performance, you can identify patterns, pinpoint mistakes, and build a strategy for future success.
Step 1: Review Each Question
Start by reviewing every question in detail. For each one, ask yourself the following:
- Did I fully understand the question and its requirements?
- Did I use the correct methods and techniques to solve it?
- Were there any calculation errors or oversights?
By focusing on these aspects, you’ll be able to identify which concepts or methods you may need to revisit in more depth.
Step 2: Identify Patterns in Mistakes
Next, look for common themes in the errors you made. For example, if you struggled with certain types of problems (e.g., solving integrals or interpreting graphs), make note of these recurring issues. Recognizing these patterns is key to improving in future assessments.
- Did I miss key details in the question or misinterpret a concept?
- Were my mistakes more procedural (e.g., wrong formula or calculation) or conceptual (e.g., misunderstanding the theory)?
Step 3: Make a Plan for Improvement
Once you’ve identified the problem areas, create an action plan for improvement. This might include:
- Reviewing textbook examples or practice problems on the topics you struggled with.
- Seeking help from a teacher or tutor to clarify any unclear concepts.
- Regularly practicing similar questions to reinforce your skills.
Analyzing your performance in this way will not only help you improve but also make you more prepared for future challenges, turning your mistakes into learning opportunities.
How to Use 2008 Answers for Practice
Using previous solutions as a reference for practice is an effective way to enhance your problem-solving skills. By working through each problem and comparing your approach to the provided solutions, you can identify areas for improvement and reinforce your understanding. This method allows you to not only test your knowledge but also to better grasp the concepts and techniques required for solving complex problems.
When practicing with these solutions, it’s important to follow a structured approach. Begin by attempting each problem on your own before reviewing the provided solutions. This will give you the opportunity to test your ability to recall information and apply methods. Once you have completed a problem, compare your solution to the example answer and note any discrepancies or mistakes.
Step 1: Work Through Problems Independently

Before looking at any solutions, try to solve the problem on your own. This will help you focus on the process and build confidence in your abilities. Keep track of your thought process and write down all the steps you take to reach the answer.
Step 2: Compare Your Approach to the Provided Solutions
Once you’ve solved the problem, compare your solution with the reference answers. Pay close attention to the following:
- Do you follow the same steps as the solution?
- Are there any alternative methods that might have been more efficient?
- Have you made any calculation errors or missed any key steps?
Reviewing the solutions carefully will allow you to identify patterns, learn different approaches, and deepen your understanding of the material.
Step 3: Focus on Weak Areas
If you find areas where your approach differs from the provided solutions, take the time to review and practice these topics. By addressing these weak spots, you will ensure that you’re prepared to handle similar problems in the future.
| Area for Improvement | Suggested Practice |
|---|---|
| Incorrect Formula Application | Review relevant formulas and practice applying them in various contexts. |
| Conceptual Understanding | Revisit textbook examples and explanations to clarify misunderstood concepts. |
| Calculation Mistakes | Work through similar problems with a focus on accuracy and careful review. |
By practicing with these solutions regularly and analyzing your progress, you’ll be able to improve your performance and gain a better grasp of the material for future assessments.
Common Mistakes and How to Avoid Them
When tackling complex problems, it’s easy to fall into certain traps that can negatively affect your performance. Whether it’s overlooking key details, making calculation errors, or misinterpreting the problem, these common mistakes can be avoided with some careful attention and practice. Recognizing where these mistakes often occur allows you to focus on correcting them and improving your problem-solving approach.
Here are some of the most frequent errors and strategies for preventing them:
1. Misunderstanding the Question
One of the most common issues is failing to fully grasp the question before attempting to solve it. This can lead to wasted time and incorrect solutions. Always take a moment to read the problem carefully, underline key information, and identify what is being asked.
- Tip: Break the problem into smaller parts to ensure you understand what’s being asked at each step.
- Tip: Rephrase the question in your own words to confirm your understanding.
2. Incorrect Application of Formulas
Using the wrong formula or applying it incorrectly can result in an entirely wrong answer. This is particularly common in problems that involve multiple steps or concepts.
- Tip: Double-check which formula is required for the given problem. Keep a list of commonly used formulas handy during practice.
- Tip: Before applying a formula, ensure that all variables are correctly substituted and that units are consistent.
3. Calculation Errors
Simple arithmetic mistakes can derail an otherwise correct solution. These errors are often the result of rushing through steps or not checking your work.
- Tip: Use a systematic approach when solving calculations. Take time to carefully complete each step and verify your results.
- Tip: After solving, recheck your calculations by going through the problem again or using a calculator if available.
4. Ignoring Units or Not Labeling Answers
In technical problems, neglecting to include appropriate units can cost valuable points. Additionally, omitting labels or symbols in your final answer can confuse reviewers or lead to incomplete responses.
- Tip: Always include the proper units with your final answer and ensure that they are consistent with the problem’s context.
- Tip: Double-check that your final response includes any necessary labels or specific requirements, such as directions or specific numerical values.
5. Rushing Through the Problem

While time management is important, rushing through problems often leads to mistakes. When trying to complete the assessment quickly, it’s easy to overlook details or skip necessary steps.
- Tip: Take the time to understand each part of the problem and ensure that you are not skipping any critical steps.
- Tip: Practice working under time constraints, but always prioritize accuracy over speed during preparation.
By being mindful of these common mistakes and adopting strategies to prevent them, you can significantly improve the quality of your solutions and increase your chances of success. Regular practice, attention to detail, and careful review of your work are essential to overcoming these obstacles and mastering the material.
Time Management During the Exam
Efficient time management is crucial when taking a challenging assessment. With a limited amount of time and a variety of problems to solve, knowing how to allocate time properly can make a significant difference in your overall performance. Effective planning ensures that you are able to give each question the necessary attention, without rushing through the simpler ones or getting bogged down in more complex problems.
Here are some strategies to help you manage your time effectively:
1. Understand the Time Constraints
Before diving into the problems, take a moment to review the overall time limit and break it down by sections. Understanding how much time you should spend on each part of the assessment will prevent you from spending too long on a single question.
- Tip: If there are multiple sections with varying numbers of questions, allocate more time to sections with more questions, but also keep a buffer for the most challenging ones.
- Tip: Practice pacing yourself during your preparation to get a sense of how long it takes to solve similar problems.
2. Prioritize Your Approach
It’s important to approach the assessment with a strategy. Start with the questions that you feel most confident in to build momentum. Avoid spending too much time on questions that seem particularly difficult at first glance. It’s better to move on and come back to them later if time allows.
- Tip: Quickly skim through the entire assessment before starting to identify the easier questions. Tackle them first to maximize your score.
- Tip: If you get stuck on a question, mark it and move on to the next one. Come back to it later with fresh eyes.
By managing your time effectively, you can ensure that you complete all sections of the assessment to the best of your ability. With practice and careful planning, you’ll be able to tackle any challenge that comes your way within the time limit.
Scoring Breakdown of the 2008 Exam
Understanding how your performance is evaluated on a challenging assessment is essential for improving your approach. The scoring system is designed to give credit for both the accuracy of your answers and the approach you use to solve the problems. Different sections of the test contribute varying amounts to your final score, so it’s important to know how each part is weighted.
1. Multiple-Choice Section
The multiple-choice section typically accounts for a significant portion of the total score. This section tests your knowledge and ability to quickly apply concepts. Each question is scored based on the number of correct responses, with no penalty for incorrect answers. Understanding how to navigate this section efficiently can boost your score significantly.
- Tip: Focus on accuracy, but also manage time well to ensure you answer all questions.
- Tip: If unsure about a question, eliminate the clearly incorrect choices to increase your chances of guessing correctly.
2. Free-Response Section

The free-response section evaluates your ability to work through complex problems and clearly communicate your reasoning. In this section, partial credit is often awarded, so it’s important to show all steps involved in your process, even if you make an error in the final answer. The clarity of your explanations is as important as arriving at the correct result.
- Tip: Write out every step, even if it feels redundant. This allows for partial credit if your final answer is incorrect.
- Tip: Pay attention to the wording of each question and ensure that your answer matches the specific requirements.
Both sections contribute to the final score, with the free-response questions typically accounting for a larger portion of the total score. By focusing on both accuracy and the process behind your answers, you can optimize your performance and achieve a higher score on the test.
Tips for Improving Your Calculus Skills
Mastering advanced mathematical concepts requires a combination of practice, understanding, and strategic learning. Whether you’re struggling with specific topics or aiming for higher proficiency, there are several techniques to enhance your skills. Focusing on foundational concepts, improving problem-solving techniques, and consistently practicing can lead to noticeable improvement.
1. Strengthen Your Fundamentals
A deep understanding of the fundamental principles is crucial for tackling more advanced topics. Strengthening your knowledge of basic concepts such as limits, derivatives, and integrals lays the groundwork for more complex material.
- Review basic definitions: Make sure you’re clear on the core terms and their applications.
- Understand the “why”: Don’t just memorize formulas–understand the reasoning behind them.
- Relate concepts: Find connections between different topics to improve understanding and retention.
2. Practice Regularly
Mathematics is best learned through repetition. The more problems you solve, the more confident and efficient you will become. Focus on solving a variety of problems to expose yourself to different methods and techniques.
- Work on diverse problems: Try problems from different sources to get a wider range of examples.
- Use practice tests: Simulate test conditions to familiarize yourself with the timing and pressure of real assessments.
- Focus on weaknesses: Identify areas where you struggle and dedicate extra time to mastering those concepts.
By consistently building on foundational knowledge and practicing problem-solving, you can significantly improve your ability in advanced mathematical topics. Over time, this will increase both your confidence and your performance on tests.
Additional Resources for AP Calculus Prep
Preparing for an advanced mathematics test involves more than just reviewing class notes. Access to a variety of supplementary materials can help reinforce concepts, provide practice, and offer alternative explanations for difficult topics. By utilizing diverse resources, students can deepen their understanding and sharpen their problem-solving skills.
1. Online Learning Platforms
Many online platforms offer tutorials, exercises, and video lessons that can help you strengthen your knowledge. These resources often include interactive content and personalized learning paths to target areas where improvement is needed.
| Platform | Features |
|---|---|
| Khan Academy | Free video tutorials, practice problems, and detailed explanations for various topics. |
| Coursera | Courses offered by universities with structured lessons and assessments. |
| Brilliant.org | Interactive problem-solving and challenges to enhance logical thinking and math skills. |
2. Practice Books and Guides
Books designed specifically for advanced math preparation offer structured approaches to review and practice. Many include detailed solutions, step-by-step methods, and helpful strategies for tackling complex problems. Using a combination of books and online platforms ensures a well-rounded preparation strategy.
- AP Calculus Prep: A focused guide that includes multiple practice tests and solutions.
- Barron’s AP Calculus: A comprehensive resource known for its thorough explanations and tips for success.
- 5 Steps to a 5: Offers structured review, practice tests, and expert strategies for high performance.
By leveraging these additional resources, you can build a more complete understanding of advanced mathematical concepts, improving both your skills and confidence for any assessment.
Preparing for Future AP Calculus Exams
Success in advanced mathematics assessments requires a strategic approach to preparation, focusing on strengthening key skills, mastering content, and developing test-taking strategies. By adopting effective study habits and utilizing a variety of resources, students can set themselves up for long-term success in future assessments.
1. Consistent Practice and Review

One of the most important aspects of preparing for future assessments is continuous practice. Regularly solving problems, reviewing key concepts, and working through challenging topics will help solidify your understanding and increase familiarity with different question types.
- Daily Practice: Set aside time each day for problem-solving to reinforce concepts.
- Focused Reviews: Revisit areas of weakness periodically to ensure comprehensive understanding.
- Mock Tests: Simulate test conditions with timed practice tests to gauge progress and improve speed.
2. Utilize a Variety of Study Materials
Diverse study materials can provide additional perspectives on complex topics and expose you to a wider range of problems. Combining textbooks, online resources, and practice guides will help you better understand the material and prepare for the unique challenges of each assessment.
- Online Resources: Platforms like Khan Academy, Coursera, and Brilliant offer interactive lessons and problem sets.
- Study Guides: Books such as Barron’s or Princeton Review provide in-depth explanations and practice problems.
- Study Groups: Collaborating with peers can offer new insights and help reinforce learning through discussion and shared problem-solving.
By employing a well-rounded approach to studying and committing to consistent practice, students can build a solid foundation for future success in advanced mathematics assessments.