
Mastering foundational concepts in mathematics is essential for building a strong understanding of more advanced topics. In this section, we will cover important principles, equations, and techniques necessary for solving geometric problems. Whether you’re preparing for an exam or simply strengthening your grasp of core ideas, a solid foundation is crucial for success.
We will explore various methods to tackle common challenges, from identifying shapes to applying formulas. Each step of the process will be broken down to ensure clarity and facilitate easier comprehension. With the right approach and practice, mastering these topics becomes a manageable and rewarding task.
Key topics such as shapes, angles, and basic algebraic operations will be reviewed with a focus on understanding their applications and solving problems effectively. By the end of this guide, you’ll be better prepared to approach similar exercises with confidence and accuracy.
Geometry Unit 1 Review Answers
In this section, we will focus on reinforcing the core concepts covered in the first part of the course. By breaking down essential topics and providing step-by-step instructions, you’ll gain the skills needed to approach related problems with confidence. The goal is to understand the underlying principles, not just memorize formulas.
We will address the following areas to ensure a comprehensive grasp of the material:
- Basic Shape Identification: Recognizing geometric figures and their properties.
- Key Formulas and Theorems: Understanding essential formulas for calculating areas, perimeters, and angles.
- Problem-Solving Techniques: Step-by-step methods for approaching and solving problems.
- Common Misunderstandings: Identifying and correcting frequent mistakes students encounter.
For each topic, we will explain the necessary steps and provide examples to guide you through the process. By the end of this section, you’ll be able to solve a variety of related questions efficiently and accurately.
Let’s begin by exploring the most common types of problems students face in this section, along with their solutions:
- Identifying Angles: Understanding how to classify and measure different angles.
- Working with Circles: Knowing the formulas for radius, diameter, and circumference.
- Applying Pythagoras’ Theorem: Using this fundamental theorem to solve for unknown sides in right triangles.
As you progress, practice will help reinforce these concepts and increase your problem-solving efficiency.
Key Concepts in Geometry Unit 1
This section introduces the foundational ideas that form the basis for understanding geometric relationships and problem-solving techniques. Emphasis is placed on recognizing shapes, understanding their properties, and learning the critical formulas used to calculate various measurements. By grasping these concepts, students are better equipped to tackle more complex topics and apply their knowledge effectively.
Essential principles include:
- Shapes and Their Properties: Understanding different types of shapes, such as triangles, quadrilaterals, and circles, and the specific characteristics that define them.
- Measuring Angles: Techniques for calculating and identifying various types of angles in geometric figures.
- Perimeter and Area: Applying formulas to calculate the boundary and surface area of common shapes.
- The Pythagorean Theorem: Using this theorem to solve problems involving right-angled triangles.
- Basic Algebraic Techniques: Understanding how algebra is applied to solve geometric equations.
These concepts serve as the building blocks for more advanced topics and help students develop the critical thinking skills necessary for solving geometric problems in practical scenarios.
Understanding Basic Geometric Shapes
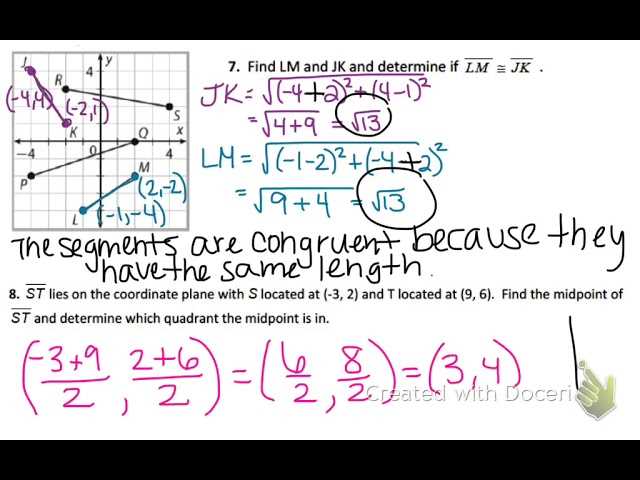
In this section, we will explore the fundamental shapes that form the foundation of geometric study. These shapes have distinct properties and are the building blocks for more complex figures. By recognizing their characteristics and understanding how they relate to each other, you will be able to solve a wide variety of geometric problems more effectively.
Triangles and Their Types
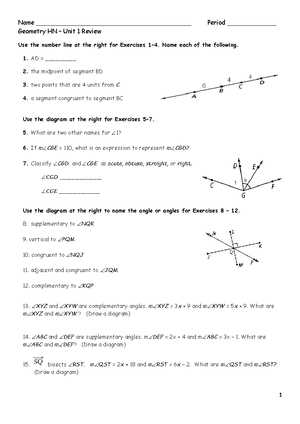
Triangles are one of the simplest yet most important shapes. They are defined by three sides and three angles. Understanding the different types of triangles, such as equilateral, isosceles, and scalene, is key to mastering geometric problem-solving. Each type has unique properties that make it easier to solve certain kinds of problems, especially when working with angles and side lengths.
Quadrilaterals and Their Properties
Quadrilaterals are four-sided polygons with varied characteristics depending on their specific type. Common quadrilaterals include squares, rectangles, trapezoids, and parallelograms. Recognizing the properties of these shapes, such as the relationship between their sides and angles, is essential for solving related questions about area, perimeter, and symmetry.
Essential Geometry Formulas to Know
Understanding key mathematical formulas is crucial for solving a wide variety of problems. These formulas allow you to calculate areas, perimeters, angles, and other important measurements in geometric figures. Mastering them helps streamline the problem-solving process and ensures you approach each problem with the right tools at hand.
Some of the most important formulas to be familiar with include:
- Area of a Triangle: Area = ½ × base × height
- Area of a Rectangle: Area = length × width
- Perimeter of a Square: Perimeter = 4 × side
- Area of a Circle: Area = π × radius²
- Circumference of a Circle: Circumference = 2 × π × radius
- Pythagorean Theorem: a² + b² = c² (for right-angled triangles)
By practicing these formulas and applying them to various scenarios, you will be better prepared to solve geometric problems accurately and efficiently.
Common Mistakes in Unit 1 Review
When studying fundamental concepts in this field, many students make recurring errors that can hinder their progress. Recognizing and avoiding these mistakes is crucial for improving accuracy and understanding. By identifying common pitfalls, you can refine your problem-solving strategies and enhance your performance in future exercises.
Below are some of the most frequent mistakes that students encounter during this section:
| Mistake | Explanation | Solution |
|---|---|---|
| Confusing the formulas for area and perimeter | Students often mix up the formulas for calculating area and perimeter, leading to incorrect results. | Always check whether you’re calculating the boundary (perimeter) or the surface area (area) of the shape. |
| Incorrectly applying the Pythagorean Theorem | Sometimes students use the Pythagorean Theorem when the triangle is not a right-angled triangle. | Ensure that the triangle has a right angle (90 degrees) before applying this theorem. |
| Overlooking units of measurement | Failing to account for units can lead to incorrect answers, especially when converting between different measurement types. | Always ensure that units are consistent across the problem and convert when necessary. |
| Forgetting to simplify fractions or expressions | In some cases, students forget to simplify their final answers, leaving them in more complex forms. | Double-check calculations to make sure all expressions are simplified to their simplest form. |
By being aware of these mistakes and taking the necessary steps to avoid them, you can significantly improve your understanding and accuracy in solving related problems.
Step-by-Step Geometry Problem Solving
Solving mathematical problems efficiently requires a structured approach that breaks down complex tasks into smaller, manageable steps. By following a clear problem-solving strategy, you can approach each question methodically, ensuring no key information is overlooked and that all necessary calculations are performed correctly. This process helps build confidence and reduces the chances of making errors along the way.
Understanding the Problem
The first step is to carefully read the problem and identify what is being asked. Pay close attention to any given data, such as side lengths, angles, or area measurements. It’s crucial to understand the relationship between different elements in the problem and how they contribute to finding the solution.
Applying the Right Approach
Once you understand the problem, the next step is to select the appropriate method or formula to use. Whether you’re dealing with angles, lengths, or areas, make sure you’re using the correct tools for the task. For example, if you’re working with right triangles, the Pythagorean theorem is often the most effective method. If you’re calculating areas, be sure to use the correct formula for the given shape.
Breaking down the problem into smaller, more manageable steps ensures that each aspect of the problem is addressed carefully, making the overall solution process more efficient and accurate.
Tips for Mastering Geometry Theorems
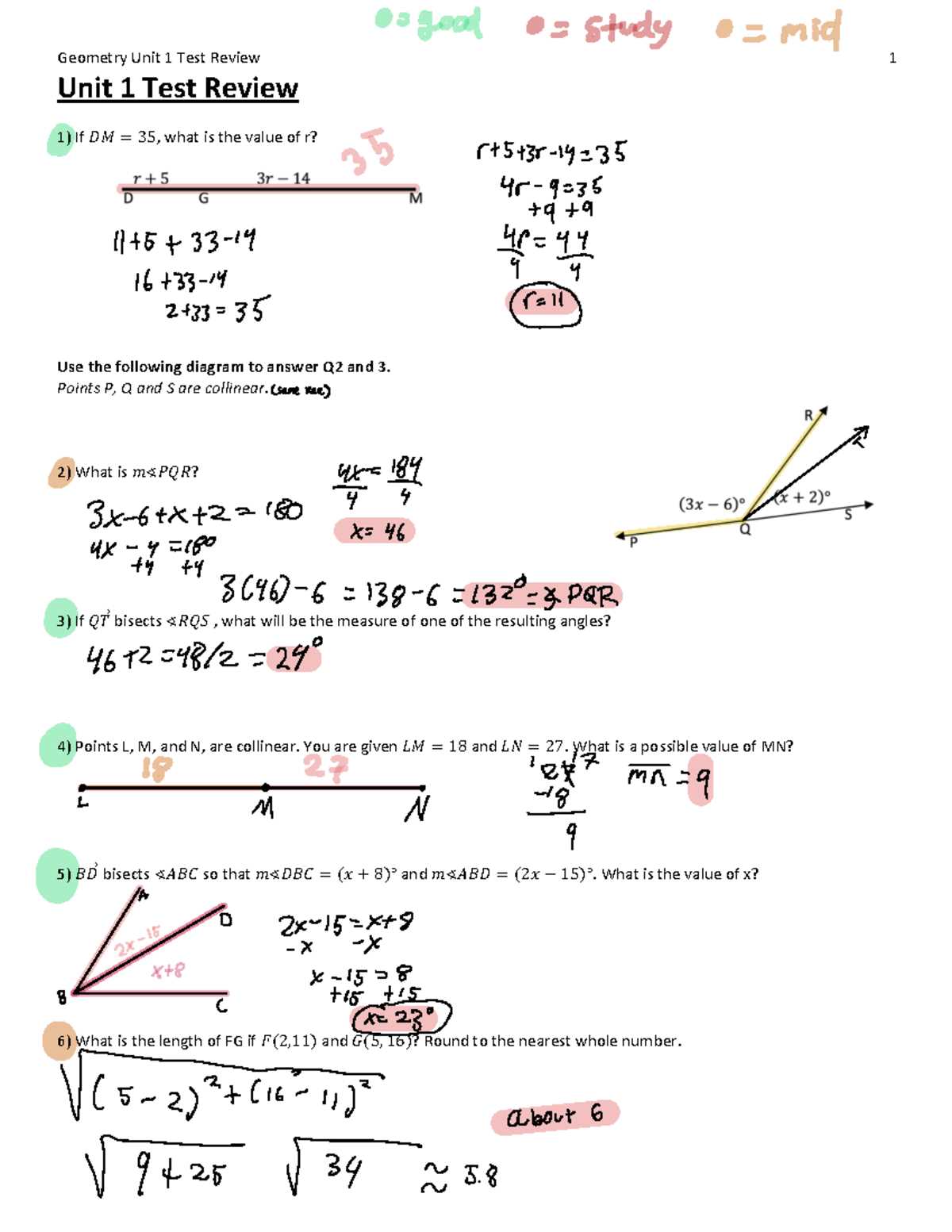
Mastering theorems is essential for solving problems effectively. These principles provide a foundation for understanding the relationships between different shapes, angles, and measurements. Grasping these rules requires practice, familiarity with their applications, and a strategic approach to applying them in various situations. By following a few helpful strategies, you can strengthen your understanding and improve your problem-solving skills.
Here are some key tips for mastering theorems:
| Tip | Description |
|---|---|
| Understand the Proof | Rather than just memorizing theorems, take the time to understand why they work. Knowing the reasoning behind each theorem helps you apply it more effectively. |
| Practice Regularly | Frequent practice is essential. The more problems you solve, the more familiar you become with the different theorems and how to apply them correctly. |
| Break Down Complex Theorems | Some theorems can seem overwhelming at first. Break them down into smaller steps to understand each component and how it contributes to the solution. |
| Visualize the Problem | Drawing diagrams or creating visual representations can help you better understand the relationships between elements in a theorem and how to apply it. |
| Make Connections | Look for patterns or connections between theorems. Many theorems are interrelated, and recognizing these relationships can help simplify complex problems. |
By incorporating these strategies into your study routine, you will gain a deeper understanding of theorems and be better prepared to tackle more advanced problems.
How to Approach Geometry Equations
When solving equations in this field, a methodical approach is key to ensuring accurate and efficient results. Whether you’re working with unknown variables, area formulas, or complex shapes, understanding the basic principles behind each equation is essential. Breaking down the process into smaller steps allows for a clearer path to the solution and minimizes the chance of making errors.
Step-by-Step Process
Follow these steps to approach each equation methodically:
- Read the problem carefully: Understand what is being asked and identify the given information.
- Choose the correct formula: Ensure you’re using the right equation for the specific problem, whether it’s for area, perimeter, or angles.
- Substitute values: Insert the known values into the equation, paying attention to units and conversions if needed.
- Solve for the unknown: Perform the necessary algebraic operations to find the unknown variable.
- Double-check your work: Verify the solution by substituting it back into the original equation to ensure it satisfies the given conditions.
Common Mistakes to Avoid
When working with equations, it’s easy to make a few common mistakes. Here are some to watch out for:
- Forgetting to simplify: Always simplify expressions fully to avoid unnecessary complexity in your calculations.
- Ignoring units: Be sure to pay attention to units of measurement and convert when needed to maintain consistency.
- Incorrect substitution: Carefully check that you’re plugging the correct values into the right parts of the equation.
- Skipping steps: Don’t rush through the problem. Missing intermediate steps can lead to errors in the final solution.
By following these steps and avoiding common mistakes, you can approach geometry equations with confidence and accuracy.
Reviewing Key Definitions in Geometry
Understanding fundamental terms is essential for solving problems effectively. These key concepts form the building blocks for more complex ideas and are crucial for interpreting relationships between various elements. Having a clear grasp of these definitions will enhance your ability to approach and solve problems with confidence.
Here are some of the key terms to review:
- Point: A location in space with no size or dimension. It is usually represented by a dot and labeled with a capital letter.
- Line: A one-dimensional figure that extends infinitely in both directions. It has no thickness and is typically represented by two points on the line with an arrowhead at both ends.
- Angle: The space between two intersecting lines or rays. It is measured in degrees and can be classified as acute, right, obtuse, or straight.
- Circle: A shape where all points are equidistant from a central point. The distance from the center to any point on the edge is called the radius.
- Perimeter: The total distance around the boundary of a shape. It is calculated by adding the lengths of all the sides.
- Area: The measure of the surface within the boundaries of a shape. It is expressed in square units and is calculated using specific formulas for different shapes.
By revisiting these definitions regularly, you will reinforce your foundational knowledge and better understand how these concepts interact in various problems.
Geometry Unit 1 Practice Problems
Practicing with various problems is one of the best ways to strengthen your understanding of key concepts. By working through different scenarios and applying the principles you’ve learned, you develop the skills needed to solve more complex tasks. This section provides a series of problems that will help reinforce your knowledge and improve problem-solving strategies.
Problem 1: Find the Perimeter of a Rectangle
A rectangle has a length of 8 units and a width of 5 units. Calculate the perimeter of the rectangle.
Solution: The formula for the perimeter of a rectangle is P = 2(l + w), where l is the length and w is the width. Substitute the values:
P = 2(8 + 5) = 2(13) = 26
Answer: The perimeter is 26 units.
Problem 2: Calculate the Area of a Triangle
A triangle has a base of 10 units and a height of 6 units. Find its area.
Solution: The formula for the area of a triangle is A = 1/2 × base × height. Substitute the given values:
A = 1/2 × 10 × 6 = 30
Answer: The area is 30 square units.
By working through these practice problems, you can better understand how to apply the formulas and strategies that are crucial for solving problems in this subject.
Identifying Geometry Postulates
Understanding fundamental principles is essential for building a solid foundation in any subject. Postulates are basic assumptions or statements that are accepted as true without proof and serve as the starting points for more complex reasoning and theorems. Recognizing these postulates is crucial, as they help establish the framework within which more detailed concepts can be explored and applied.
Here are some key postulates commonly encountered in this field:
- Through any two points, there is exactly one line: This postulate states that a unique line can be drawn connecting two distinct points, no matter where those points are located.
- A line can be extended indefinitely in both directions: Once a line is drawn, it can be extended infinitely in both directions, maintaining its straightness and direction.
- All right angles are congruent: This postulate asserts that all right angles, by definition, are of equal measure (90 degrees), regardless of the situation.
- Through any three non-collinear points, there is exactly one plane: If three points do not lie on the same straight line, they define a unique flat surface, known as a plane.
- If two points lie in a plane, the line connecting them lies in that plane: This postulate states that if two points are within the same plane, the straight line connecting them will also lie within that plane.
Mastering the identification and application of these postulates is vital for developing a deeper understanding of relationships in space and laying the groundwork for solving more complex problems in the subject.
Proof Strategies and Techniques
Developing effective strategies for proving statements is a cornerstone of understanding logical reasoning in mathematics. Proofs require a structured approach that builds upon known facts, definitions, and postulates to demonstrate the truth of a given statement. Mastering these techniques will allow you to confidently tackle complex problems and establish logical conclusions from assumptions.
Direct Proof
A direct proof is a straightforward method where the statement is proven by logical deductions from accepted facts. In this approach, you start with known premises and apply logical steps to arrive at the desired conclusion. This method is often used when there is a clear path from hypothesis to conclusion.
Proof by Contradiction
Proof by contradiction works by assuming the opposite of what you are trying to prove. If the assumption leads to an impossibility or contradiction, then the original statement must be true. This technique is particularly useful when a direct approach is difficult or when proving the impossibility of a certain scenario.
By understanding these core techniques, you can begin to approach proofs with more confidence and efficiency, building your ability to logically connect ideas and solve complex problems.
Understanding Coordinate Geometry Basics
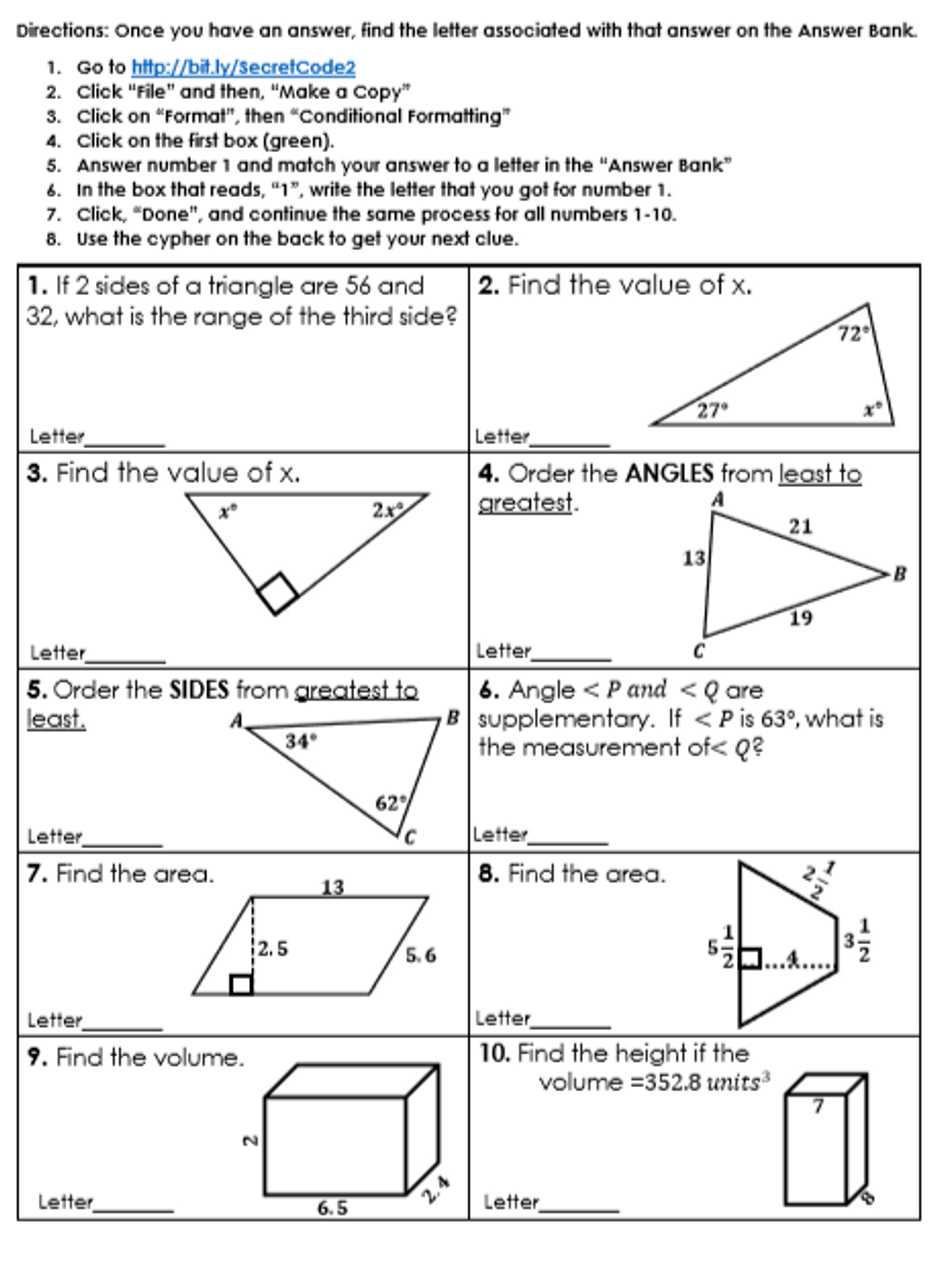
Coordinate-based methods offer a powerful way to analyze relationships between points, lines, and shapes in a two-dimensional space. By using a grid system where each point is defined by its location relative to two perpendicular axes, you can derive distances, slopes, and equations that describe various geometric objects. This approach simplifies many problems and provides a concrete way to solve them using algebraic methods.
Working with the Coordinate Plane
The coordinate plane consists of two perpendicular axes: the horizontal axis (x-axis) and the vertical axis (y-axis). The point where these axes intersect is known as the origin, marked as (0, 0). Every other point in the plane is defined by a pair of numbers, called coordinates, which represent its horizontal and vertical distances from the origin. The first number indicates the horizontal distance (left or right), and the second number indicates the vertical distance (up or down).
Key Concepts: Distance and Slope
Two important concepts in coordinate-based geometry are distance and slope. The distance between two points can be found using the distance formula, which is derived from the Pythagorean theorem. The slope of a line represents its steepness and is calculated by dividing the change in the y-coordinates by the change in the x-coordinates between two points on the line. These basic tools allow you to describe and analyze geometric relationships on the coordinate plane.
With these fundamental principles, you can tackle a wide variety of problems that involve locations and relationships between points, helping you gain a deeper understanding of space and structure.
Real-World Applications of Geometry
The concepts and principles that are studied in mathematical spaces extend far beyond theoretical exercises. They play a critical role in various industries and everyday activities. From architecture to technology, the ability to analyze shapes, sizes, and spatial relationships is essential for solving real-world challenges. Understanding these principles allows professionals to make informed decisions, design functional structures, and optimize spaces.
Applications in Architecture and Design

In the field of architecture, the knowledge of spatial relationships is crucial. Architects use these principles to design buildings that are not only aesthetically pleasing but also structurally sound. Some key areas where geometry plays a role include:
- Creating blueprints and floor plans that ensure efficient use of space.
- Designing structures that can withstand various forces (e.g., gravity, wind, earthquakes).
- Optimizing the placement of windows, doors, and rooms for natural light and airflow.
Technology and Engineering Applications
In the world of technology and engineering, spatial reasoning is at the heart of many innovations. Whether it’s the design of computer graphics, the creation of video games, or the development of machinery, understanding the relationships between points, lines, and surfaces is essential. Some examples include:
- Modeling 3D objects in software for product design.
- Building algorithms for computer simulations and animations.
- Designing efficient transportation systems, from road networks to public transit.
These practical applications show how mathematical principles are used to shape the world around us, ensuring that everything from the homes we live in to the technology we rely on is designed efficiently and effectively.
Strategies for Geometry Test Success
Achieving success in spatial reasoning tests requires more than just memorizing formulas and theorems. It involves a deep understanding of key concepts, the ability to apply them in different scenarios, and effective test-taking strategies. By approaching each problem with a clear strategy, students can improve their performance and feel more confident during assessments.
Here are some practical approaches to help you succeed in your next assessment:
- Practice Regularly: Consistent practice is essential. Work through a variety of problems to build familiarity with different types of questions and increase your problem-solving speed.
- Understand Key Concepts: Focus on mastering the core principles, such as relationships between points, lines, angles, and shapes. Strong foundational knowledge will make complex problems easier to solve.
- Break Down Problems: When facing a challenging question, break it into smaller, more manageable parts. Identify known values, key relationships, and what you are asked to find.
- Check Your Work: If time allows, review your answers to ensure no calculation errors have been made and that all steps are properly followed.
- Use Visual Aids: Draw diagrams, label important elements, and use tools such as a ruler or protractor to help visualize the problem and find solutions more effectively.
By applying these techniques and preparing thoughtfully, you can enhance your ability to perform well and approach each test with confidence.
Geometry Unit 1 Commonly Asked Questions
When preparing for tests and assignments, many students have similar concerns and questions about the concepts covered. Understanding common queries can help clarify difficult topics and ensure that key principles are well understood. Below are some frequently asked questions that may assist in clearing up any confusion as you review.
Frequently Asked Questions
| Question | Answer |
|---|---|
| What is the best way to memorize key definitions? | Start by writing them out and using them in context. Create flashcards or diagrams to help visualize the concepts. Regularly review these definitions to reinforce memory. |
| How do I know which formula to use in a problem? | Identify the elements in the problem (such as angles, lines, or shapes) and match them to the corresponding formula. Pay close attention to keywords like “area,” “perimeter,” or “volume” that guide the choice. |
| What should I do if I get stuck on a question? | Take a deep breath and break the problem into smaller steps. Recheck the information you have, draw diagrams if needed, and look for any known relationships between elements. |
| How can I improve my problem-solving speed? | Practice regularly and time yourself during practice tests. Familiarity with different problem types and strategies will allow you to solve questions faster and with more confidence. |
| What resources can I use to supplement my learning? | Utilize online tutorials, study guides, and practice problem sets. Work with peers or seek help from a tutor if needed to clarify concepts that are particularly challenging. |
By reviewing these common questions and applying the tips provided, you’ll be better prepared to tackle any difficulties you encounter during your studies.
Mastering Vocabulary for Unit 1
Understanding the language of mathematics is crucial to mastering any topic. In this section, we will focus on key terms and concepts that will help you navigate through the material. Being comfortable with the vocabulary enables you to communicate ideas clearly, solve problems more efficiently, and understand the connections between different concepts.
Key Terms to Remember
Here are some essential terms that form the foundation of the subject in the first section:
- Point: A location in space with no size, usually represented by a dot and labeled with a capital letter.
- Line: A straight path that extends infinitely in both directions. It has length but no thickness.
- Segment: A part of a line with two endpoints. Unlike a line, a segment has a definite length.
- Angle: A figure formed by two rays with a common endpoint called the vertex.
- Parallel Lines: Lines in the same plane that never intersect, no matter how far they are extended.
Effective Strategies for Vocabulary Mastery
Mastering the terminology is a step-by-step process. Here are some strategies to help retain key terms:
- Use Flashcards: Write down terms and their definitions on flashcards. Quiz yourself regularly to reinforce the information.
- Group Related Terms: Group similar terms together to understand their relationships. For instance, “ray,” “line,” and “segment” are all different ways to describe parts of a straight path.
- Practice with Visuals: Draw diagrams to visually connect the terms with their real-life representations.
- Teach Someone Else: Teaching someone else is a great way to ensure you understand the terminology yourself.
By mastering these terms and practicing their usage, you’ll be well-equipped to handle more complex problems as you progress through the material.