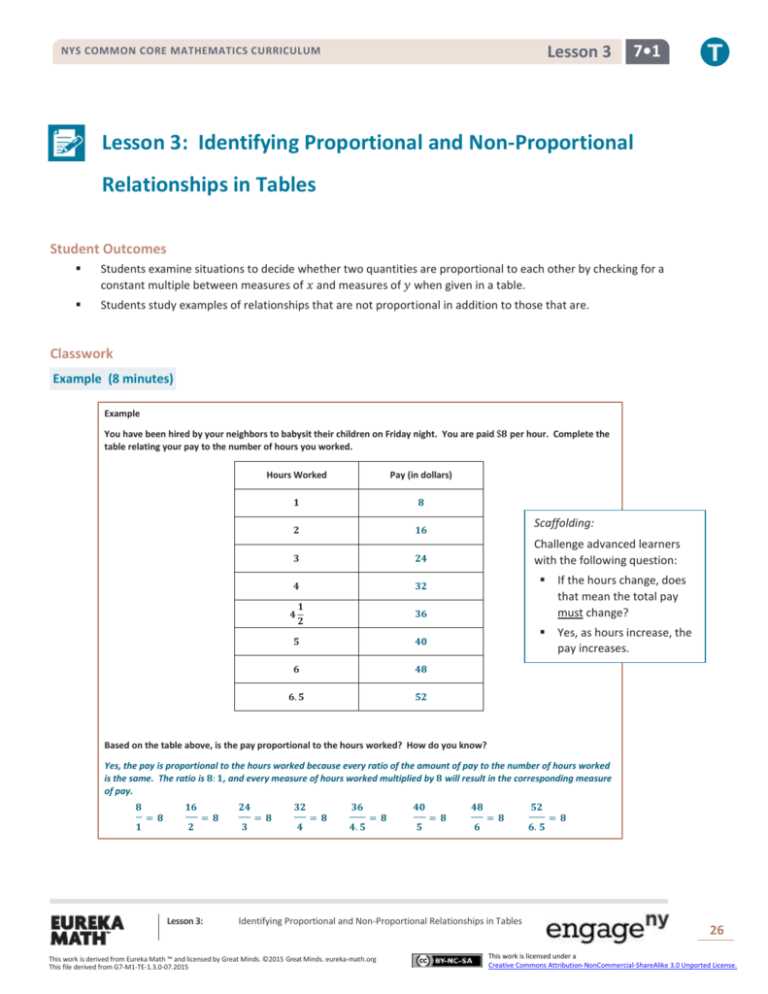
In this section, we will explore essential solutions and strategies for successfully navigating the challenges of the third unit in the curriculum. The goal is to provide students with the necessary support to grasp the core principles and develop problem-solving skills. Whether it’s tackling exercises or understanding theoretical concepts, this guide aims to break down complex topics into manageable steps.
Students and educators alike can benefit from reviewing these explanations, as they highlight the most effective ways to approach assignments. By examining detailed examples and following practical steps, learners can improve their comprehension and confidence in tackling similar problems.
The objective is not only to provide direct solutions but also to enhance understanding through systematic breakdowns. With the right approach, even the most challenging exercises can be simplified, leading to greater academic success. This resource is designed to support learners in mastering the material and excelling in their studies.
Eureka Math Grade 7 Module 3 Answer Key
This section provides a comprehensive guide to the solutions for the third set of lessons in the curriculum. It aims to assist students in understanding the correct methods for solving problems, while also offering a deeper explanation of how to approach various types of exercises. The goal is to help learners build confidence and master the concepts necessary for success.
Overview of Key Concepts
Understanding the core principles covered in this unit is crucial for solving the exercises efficiently. Below are some of the major areas that students should focus on:
- Solving algebraic expressions
- Identifying patterns and relationships
- Working with ratios and proportions
- Understanding geometric concepts
- Analyzing data and graphs
Step-by-Step Solutions
For each problem set, we provide clear, step-by-step explanations that break down the process into manageable parts. Here’s how to approach typical problems:
- Read the problem carefully to identify what is being asked.
- Determine the best method to solve the problem based on the concepts learned.
- Break the problem down into smaller steps to avoid confusion.
- Double-check calculations and ensure the answer makes sense.
By following these steps, students can improve their problem-solving skills and enhance their understanding of the material. Each solution aims to clarify common challenges, providing a path to better comprehension.
Overview of Module 3 Topics
This section covers the core themes and areas of study for the third unit of the curriculum. The primary focus is on developing a strong understanding of key concepts, which are essential for progressing in more advanced topics. Students will encounter a variety of exercises that help to reinforce these ideas, with an emphasis on real-world applications and problem-solving strategies.
The unit introduces several important concepts, each building on the previous one, ensuring that students grasp the fundamental principles required to solve increasingly complex problems. Below is a breakdown of the main topics covered:
| Topic | Description |
|---|---|
| Ratios and Proportions | Understanding the relationship between different quantities and how they can be compared using ratios and proportional reasoning. |
| Expressions and Equations | Working with algebraic expressions and understanding how to solve equations through various methods. |
| Linear Relationships | Exploring how two variables are connected in a linear pattern, and using graphs to represent these relationships. |
| Geometric Figures | Identifying and working with various geometric shapes, focusing on properties and measurements like area, perimeter, and volume. |
| Data Analysis | Analyzing sets of data to recognize trends, make predictions, and interpret statistical information. |
Each of these topics forms a vital part of the unit, offering students the tools and knowledge they need to solve both abstract and practical problems. By mastering these concepts, learners will be better equipped to handle future lessons and apply their skills in real-world situations.
Understanding Key Concepts in Module 3
In this section, we dive into the essential principles that form the foundation of the third unit. The goal is to ensure that students fully grasp these critical ideas, which are vital for solving a wide range of problems. Each concept builds upon the last, helping learners develop the necessary skills to approach more complex challenges with confidence.
Working with Ratios and Proportions
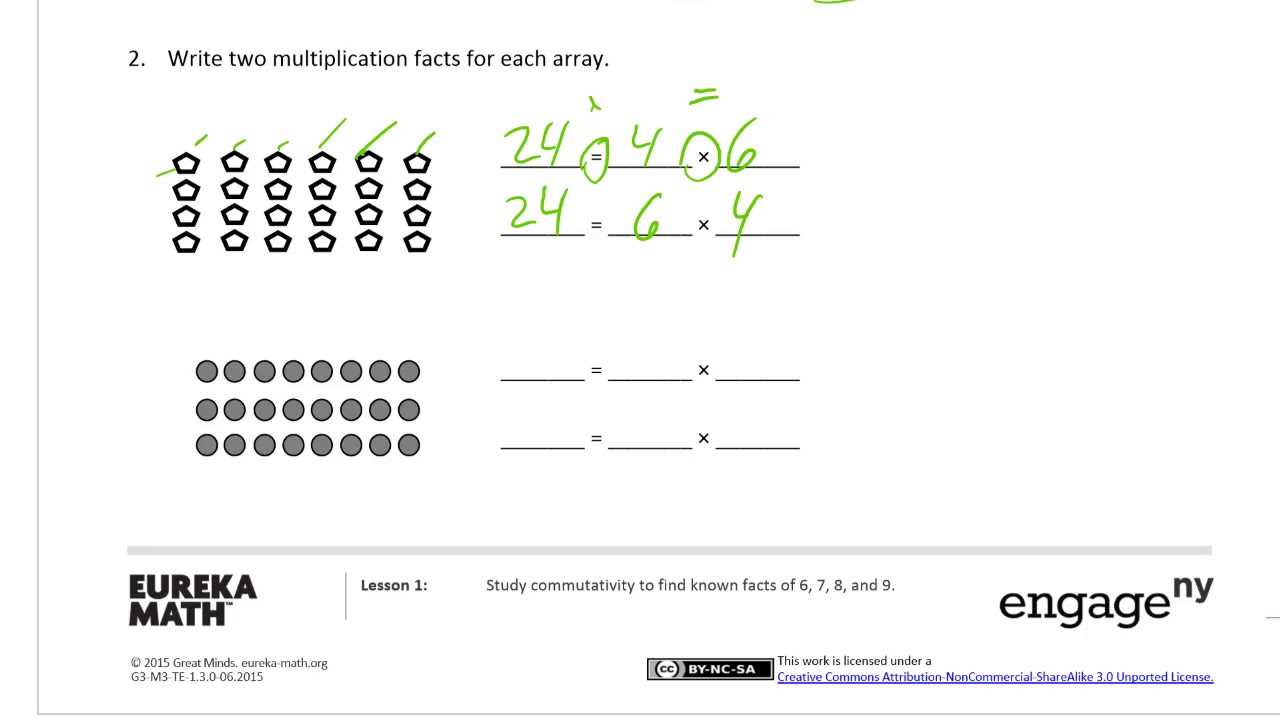
One of the primary focuses of this unit is understanding how quantities relate to one another. Ratios and proportions help students compare values, solve real-world problems, and model various situations. This concept is key to understanding many mathematical relationships and can be applied across multiple disciplines, from science to economics.
Exploring Algebraic Expressions and Equations
Another crucial topic in this unit is working with algebraic expressions and equations. Students will learn how to manipulate variables, solve for unknowns, and interpret relationships between numbers. Mastering these skills lays the groundwork for more advanced topics in algebra and beyond.
By thoroughly understanding these key concepts, students will be able to approach exercises with a clearer perspective, making it easier to solve problems and apply their knowledge in practical ways.
Step-by-Step Solutions for Each Problem
Breaking down problems into manageable steps is essential for achieving clarity and understanding. In this section, we will provide detailed solutions to each exercise, guiding you through the process from start to finish. By following these steps, learners can approach complex problems with confidence and accuracy.
Approaching Algebraic Challenges
When solving algebraic problems, it’s important to identify the unknowns and use the appropriate methods to isolate them. Begin by simplifying both sides of the equation and applying operations systematically to find the solution. Keep in mind that every step plays a crucial role in arriving at the correct result.
Solving Proportions and Ratios
In problems involving proportions, the goal is to determine the relationship between two sets of values. Start by setting up a proportion and cross-multiply to solve for the unknown. Double-check your calculations to ensure accuracy before finalizing the solution.
Each solution is presented with clear instructions to help students understand the underlying process. By practicing these step-by-step methods, learners will improve their problem-solving skills and become more proficient in tackling similar exercises in the future.
How to Use the Answer Key Effectively
Using a solution guide can be an invaluable resource for mastering new concepts. However, it is important to approach it strategically to gain the most benefit. Simply looking at the final results without understanding the process may not lead to meaningful learning. Instead, the solution guide should be used as a tool to enhance problem-solving skills and reinforce key concepts.
To use the solution guide effectively, follow these steps:
| Step | Action | Benefit |
|---|---|---|
| 1 | Attempt the problems first | Helps identify areas of difficulty and strengthens understanding |
| 2 | Review the solution process | Clarifies the reasoning and methods used to solve the problem |
| 3 | Compare your approach | Reveals any errors or alternative methods to improve problem-solving |
| 4 | Reattempt similar problems | Reinforces learning and builds confidence |
By following this approach, you not only find the correct solutions but also develop a deeper understanding of the concepts behind the problems. This way, the guide becomes a tool for active learning rather than just a quick fix for homework. Remember, the goal is to master the material and apply the skills learned in future tasks.
Common Mistakes and How to Avoid Them
When solving problems in any subject, it’s easy to make errors, especially when dealing with complex exercises. Recognizing common pitfalls and learning how to avoid them is a key step toward improving problem-solving abilities. In this section, we will highlight some frequent mistakes students make and provide tips on how to steer clear of them.
Misinterpreting the Problem

One of the most common mistakes is misunderstanding the question. It is important to read the problem carefully and identify what is being asked before beginning the solution. Often, students rush through this step and end up solving for the wrong variable or focusing on the wrong aspect of the problem.
- Tip: Take a moment to rephrase the question in your own words. This will help clarify what is being asked.
- Tip: Highlight key information in the problem to ensure nothing is overlooked.
Forgetting to Check Work
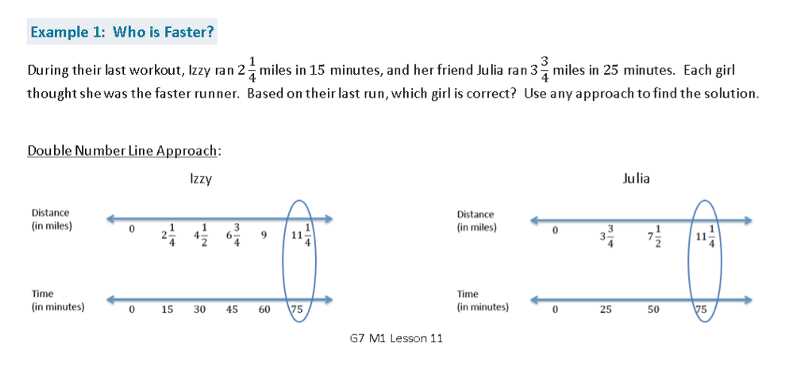
Another common mistake is failing to check the solution after completing the problem. While it’s easy to assume that the answer is correct, overlooking simple mistakes in calculations or logic can lead to errors that affect the entire process.
- Tip: Always review your steps before finalizing the solution. Double-check any calculations and ensure that the solution makes sense in the context of the problem.
- Tip: If time allows, solve the problem a second time to confirm the result.
By being aware of these common mistakes and following the tips provided, students can improve their problem-solving techniques and avoid unnecessary errors, leading to more accurate and efficient solutions.
Reviewing Practice Problems from Module 3
Regularly revisiting practice exercises is an essential step in reinforcing the concepts learned. By reviewing past problems, students can strengthen their understanding and identify areas where they might need further clarification. This process helps solidify the knowledge gained and ensures greater retention of key principles.
To make the most of this review, focus on understanding each step of the solution, not just the final result. Analyzing how each problem is approached allows learners to see how concepts are applied in different contexts. Additionally, reviewing mistakes is just as important as reviewing correct answers, as it provides insight into common errors and helps refine problem-solving strategies.
As you go through each exercise again, take note of any areas where you feel uncertain or need additional practice. Use these as opportunities to reinforce weak points and further develop your skills. By consistently practicing and reviewing, you’ll build confidence in applying the learned techniques to new challenges.
Key Strategies for Solving Module 3 Exercises
To solve exercises effectively, it’s crucial to adopt the right strategies. Developing a systematic approach not only makes solving problems more manageable but also enhances understanding of core concepts. By following specific techniques and strategies, students can tackle complex exercises with greater confidence and accuracy.
Breaking Down the Problem
The first step in solving any problem is to break it down into smaller, more manageable parts. Start by identifying what is being asked and highlighting the important information. This step ensures that no key details are overlooked and gives you a clear path to follow as you work through the problem.
- Tip: Draw diagrams or write equations to visualize the relationships between variables.
- Tip: Identify known and unknown values to create a structured approach.
Applying Logical Steps
Once the problem is understood, the next step is to apply the correct methods in a logical order. Whether it’s solving for an unknown variable or simplifying an equation, following a step-by-step process ensures that no mistakes are made along the way. Each action should build on the previous one to arrive at the correct solution.
- Tip: Work through each step methodically, checking each calculation as you go.
- Tip: If necessary, reverse-engineer the problem by testing the solution to ensure its validity.
By using these strategies, students can enhance their problem-solving abilities and approach exercises with a clear and focused mindset. These methods not only improve accuracy but also help build a stronger foundation for tackling future challenges.
How Module 3 Prepares Students for Advanced Topics
Building a solid foundation is crucial for success in more complex subjects. The exercises in this section are designed to help students strengthen their understanding of core principles, ensuring that they are well-equipped to tackle more advanced topics in the future. By mastering the concepts covered here, students gain the skills and confidence needed to approach new challenges with greater ease.
Strengthening Problem-Solving Skills
The key to excelling in advanced topics lies in honing problem-solving abilities. The techniques practiced in this section emphasize logical reasoning, careful analysis, and systematic approaches. These skills not only improve performance in future lessons but also serve as the building blocks for more advanced problem-solving strategies.
- Tip: Develop a habit of breaking down complex problems into smaller steps to make them more manageable.
- Tip: Regular practice of different problem types helps strengthen cognitive flexibility and adaptability.
Building Connections Across Concepts
This section also encourages students to make connections between different topics. By applying previously learned concepts in new contexts, students can see how various mathematical ideas are interrelated. This not only deepens understanding but also prepares them to approach more advanced concepts with a broader perspective.
- Tip: Look for patterns across different exercises and draw comparisons to recognize recurring themes.
- Tip: Reinforce your learning by reviewing related concepts from earlier lessons to understand their application in new situations.
Through these exercises, students gain the necessary skills to tackle more complex topics with confidence. By mastering the foundational principles here, they will be better prepared to excel in future lessons and succeed in advanced academic challenges.
Tips for Parents Helping with Homework
Supporting children with their assignments can be challenging but highly rewarding. As a parent, your role is not to provide the answers, but to guide and encourage your child through the problem-solving process. By offering the right kind of help, you can foster independence and a deeper understanding of the concepts being taught.
Create a Positive Learning Environment
A quiet, comfortable space free from distractions is essential for effective homework time. Establishing a designated study area with all the necessary tools–such as pencils, paper, and a calculator–helps your child focus on their work. It also signals to them that learning is important and that they should dedicate time and effort to it.
- Tip: Make sure the space is well-lit and organized to avoid unnecessary distractions.
- Tip: Keep electronic devices out of reach unless they are needed for the assignment.
Encourage Critical Thinking
Instead of simply providing answers, encourage your child to think through the problem on their own. Ask guiding questions that help them break down the problem into smaller, more manageable parts. This helps them develop critical thinking skills and gives them the confidence to approach future challenges independently.
- Tip: Ask questions like, “What do you think comes next?” or “Can you explain why you chose this method?”
- Tip: Encourage them to review the problem after completing it to see if the solution makes sense.
Be Patient and Offer Praise
Homework can sometimes be frustrating, and it’s important for parents to remain patient. A positive attitude and constant encouragement go a long way in helping children feel motivated. Recognizing their effort and praising their hard work, rather than just the final result, fosters a growth mindset.
- Tip: Praise effort over outcome to help them focus on the process, not just the end result.
- Tip: Break tasks into smaller steps, so they can experience success throughout the process.
By following these tips, parents can provide valuable support without taking over the task. The goal is to help students develop the skills and confidence they need to become independent learners, which will benefit them in the long term.
How Module 3 Connects to Other Modules
The concepts explored in this section play a crucial role in laying the groundwork for topics that will be covered later in the curriculum. The lessons learned here are not isolated; they connect to other areas, creating a more comprehensive understanding of the broader subject. By recognizing these connections, students can see how each piece fits into the bigger picture, enhancing both their comprehension and retention.
Building on Previous Knowledge
The skills developed in this section build on the foundational knowledge from earlier lessons. By revisiting and applying what was learned previously, students can strengthen their understanding and become better prepared for upcoming challenges. Each new concept introduced in this section is linked to those from earlier studies, ensuring a smooth transition as they progress through the curriculum.
- Tip: Review earlier lessons to understand how current topics connect to past knowledge.
- Tip: Use examples from previous lessons to reinforce new concepts.
Preparing for Future Topics

The skills and strategies practiced in this section serve as a foundation for more complex concepts that will be covered in future lessons. By developing a solid grasp of the current material, students are better prepared for upcoming topics that build on the same principles. This continuity ensures that students are always progressing, with each lesson adding to their growing body of knowledge.
- Tip: Identify recurring themes and principles that will be relevant in future lessons.
- Tip: Keep track of how current exercises relate to what is coming next in the curriculum.
By understanding how this section connects to other lessons, students can approach new topics with greater confidence and clarity. Recognizing these links makes learning more meaningful and ensures a smoother educational journey.
Interactive Tools for Eureka Success
In today’s digital age, interactive tools can significantly enhance the learning experience. These resources are designed to engage students, making complex concepts more accessible and enjoyable. By incorporating technology into learning, students can explore concepts at their own pace, receive instant feedback, and deepen their understanding of the material.
Online Practice Platforms
Many online platforms offer a variety of interactive exercises and quizzes that reinforce the lessons taught in class. These tools allow students to work through problems step-by-step, with instant feedback helping them identify areas where they may need more practice. The ability to track progress over time also helps students stay motivated and focused on their learning goals.
- Tip: Use platforms that offer adaptive learning paths, so exercises adjust to the student’s skill level.
- Tip: Encourage consistent practice to improve retention and mastery of concepts.
Interactive Whiteboards and Apps
Interactive whiteboards and mobile apps offer a dynamic way to visualize and manipulate problems. Students can interact directly with the content, dragging and dropping objects, solving puzzles, and visualizing complex solutions in real time. These tools provide a hands-on learning experience that is both engaging and educational.
- Tip: Take advantage of apps that allow students to work through problems collaboratively with peers or teachers.
- Tip: Use visual aids such as graphs and charts to better understand abstract concepts.
Video Tutorials and Interactive Lessons
Video tutorials offer step-by-step instructions on how to solve specific types of problems. Interactive lessons, where students can pause, rewind, and revisit material, make learning more flexible and personalized. These tools are especially helpful for reinforcing concepts after a lesson or for preparing for assessments.
- Tip: Select tutorials that break down each step in a clear, easy-to-follow manner.
- Tip: Encourage students to take notes and pause videos at key points to reflect on the material.
By using interactive tools, students can enhance their learning experience, gain deeper insights, and ultimately achieve greater success in mastering the concepts. These resources provide the opportunity for a more engaging, flexible, and effective approach to education.
Guidelines for Assessing Student Progress
Assessing student progress is crucial for understanding their grasp of key concepts and ensuring they are on track to meet learning objectives. A clear and consistent evaluation process not only helps identify areas of strength but also highlights opportunities for improvement. It’s essential to use a variety of assessment methods to get a well-rounded picture of student performance.
Utilize a Combination of Assessment Types
Different types of assessments provide valuable insights into student understanding. By using a mix of formal and informal evaluations, educators can ensure a comprehensive approach to tracking progress.
- Formative Assessments: These ongoing evaluations, such as quizzes and exit tickets, help track daily progress and provide immediate feedback.
- Summative Assessments: These are typically given at the end of a unit or topic to evaluate overall understanding and mastery of the material.
- Performance-Based Assessments: These tasks require students to apply what they’ve learned in real-world scenarios or problem-solving situations.
- Self-Assessments: Encourage students to evaluate their own work and reflect on their learning process, which promotes self-awareness and responsibility.
Focus on Specific Learning Objectives
When assessing progress, it’s important to align evaluations with the specific learning goals for the subject or topic. Focus on key concepts and ensure that assessments address these objectives directly. This way, students are evaluated based on their mastery of the most critical areas.
- Targeted Feedback: Provide feedback that is directly related to the learning goals, helping students understand what they have mastered and what they still need to work on.
- Track Patterns: Regularly review assessments to identify recurring patterns, which may indicate areas of difficulty for a group of students.
Incorporate Multiple Forms of Evidence
In order to have a thorough understanding of a student’s progress, it’s important to gather data from multiple sources. This approach ensures that assessments are not solely reliant on tests or assignments but include a variety of evidence from different activities.
- Class Participation: Observing how actively a student engages in lessons, discussions, and group work can be a key indicator of their understanding.
- Homework and Independent Work: Regularly reviewing homework assignments and classwork provides insight into a student’s ability to apply concepts independently.
- Peer and Teacher Feedback: Input from peers and teachers can offer different perspectives on a student’s progress and areas for improvement.
Use Data to Adjust Instruction
Regular assessments provide valuable data that can help guide instructional decisions. If a student is struggling, adjustments in teaching methods or additional practice opportunities may be needed. By continuously assessing progress, educators can tailor their approach to meet the unique needs of each student.
- Group Instruction: Identify areas where the entire class may need more attention and provide targeted lessons to address these gaps.
- Individualized Support: For students who may be falling behind, consider offering additional one-on-one support or alternative learning strategies.
By following these guidelines, educators can effectively assess student progress, providing the necessary feedback and adjustments to help every student succeed. A holistic approach to evaluation not only ensures mastery of the content but also encourages a deeper, more meaningful learning experience.
How to Approach Word Problems in Module 3
Word problems often present students with complex scenarios that require careful reading and systematic problem-solving techniques. These exercises are designed to test students’ ability to translate real-world situations into mathematical expressions, helping to build critical thinking and analytical skills. Approaching word problems with a step-by-step strategy ensures a more structured and manageable solution process.
Step-by-Step Strategy for Word Problems
To solve word problems effectively, it is important to break them down into smaller, manageable parts. Follow these steps for a clear approach:
- Read the Problem Carefully: Begin by reading the problem multiple times to ensure you understand the context and all the given information.
- Identify Key Information: Highlight or underline important numbers, units, and relationships that are essential for solving the problem.
- Translate the Words into Mathematical Operations: Convert the scenario into an equation or set of operations that reflect the relationships described in the problem.
- Set Up the Equation: Organize the information into a clear mathematical statement, ensuring that all variables are properly defined.
- Solve the Equation: Follow the correct mathematical procedures to solve for the unknowns, ensuring that you perform the operations step by step.
- Check Your Solution: After obtaining an answer, go back to the original problem to verify that the solution makes sense in the given context.
Common Strategies to Enhance Problem-Solving
Besides the basic approach outlined above, students can use various strategies to improve their performance in word problems:
- Draw a Diagram: Visual aids can help students understand the relationships between different elements in the problem.
- Use Estimation: Before solving the problem exactly, try to estimate the answer to get a sense of the solution’s magnitude.
- Work Backwards: If the problem provides the result and asks for the process, reverse engineer the steps to find the correct answer.
- Use a Table: Organizing information in a table format can make it easier to analyze and identify patterns or relationships.
By following a structured approach and incorporating these strategies, students can gain confidence in tackling word problems and develop essential problem-solving skills that are applicable to many different areas of study.
Additional Resources for Learning Support
When working through challenging concepts, students can benefit from a variety of resources designed to reinforce understanding and provide alternative explanations. These materials can help clarify difficult topics, offer practice opportunities, and provide guidance in areas where students may struggle. Utilizing additional support tools can lead to a deeper comprehension of the subject matter, making it easier to grasp complex ideas and improve overall performance.
Helpful Online Platforms and Tools
In today’s digital age, numerous online platforms and tools offer interactive learning experiences, video tutorials, and practice exercises that can supplement classroom instruction. These resources are particularly useful for students who need extra support or prefer learning at their own pace.
- Khan Academy: Provides free instructional videos and practice exercises on a wide range of topics. Its step-by-step lessons are helpful for reviewing difficult concepts.
- IXL: Offers personalized practice questions, tracking progress and providing targeted recommendations based on students’ needs.
- Edpuzzle: Allows teachers to create interactive lessons using videos, with embedded quizzes and assessments to engage students actively.
- Quizlet: An excellent tool for flashcards and quizzes, enabling students to review key concepts and test their knowledge regularly.
Books and Study Guides for Reinforcement
Printed and digital study guides offer structured lessons and practice problems that students can work through outside of the classroom. These resources can provide additional examples and detailed explanations that reinforce the material learned in class.
- Workbooks: Many publishers offer workbooks specifically designed to support learners in different subjects, with practice problems, examples, and solutions.
- Teacher’s Editions: These editions of textbooks often include answers, teaching tips, and suggestions for guiding students through challenging content.
- Study Guides: Comprehensive study guides that summarize essential concepts, providing focused review materials that make it easier to prepare for assessments.
By incorporating these additional resources, students can strengthen their skills, clarify uncertainties, and reinforce their learning, ultimately leading to improved academic success.
Addressing Challenges in Module 3 Lessons
As students progress through new and complex content, they may encounter challenges that can hinder their understanding. These difficulties are common in subjects that require logical thinking and problem-solving skills. Recognizing and addressing these struggles early on is crucial to ensuring students can continue progressing with confidence. By providing targeted support and utilizing effective teaching strategies, many obstacles can be overcome, helping students build both competence and motivation.
Common Struggles in Learning New Concepts
Students often face difficulties when they are introduced to unfamiliar ideas. In particular, concepts that require multi-step problem solving, abstract reasoning, or applying formulas in different contexts can seem overwhelming. The key to overcoming these challenges lies in breaking down the material into smaller, more manageable parts and providing ample opportunities for practice.
- Understanding Word Problems: Many students find it challenging to extract relevant information from word problems. Emphasizing the importance of reading the problem carefully and identifying key terms can help students approach these exercises with greater clarity.
- Multi-step Solutions: Some exercises require students to follow multiple steps in order to arrive at the correct solution. Encouraging students to work through each step methodically and to check their work can reduce mistakes.
- Application of Formulas: Applying formulas in various scenarios can be difficult. Providing visual aids, such as charts or diagrams, can help students see how formulas are used in real-world situations.
Effective Strategies for Overcoming These Obstacles
There are several effective strategies that educators and students can use to address these challenges. By incorporating a variety of approaches, students are more likely to engage with the material and develop a deeper understanding of the concepts.
- Visual Aids: Diagrams, charts, and other visual tools can help students understand complex relationships and better grasp abstract ideas.
- Practice and Repetition: Repetition is a powerful tool in reinforcing learning. Regular practice, especially with varied examples, helps solidify understanding and build confidence.
- Peer Collaboration: Pairing students with peers who have mastered certain concepts can provide additional support and foster a collaborative learning environment.
- Scaffolded Instruction: Gradually introducing new concepts and providing scaffolding (such as hints or partial solutions) can allow students to build their skills step by step without feeling overwhelmed.
By addressing challenges proactively and offering the right tools, students can overcome obstacles and master the skills necessary to succeed in more advanced topics.
Importance of Mastering Module 3 Concepts
Mastering the key concepts presented in this section is essential for students to build a strong foundation for future academic success. The skills learned in this phase not only serve as a stepping stone for more advanced topics but also develop critical thinking and problem-solving abilities. A deep understanding of these principles helps students approach increasingly complex material with confidence, ensuring they are well-prepared for future challenges in their education.
Building a Strong Foundation for Future Learning

As students progress through their education, the concepts introduced in this section become more relevant and are often revisited in higher-level courses. Gaining proficiency in these topics early on enables students to engage with more sophisticated ideas with ease. The mastery of fundamental principles creates a smoother transition to future learning, providing a lasting advantage in both academic and real-world applications.
- Critical Thinking Development: Mastering these concepts encourages students to think critically and solve problems systematically, skills that are valuable across all disciplines.
- Preparation for Advanced Topics: The topics explored lay the groundwork for tackling more complex ideas, ensuring students are ready for upcoming lessons that require a deeper understanding.
- Improved Problem-Solving Skills: By developing a strong grasp of these concepts, students are able to tackle multi-step problems with confidence, enhancing their ability to solve problems effectively.
Confidence and Motivation in Future Challenges
When students have a clear understanding of the material and can apply it with ease, their confidence naturally increases. This confidence motivates them to take on more challenging topics and to persist through difficulties. In turn, this builds a positive learning cycle, where students feel capable of achieving academic success and are more likely to engage actively in their education.
- Increased Engagement: A solid understanding of the material encourages students to become more involved in their learning, leading to better outcomes in the long term.
- Stronger Retention: When students feel confident, they are more likely to retain the information and apply it effectively when needed.
- Resilience in Overcoming Obstacles: Mastery of key concepts equips students with the resilience to face future challenges and to overcome setbacks with perseverance.
By focusing on mastering the concepts in this section, students not only prepare themselves for future academic success but also develop valuable life skills that will benefit them in a variety of contexts.
Final Thoughts on Module 3
As students progress through this section, the importance of understanding the core principles cannot be overstated. The concepts covered are foundational not only for the immediate lessons but also for future academic pursuits. Mastering these topics provides students with the tools to tackle more complex subjects with confidence and clarity. It also reinforces the skills of critical thinking, problem-solving, and perseverance, which are applicable far beyond the classroom.
While the material may present challenges along the way, the key to success lies in a strategic approach and consistent practice. By breaking down problems into manageable steps and understanding the underlying principles, students can overcome obstacles and reach their academic goals. It is essential to maintain a positive attitude and keep track of progress throughout the learning journey, as small victories along the way build a strong foundation for success.
| Key Takeaways | How They Contribute to Success |
|---|---|
| Understanding Core Principles | Allows students to build a solid foundation for more advanced topics and increases overall comprehension. |
| Practice and Persistence | Helps students refine their problem-solving skills and develop the resilience needed to tackle challenging material. |
| Strategic Problem-Solving | Enables students to approach tasks systematically, improving efficiency and accuracy. |
In conclusion, the success of students in this section depends on their commitment to understanding the material, practicing regularly, and applying the strategies learned. By adopting a thoughtful approach and utilizing available resources, students can navigate the complexities of the subject with confidence, ultimately preparing them for success in future academic challenges.