Algebra 1 Final Exam Answer Key for Students

Achieving success in mathematics often requires careful preparation and understanding of the core principles. When it comes to completing important assessments, having the right resources can make a significant difference in performance. This section is dedicated to helping you navigate through the process of reviewing and mastering essential concepts that are typically tested in major math assessments.

Understanding the problems and identifying key techniques are crucial steps in the learning journey. Whether you’re looking to fine-tune your skills or ensure you can tackle various types of questions with confidence, having access to the correct methods can streamline your efforts and boost your performance.

By focusing on the most effective problem-solving strategies and practicing with accurate solutions, you can better prepare for any upcoming math challenges. The aim is to provide clear guidance, allowing you to approach your next test with clarity and precision.

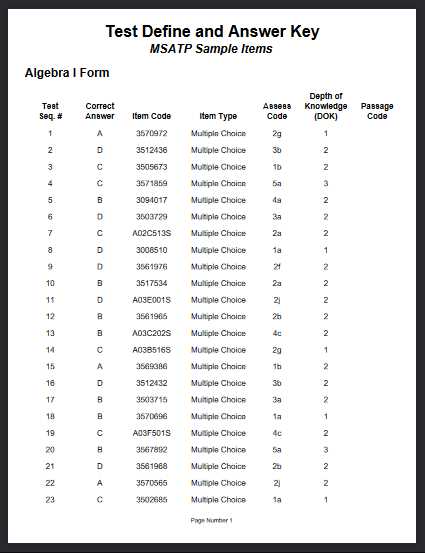
Algebra 1 Final Exam Answer Key Overview

In any major mathematics assessment, it is essential to have access to reliable resources to help you check your work and understand the reasoning behind each solution. This section focuses on providing a comprehensive guide to review materials, ensuring that you are able to accurately verify your solutions and identify areas that need improvement.

Having an organized set of solutions allows for efficient learning and understanding of complex problems. By breaking down the solution steps clearly, you can easily follow through each question and ensure that your approach aligns with the correct methods. With this guide, you will be better equipped to review common types of problems and focus on the most crucial areas for improvement.

How to Use This Guide Effectively

• Start by reviewing each problem step by step to understand the solution process.
• Compare your methods with the provided solutions to identify any discrepancies.
• Focus on the problem areas and revisit similar questions to reinforce understanding.

Common Challenges and Solutions

• Recognizing and correcting miscalculations in complex equations.
• Understanding the proper application of formulas and mathematical rules.
• Improving speed and accuracy when solving word problems and multi-step calculations.

Essential Tips for Algebra 1 Exam Success

Achieving success in any mathematical assessment requires more than just knowledge of formulas and techniques. It is equally important to approach the assessment strategically, ensuring that you are well-prepared and confident in your ability to solve various types of problems. This section provides key recommendations for maximizing your performance and ensuring that you can navigate through complex questions with ease.

Effective preparation involves developing a solid understanding of the fundamental concepts while also practicing regularly to build familiarity with the types of problems you may encounter. Beyond technical skills, good time management, a calm mindset, and a systematic approach can make all the difference.

Key Strategies for Success

Tip	Description
Review Core Concepts	Ensure you have a strong grasp of the fundamental principles that are commonly tested. Regularly revisit key topics such as equations, functions, and graphs.
Practice with Sample Problems	Consistent practice helps reinforce your understanding and makes problem-solving faster. Try solving practice problems under timed conditions to simulate the real experience.
Stay Organized	Keep your notes and materials well-organized. Clear, structured notes make it easier to locate important formulas and solutions during review sessions.
Manage Your Time Wisely	During your preparation, practice solving problems within a set time frame to enhance your efficiency. Allocate specific time slots for each section during the assessment.
Stay Calm and Confident	It’s important to stay calm and focused during the assessment. Take deep breaths and approach each question methodically without rushing.

How to Use the Answer Key Effectively

Accessing reliable solutions after attempting a set of problems can be a great way to enhance your understanding of mathematical concepts. However, simply looking at the results isn’t enough. To gain the most from this resource, it’s important to approach it with a strategy. This section will guide you on how to use the provided solutions in a way that maximizes your learning and helps you improve your problem-solving skills.

Rather than focusing solely on checking if your results are correct, take the time to analyze each step involved in the solution process. By doing so, you can identify where your approach may differ and learn from any mistakes made along the way. This will help you strengthen your overall mathematical abilities and improve your confidence.

Steps to Effectively Use the Solution Guide

• Start by attempting the problem on your own, without looking at the solution.
• Once you’ve completed the problem, compare your solution with the one provided.
• If your solution differs, carefully go through the steps in the provided solution to identify where your approach went wrong.
• Pay close attention to the methods used to simplify or solve equations, as these may introduce new techniques for you to apply in future problems.
• After understanding the correct approach, practice similar problems to reinforce the concepts learned.

Common Mistakes to Avoid When Using the Guide

• Relying too heavily on the solutions without trying the problems yourself.
• Ignoring the process and only checking if the final answer matches.
• Not reviewing the steps carefully enough to understand the logic behind the solution.

Common Mistakes on Algebra 1 Final

Even the most well-prepared students can make mistakes during a math assessment. Recognizing the most common errors can help you avoid them and improve your overall performance. This section highlights frequent pitfalls that learners face when solving complex mathematical problems, allowing you to address these issues proactively.

Whether it’s a simple miscalculation, overlooking an important step, or misunderstanding a concept, being aware of these common mistakes can save you valuable time and prevent unnecessary errors. The key is to approach each problem systematically and double-check your work to ensure you haven’t overlooked any critical details.

Common Errors to Watch Out For

• Misreading the Question: Sometimes students fail to fully understand the problem before attempting a solution. Always take a moment to carefully read each question and ensure you know what is being asked.
• Incorrectly Applying Formulas: Using the wrong formula or applying it incorrectly is a common mistake. Ensure that you are using the appropriate formulas for each type of problem.
• Forgetting to Simplify: Students often forget to simplify their results, leaving answers that are not in their simplest form. Remember to check if the solution can be simplified further.
• Skipping Steps: Rushing through a problem can cause you to skip necessary steps, leading to incorrect answers. Always work through each step in the solution process.
• Sign Errors: Mistaking positive and negative signs, especially when working with equations, is a frequent error. Double-check your signs at every step of the calculation.
• Not Checking Work: Failing to review your solutions is a critical mistake. Set aside time to go over your answers and ensure there are no calculation or logical errors.

Step-by-Step Problem Solving Guide

Solving complex problems requires a structured approach to ensure accuracy and clarity. By breaking down each task into manageable steps, you can systematically tackle even the most challenging questions. This guide will walk you through the essential process for approaching and solving mathematical problems with confidence and efficiency.

The key to successful problem-solving is recognizing the specific method that applies to each type of question. By following a logical sequence and remaining focused, you can eliminate confusion and reduce the risk of errors. This guide will help you understand how to deconstruct problems and apply the right strategies for success.

Steps to Solve Math Problems Effectively

• Read the Problem Carefully: Begin by thoroughly understanding the question. Identify the key information, what is being asked, and the specific approach needed to solve it.
• Organize Your Work: Write down the given data, define variables if necessary, and outline the steps you plan to take. A clear structure will help you stay on track.
• Apply Relevant Formulas: Use appropriate formulas or methods that are relevant to the type of problem you are solving. Double-check that you are applying them correctly.
• Perform Calculations: Carry out the necessary calculations step by step. Keep your work organized and clearly show your steps to avoid errors.
• Simplify the Result: Once you’ve found your answer, ensure it’s in the simplest form, if applicable. This may involve reducing fractions or combining like terms.
• Double-Check Your Work: Review your solution carefully. Recheck calculations and verify that the answer makes sense in the context of the question.

Common Problem-Solving Pitfalls

• Rushing Through Steps: It’s tempting to move quickly through problems, but rushing can lead to mistakes. Take your time and follow each step methodically.
• Neglecting to Simplify: Not simplifying your solution to its most basic form can lead to incomplete answers.
• Overlooking Important Details: Pay attention to all parts of the problem, including units, signs, and additional information, which are essential to finding the correct solution.

Understanding Key Algebraic Concepts

Mastering mathematical principles requires a solid understanding of core concepts that form the foundation of problem-solving. These concepts often appear across various problems and serve as building blocks for more complex ideas. Gaining a clear understanding of these topics will help you navigate through various types of questions with confidence and accuracy.

By focusing on these key concepts, you will develop the ability to recognize patterns, apply appropriate methods, and solve problems efficiently. Whether you’re working with equations, functions, or expressions, understanding the underlying principles is crucial to achieving success.

Essential Concepts to Understand

• Variables and Expressions: Understand how to manipulate variables and simplify expressions. Recognize the role of constants and coefficients within equations and how they relate to each other.
• Equations and Inequalities: Learn how to solve different types of equations and inequalities, including linear and quadratic forms, and understand their graphical representations.
• Functions and Graphs: Be familiar with the concept of a function, how to graph it, and how to analyze the relationships between variables within different function types.
• Factoring: Master the techniques of factoring polynomials, which are essential for solving quadratic equations and simplifying complex expressions.
• Rational Expressions: Understand how to simplify, multiply, and divide rational expressions and how to handle any restrictions in their domains.
• Systems of Equations: Learn how to solve systems of equations using various methods such as substitution, elimination, and graphing.

How These Concepts Relate to One Another

• Understanding the relationship between equations and their solutions helps in solving for unknown variables.
• Graphing functions and interpreting their graphs provides visual insight into the relationships between variables.
• Factoring and simplifying expressions enables you to break down complex problems into more manageable steps.

How to Prepare for the Exam

Proper preparation is the key to success when tackling any mathematical assessment. It’s not just about reviewing formulas or practicing problems, but also about developing a strategy that will help you retain critical information and apply it effectively during the assessment. By focusing on understanding the concepts and organizing your study sessions, you can ensure that you’re ready for the challenge ahead.

To prepare effectively, it’s essential to approach your study plan in a structured way. Identify areas where you need the most practice and allocate more time to these topics. Additionally, practicing problems under timed conditions can simulate the real test environment and improve your time management skills.

Effective Study Strategies

• Create a Study Schedule: Plan your study time in advance, allowing adequate time for each topic. Prioritize areas where you’re struggling or that carry more weight on the test.
• Use Practice Problems: Work through a variety of practice problems to reinforce your understanding. Focus on problems similar to those you expect to encounter.
• Review Mistakes: After solving problems, review any mistakes carefully to understand where you went wrong. This helps prevent the same errors from happening again.
• Group Study Sessions: Studying in groups allows you to discuss difficult topics and explain concepts to others, reinforcing your own understanding.

Additional Tips for Test Day

• Get Enough Rest: Ensure you are well-rested the night before the test. A clear and rested mind will perform better under pressure.
• Stay Calm and Focused: On the day of the test, stay calm and approach each problem with a clear strategy. Don’t panic if you encounter a difficult question.
• Manage Your Time Wisely: Allocate a set amount of time for each section, and if you get stuck on a problem, move on and return to it later.

Strategies for Time Management During Exams

Managing your time effectively during an assessment is crucial to ensuring you complete all sections and perform to the best of your abilities. Time management allows you to approach each problem with a clear mind, avoid rushing, and minimize the likelihood of errors. With the right strategies, you can navigate the test confidently and maximize your performance.

During any timed assessment, the ability to prioritize tasks and allocate sufficient time to each section is essential. Developing a strategy before the test begins will help you stay on track and avoid feeling overwhelmed. It’s also important to remember that pacing yourself is as critical as solving the problems themselves.

Effective Time Management Tips

• Read the Instructions Carefully: Before starting, take a few moments to read through the instructions and understand the structure of the test. This will help you plan how to allocate your time.
• Start with the Easier Questions: Begin by tackling the questions that are easier for you. This will build confidence and ensure you gain easy points early on, leaving more time for the harder questions.
• Estimate Time per Section: Divide the total time by the number of sections or questions and estimate how much time you should spend on each part. Stick to this plan as closely as possible.
• Skip and Return: If you come across a particularly difficult problem, skip it and move on to the next one. Returning to it later with fresh eyes can make it easier to solve.
• Use a Timer: Bring a watch or use a timer to keep track of the time. Set reminders at intervals to ensure you’re staying on track and not spending too much time on one section.

Handling Stress and Staying on Track

• Stay Calm and Breathe: If you start to feel rushed or stressed, pause for a moment to take deep breaths. Staying calm will help you think more clearly and manage your time more effectively.
• Review and Adjust: If you’re running short on time, quickly review how far you’ve gotten and adjust your strategy. Focus on answering as many questions as possible, even if it means skipping some parts.

Importance of Reviewing Practice Problems

Reviewing practice problems is a critical part of preparing for any assessment. It not only helps reinforce the concepts you’ve learned but also highlights areas where you may need additional practice. By thoroughly working through sample problems, you can build a deeper understanding of how to apply different methods and avoid common mistakes.

Through consistent review, you become more familiar with the structure of questions and develop strategies to tackle them efficiently. Additionally, understanding why certain solutions work, and others don’t, can improve your problem-solving skills and boost your confidence during the actual test.

Benefits of Reviewing Practice Problems

• Identify Common Mistakes: By reviewing your solutions, you can spot recurring errors and focus on correcting them before the real test.
• Improve Problem-Solving Skills: Repetition helps reinforce the techniques needed to solve different types of problems, enhancing your speed and accuracy.
• Build Confidence: Consistent practice boosts your self-assurance, making it easier to approach more challenging problems during the test.
• Master Time Management: Reviewing problems under timed conditions helps you practice pacing yourself and managing your time more effectively.

How to Review Practice Problems Effectively

• Work Through Multiple Problems: Don’t just focus on one type of problem; practice a variety of questions to strengthen your overall understanding.
• Understand the Solutions: Simply knowing the correct answer isn’t enough. Take time to understand why a solution works and what strategies were used to arrive at it.
• Revisit Difficult Problems: If you find certain problems challenging, revisit them regularly to reinforce your skills and improve your approach.
• Seek Help When Needed: If you’re struggling with a particular concept, seek clarification from teachers, peers, or additional resources to ensure you understand the material fully.

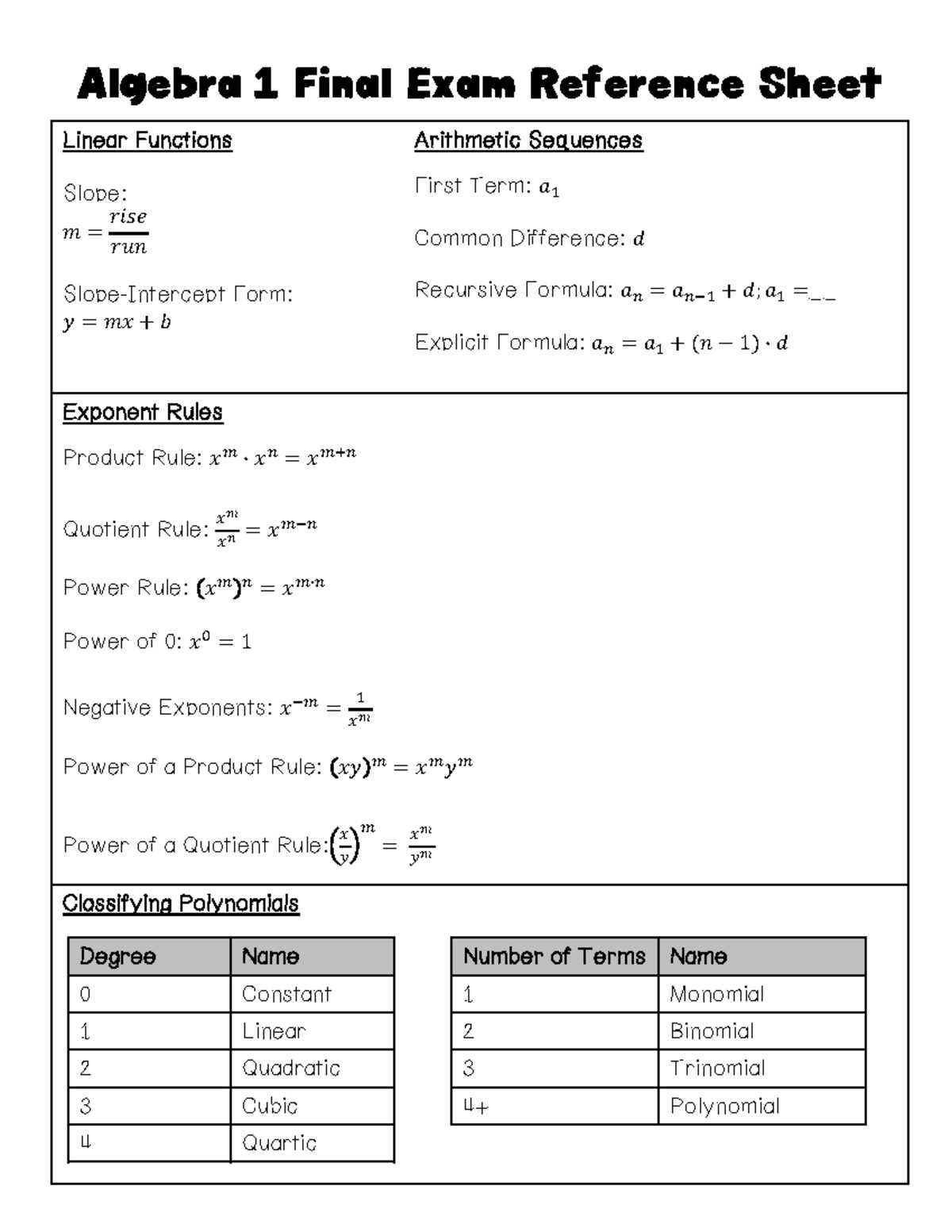
Key Formulas for Algebra 1 Finals

Having a solid grasp of essential formulas is crucial for success in any mathematics assessment. These formulas serve as the foundation for solving various problems efficiently. Knowing when and how to apply them can significantly simplify complex questions and save valuable time during the test. In this section, we’ll highlight some of the most important formulas you’ll need to remember.

From basic operations to more advanced techniques, mastering these formulas ensures that you can approach each problem with confidence. Make sure to understand the logic behind each formula and practice applying them in different contexts to solidify your knowledge.

Here are some key formulas to keep in mind:

• Linear Equation: y = mx + b – Represents the equation of a straight line where m is the slope and b is the y-intercept.
• Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a – Used to find the roots of a quadratic equation ax² + bx + c = 0.
• Point-Slope Form: y – y₁ = m(x – x₁) – A way to express the equation of a line given a point (x₁, y₁) and the slope m.
• Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) – Helps calculate the slope between two points (x₁, y₁) and (x₂, y₂).
• Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²) – Used to find the distance between two points in the coordinate plane.
• Pythagorean Theorem: a² + b² = c² – Relates the sides of a right triangle, where c is the hypotenuse and a and b are the other sides.

By reviewing and understanding these formulas, you will be better equipped to handle a variety of problems. Regular practice with these key concepts can make a significant difference when it comes time to apply them during the assessment.

What to Do After Completing the Exam

Once you have finished your assessment, it’s important to stay calm and approach the next steps thoughtfully. Many students rush to finish, but the time after completing the test can be just as crucial for your overall preparation and peace of mind. Taking a moment to reflect and double-check your work can make a significant difference in ensuring that you did your best.

Instead of immediately worrying about the outcome, take this time to relax and gather your thoughts. Reflect on any difficult questions and assess whether you may have missed something important. It’s also helpful to understand how you can improve for future assessments, regardless of how well you think you did.

Here are some things to consider after finishing the assessment:

• Review Your Responses: If time permits, quickly scan through your answers to check for any mistakes or overlooked questions.
• Stay Calm: Avoid stressing about the results immediately after completing the assessment. Trust in your preparation.
• Ask for Clarification: If you have any lingering doubts or need further explanation, approach the instructor or exam coordinator for feedback.
• Reflect on Your Approach: Think about which parts of the test you felt most confident about and which areas could use more focus for next time.
• Take a Break: After the test, give yourself some time to relax and recharge, especially if you have more assessments ahead.

By following these steps, you not only ensure that you’ve done your best but also set yourself up for improvement in future assessments. Staying composed and thoughtful after completing the test can help reduce any anxiety and contribute to a better overall learning experience.

Tips for Analyzing Incorrect Answers

When reviewing a completed assessment, it’s essential to carefully analyze any mistakes. Understanding why a particular solution was incorrect not only helps identify gaps in knowledge but also allows you to refine your problem-solving strategies for future challenges. By dissecting each error, you can better recognize patterns and avoid repeating the same mistakes.

Rather than viewing incorrect responses as failures, approach them as opportunities for improvement. Take the time to break down your thought process and pinpoint where things went wrong. This critical reflection will not only boost your understanding but also build confidence in tackling similar problems in the future.

Here are some helpful tips for analyzing your incorrect responses:

• Revisit the Question: Ensure you understood what was being asked before jumping into solving the problem. Misinterpreting the question can lead to errors.
• Identify Calculation Mistakes: Double-check your mathematical steps. A small mistake in arithmetic or simplifying can lead to incorrect results.
• Check Your Work Method: Review the method or approach you used. Sometimes, a wrong formula or incorrect strategy might have been applied.
• Understand the Concept: Analyze whether the mistake was due to a lack of understanding of the underlying concept. If so, take the time to review the theory behind it.
• Look for Patterns: If you made similar mistakes on multiple questions, try to spot a pattern. It could indicate an area that needs more attention or practice.
• Seek Feedback: If possible, ask for feedback from a teacher or peer. Discussing the mistake with someone else can offer a new perspective and clarify any confusion.

By carefully analyzing incorrect responses, you not only improve your skills but also gain a deeper understanding of the material. Taking a reflective approach will ensure that you are better prepared for future challenges and able to overcome any hurdles more effectively.

Breaking Down Difficult Problems

When faced with challenging questions, it’s important to approach them systematically to make them more manageable. Complex problems can often seem overwhelming at first glance, but breaking them down into smaller, more digestible steps can help clarify the process. This method not only reduces anxiety but also enhances your ability to solve similar problems in the future.

By carefully analyzing each part of a difficult problem, you can uncover the underlying concepts that are being tested and determine the best approach to solve it. The key is to remain patient and persistent, as complex problems are often built on simpler principles that, once understood, can be tackled more effectively.

Step-by-Step Approach

Here is a methodical way to break down difficult problems:

• Read the Problem Thoroughly: Start by reading the entire problem carefully to ensure you understand what is being asked. Pay attention to key terms and conditions.
• Identify Known and Unknown Values: Write down the information given in the problem, and highlight the unknowns. This will help you visualize the problem.
• Choose the Right Method: Determine which method or formula is best suited for solving the problem. This may involve selecting the appropriate equation or strategy.
• Solve in Steps: Break the solution into smaller parts. Solve one step at a time, ensuring that each intermediate result makes sense before moving to the next.
• Check Your Work: Once you arrive at a solution, go back through your steps to verify that your calculations and logic were correct.

Strategies for Overcoming Obstacles

If you get stuck, here are a few strategies to keep in mind:

• Look for Patterns: Sometimes, recognizing patterns in the problem can lead to shortcuts or reveal a more straightforward solution.
• Take Breaks: If frustration sets in, step away for a few minutes. Coming back with a fresh mind can help you see the problem from a new perspective.
• Ask for Help: Don’t hesitate to seek clarification from a teacher, tutor, or peer if you’re having trouble understanding a concept or step in the process.

By breaking down difficult problems into smaller steps and using the right strategies, you’ll be able to solve even the most challenging questions with greater confidence and accuracy.

​

Using Online Resources for Help

The internet offers a vast array of tools and platforms that can assist you in mastering mathematical concepts. With the right resources, you can find step-by-step explanations, interactive problem solvers, and video tutorials to guide you through challenging problems. Online platforms allow you to learn at your own pace, providing flexibility to address areas where you need more practice or clarification.

These resources can be particularly helpful when you’re struggling with specific types of problems or concepts. Rather than simply reviewing static textbooks, you can explore dynamic platforms that offer a variety of methods and strategies for solving problems. Whether you prefer watching videos, reading articles, or using interactive tools, the internet provides numerous options to reinforce your understanding.

Top Online Platforms for Assistance

Platform	Description
Khan Academy	Free online lessons with video tutorials and practice exercises on a wide range of mathematical topics.
Wolfram Alpha	Powerful computational engine that can solve problems step-by-step and provide detailed explanations.
Mathway	Interactive tool for solving problems and getting instant solutions with explanations.
PatrickJMT	Video tutorials focused on helping students understand complex mathematical concepts.

How to Use These Resources Effectively

• Start with the Basics: Before jumping into complex problems, use these resources to reinforce foundational knowledge.
• Watch Video Tutorials: Visual learners can benefit from seeing problem-solving strategies demonstrated step-by-step.
• Practice Regularly: Consistent practice is key to mastering any concept. Use interactive platforms to work through problems and check your solutions.
• Seek Help When Needed: If you’re struggling, don’t hesitate to ask questions on forums or reach out to online tutors for personalized help.

By utilizing these online resources, you can enhance your understanding and improve your problem-solving skills. The ability to learn and practice at your own pace will give you the confidence to tackle even the most challenging problems.

Best Study Materials for Mastering Math Concepts

To excel in mathematics, having access to the right resources can make all the difference. Quality study materials provide clear explanations, varied practice exercises, and helpful examples that enhance understanding and retention. Whether you’re reviewing for an important assessment or simply looking to strengthen your skills, the following materials offer valuable support for anyone working through mathematical concepts.

Choosing the right materials can help you build a solid foundation, improve problem-solving strategies, and boost your confidence. The best resources cater to different learning styles, whether you prefer reading detailed explanations, watching instructional videos, or solving interactive problems. Using a variety of materials will ensure that you gain a well-rounded understanding of key topics.

Top Recommended Resources

• Textbooks: Comprehensive textbooks provide in-depth coverage of key topics, step-by-step problem solutions, and practice questions.
• Online Courses: Websites like Coursera, Udemy, and edX offer structured online courses with video lectures, quizzes, and assignments to help reinforce learning.
• Interactive Tools: Websites such as Desmos and GeoGebra allow you to visualize mathematical concepts and interact with graphs and equations.
• Workbooks: Practice workbooks filled with exercises offer an opportunity for hands-on learning, helping to reinforce what you’ve studied.
• Video Tutorials: YouTube channels like PatrickJMT and Khan Academy feature clear and concise video lessons that explain complex concepts in a simple way.

By using a mix of textbooks, online resources, and practice tools, you can ensure that you’re well-prepared to tackle any challenge. Remember, consistency is key when studying, and these materials will give you the variety and depth needed to improve your skills and succeed.

How to Improve Math Skills for Future Assessments

Improving mathematical skills requires consistent practice, understanding fundamental concepts, and learning how to approach problems efficiently. Whether you’re preparing for an upcoming assessment or aiming to strengthen your overall proficiency, adopting effective strategies is crucial for long-term success. By breaking down complex topics into manageable parts and continuously applying what you’ve learned, you can build a strong foundation for future challenges.

The key to mastering math lies in developing a clear approach to problem-solving, recognizing patterns, and refining techniques over time. Below are some essential strategies that can help you sharpen your skills and boost your performance on future tests.

Essential Strategies for Improvement

• Regular Practice: The more you practice, the more familiar you become with the types of problems you will encounter. Consistent daily exercises reinforce learning and help retain concepts.
• Focus on Weak Areas: Identify the topics where you struggle the most and dedicate extra time to understanding these concepts. Breaking them down into smaller steps can make complex topics more manageable.
• Study with a Group: Discussing problems and solutions with peers can help clarify misunderstandings and expose you to different problem-solving methods.
• Use Visual Aids: Graphs, charts, and diagrams can often make abstract concepts more tangible. Tools like graphing calculators or apps can provide visual support and assist in understanding difficult problems.

Helpful Resources

Using the right tools can accelerate your learning process. Below is a list of resources that can enhance your study routine:

Resource Type	Purpose
Textbooks	Provide detailed explanations and practice problems to reinforce key concepts.
Online Practice Tools	Interactive platforms like Khan Academy and Desmos help visualize problems and offer step-by-step guidance.
Workbooks	Provide additional exercises that can help you master different types of questions and practice problem-solving.
Video Tutorials	Allow you to learn at your own pace, with instructors breaking down complex concepts into easy-to-understand steps.

By incorporating these strategies and utilizing these resources, you will be better equipped to tackle any mathematical challenge and improve your performance in future assessments.