In this section, we will explore a range of essential concepts that build a strong foundation for students tackling advanced mathematical challenges. The focus is on developing a deeper comprehension of fundamental principles, which are key to mastering complex topics. Students will gain insight into how to approach different types of problems and enhance their problem-solving abilities.
By carefully analyzing various exercises and learning techniques, learners can improve their skills and confidence in handling different types of calculations. With the proper approach, difficult concepts become more accessible, allowing for more effective study habits. This section serves as a practical guide to achieving success and mastering the material, ensuring students are well-prepared for upcoming challenges.
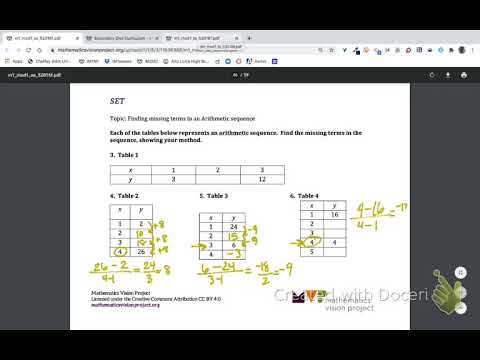
Mathematics Vision Project Module 8 Answer Key
This section provides a comprehensive guide for students working through a challenging set of exercises. It highlights strategies for solving various types of problems and offers insights into common pitfalls and how to avoid them. Understanding these solutions enhances problem-solving skills and ensures a better grasp of complex concepts.
By reviewing detailed solutions, learners can gain clarity on their approach and deepen their understanding of the material. The guide is designed to complement the learning process, reinforcing key principles while helping to overcome any obstacles students may encounter. This approach encourages independent thinking and improves overall performance in tackling related problems.
Understanding Module 8 in Detail
This section focuses on a deep exploration of the content designed to strengthen key skills and broaden understanding in complex areas. The primary goal is to ensure that learners can confidently apply concepts to various scenarios, whether theoretical or practical. The structured approach to these topics builds a strong foundation for more advanced studies.
Within this section, students are introduced to essential techniques, guided through exercises, and offered detailed insights into their applications. These methods help clarify difficult concepts and offer practical strategies for mastering challenges that may initially seem overwhelming.
| Topic | Focus Area | Key Skill Developed |
|---|---|---|
| Algebraic Expressions | Understanding variable manipulation | Solving equations |
| Geometric Principles | Shapes, angles, and their properties | Critical thinking and spatial reasoning |
| Data Interpretation | Analyzing and interpreting graphs | Data-driven decision-making |
Key Concepts in Mathematics Vision Project
In this section, we focus on the fundamental principles that serve as the backbone of the curriculum. These concepts are designed to deepen understanding and provide a solid framework for tackling more advanced topics. Grasping these core ideas is essential for building proficiency in problem-solving and critical thinking.
Algebraic manipulation and geometric reasoning are central to the approach, emphasizing not only the ability to solve equations but also to comprehend the underlying relationships between numbers and shapes. A strong grasp of these areas is crucial for understanding more complex ideas and applying them in diverse contexts.
Another important area is data analysis, which involves interpreting and making decisions based on numerical information. Students are encouraged to explore different methods for organizing and analyzing data, helping them develop a more analytical mindset that can be applied across various disciplines.
How to Use the Answer Key Effectively
Using the provided solutions is an essential part of the learning process, but it’s important to approach them with the right mindset. Instead of simply copying the results, students should focus on understanding the reasoning behind each step. This will not only help reinforce the material but also develop problem-solving skills that can be applied to future challenges.
Step-by-Step Review
Carefully examine each solution and work through the steps on your own before checking the provided answers. This will help you identify any areas where you might have gone wrong and improve your understanding of the method used. Reflecting on the process will strengthen your comprehension and ability to apply the technique independently in different situations.
Utilize as a Learning Tool
Rather than viewing the solutions as a shortcut, use them as a guide to refine your approach. Highlight key concepts and methods that you find challenging, and try to apply them to similar problems. This active engagement with the material ensures that you are truly absorbing the information rather than just memorizing answers.
Important Topics Covered in Module 8
This section delves into a variety of fundamental topics that play a crucial role in building a deeper understanding of key concepts. These subjects not only reinforce essential techniques but also pave the way for more complex applications in future studies. By mastering these areas, learners can improve their problem-solving abilities and approach challenges with confidence.
Algebraic Techniques and Strategies
A significant focus is placed on refining algebraic skills, such as simplifying expressions and solving equations. Students will gain a thorough understanding of how to manipulate variables and apply these methods to real-world scenarios, strengthening their overall mathematical fluency.
Geometric Relationships and Applications
The study of geometric properties, including shapes, angles, and their interrelationships, is another key area. By learning how to visualize and calculate geometric figures, students enhance their spatial reasoning and analytical abilities, which are vital in both academic and practical contexts.
Step-by-Step Approach to Solving Problems
To effectively tackle challenging tasks, it’s essential to follow a structured process that breaks down complex problems into manageable steps. By approaching each problem systematically, learners can avoid feeling overwhelmed and ensure that they are focusing on the right aspects at each stage. This method not only improves accuracy but also enhances critical thinking skills.
The following steps outline a recommended approach for solving problems efficiently:
- Read the Problem Carefully: Understand the given information and identify what is being asked.
- Organize the Information: Write down known values, variables, and relationships to clarify the problem.
- Choose a Strategy: Decide on the most appropriate method or formula to use based on the problem’s type.
- Work Through the Steps: Apply the chosen strategy step-by-step, showing all calculations or reasoning.
- Check Your Work: Review the solution to ensure there are no errors or miscalculations.
By consistently following these steps, students will develop a more methodical approach to problem-solving, which can be applied across various topics and challenges.
Common Mistakes to Avoid in Module 8
As with any complex topic, there are certain pitfalls that learners often encounter when working through exercises. Recognizing these common mistakes early on can help prevent frustration and ensure a more effective learning process. By being aware of these errors, students can improve their problem-solving strategies and avoid repeating the same missteps.
Misinterpreting the Problem Statement
One of the most frequent mistakes is misunderstanding the question or misinterpreting the information provided. It’s essential to read the problem carefully and identify exactly what is being asked. Taking the time to break down the question into smaller, manageable parts can prevent confusion and lead to more accurate solutions.
Skipping Steps or Rushing Through Calculations
Another common error is attempting to skip steps or rush through the process, especially when working with more complex problems. It’s important to show each step clearly and double-check calculations to avoid simple arithmetic mistakes. Taking a methodical approach ensures that no critical details are overlooked and helps prevent errors in the final solution.
How Module 8 Enhances Problem Solving Skills
This section focuses on how the material helps students develop essential problem-solving abilities. By working through various challenges, learners are exposed to different strategies and techniques that not only improve their analytical thinking but also enhance their ability to approach unfamiliar problems with confidence. The goal is to build a strong foundation for solving increasingly complex tasks with greater efficiency and precision.
The exercises provided throughout this section are designed to sharpen reasoning skills and encourage students to think critically. These tasks allow learners to practice applying different methods and techniques, ultimately reinforcing their ability to identify the best approach for each situation.
| Problem Type | Skills Developed | Approach Used |
|---|---|---|
| Algebraic Equations | Logical reasoning, pattern recognition | Step-by-step solving, simplification |
| Geometric Problems | Spatial reasoning, visualization | Drawing diagrams, applying properties |
| Data Analysis | Interpretation, decision-making | Organizing data, drawing conclusions |
Practical Applications of Mathematical Principles
Understanding the core concepts behind number manipulation, patterns, and logical reasoning extends far beyond the classroom. These principles play a vital role in various real-world applications, from engineering to economics. By mastering these skills, individuals are better equipped to tackle problems in everyday situations, whether in their careers or personal lives.
In this section, we explore how mathematical concepts are applied in different fields, demonstrating their relevance and importance in shaping solutions to practical challenges.
Engineering and Design
Mathematical principles are crucial in designing structures, optimizing systems, and ensuring safety in engineering projects. Concepts such as geometry, algebra, and trigonometry are used to calculate measurements, analyze forces, and solve for unknowns in blueprints and models.
Finance and Economics
From budgeting to investment analysis, mathematical principles form the foundation of financial decision-making. Techniques such as statistical analysis, probability, and compound interest calculations help individuals and businesses make informed choices and predict trends.
| Application Area | Mathematical Concepts Used | Impact |
|---|---|---|
| Engineering | Geometry, Algebra, Trigonometry | Improves accuracy and efficiency in design |
| Finance | Statistics, Probability, Compound Interest | Helps in making better investment decisions |
| Computer Science | Algorithms, Cryptography, Logic | Enhances security and system optimization |
How to Approach Word Problems in Module 8
Word problems often pose a unique challenge because they require more than just applying formulas or performing calculations–they demand the ability to interpret a narrative and extract relevant information. The key to solving these types of problems is to break them down into manageable parts and systematically address each one. By following a clear approach, students can improve their understanding and gain confidence in tackling even the most complex word-based challenges.
Here’s a structured approach to help you effectively solve word problems:
- Read the Problem Carefully: Before jumping into calculations, take the time to understand what the problem is asking. Identify the key information and note any numbers or variables provided.
- Visualize the Problem: Drawing a diagram or creating a chart can make abstract information more concrete, especially for problems involving geometry or data.
- Translate Words into Mathematical Terms: Convert the phrases or descriptions into equations or expressions that can be solved.
- Choose the Appropriate Strategy: Determine the best approach based on the problem’s type–whether it’s algebraic, geometric, or involves data analysis.
- Check Your Solution: After solving, review your work to ensure that the solution makes sense in the context of the problem.
By following these steps, learners will develop a logical and methodical approach to solving word problems, making the process less daunting and more approachable.
Mastering Algebra and Geometry in Module 8
To excel in solving mathematical problems, it’s essential to have a firm grasp of both algebraic and geometric principles. These areas are foundational in understanding a wide range of problems and can often be interwoven to provide more efficient solutions. Mastering the techniques within these fields equips learners with the tools needed to approach challenges with confidence and clarity.
In this section, we focus on key strategies for mastering algebra and geometry, breaking them down into digestible steps and offering practical tips for applying them in different contexts.
Mastering Algebraic Techniques
Algebra is a critical tool for solving equations and understanding relationships between variables. By applying algebraic rules and methods, students can manipulate equations to isolate unknowns and solve for specific values.
- Solving Equations: Use inverse operations to isolate the variable on one side of the equation.
- Working with Expressions: Simplify expressions by combining like terms and applying distributive properties.
- Factoring: Recognize patterns and factor quadratic expressions to simplify complex problems.
Enhancing Geometry Skills
Geometry involves the study of shapes, sizes, and the properties of space. Mastering geometric principles helps students visualize problems and apply appropriate formulas to find unknown dimensions or areas.
- Understanding Shapes: Study the properties of different geometric figures like triangles, circles, and quadrilaterals.
- Using Formulas: Apply area, perimeter, and volume formulas to solve for dimensions in geometric problems.
- Visualizing Problems: Draw diagrams to scale to better understand relationships between different parts of a figure.
By refining skills in both algebra and geometry, learners gain a deeper understanding of how these concepts work together, helping them approach problems from a more holistic perspective.
Review of Key Exercises in Module 8
Reviewing key exercises helps consolidate knowledge and provides an opportunity to reinforce important concepts. In this section, we’ll go over some of the most essential exercises that challenge learners to apply what they’ve learned and test their understanding. These exercises offer practical ways to engage with the material and improve problem-solving abilities.
Here are some of the critical exercises to focus on:
Essential Algebraic Problems
Algebraic exercises test the ability to manipulate variables, solve for unknowns, and simplify expressions. These exercises are fundamental to mastering equations and developing logical thinking.
- Solving Linear Equations: Practice solving equations with one variable, ensuring a solid understanding of inverse operations and balancing both sides.
- Factoring Quadratic Expressions: Work on factoring expressions into binomial products to solve quadratic equations more easily.
- Systems of Equations: Solve problems that involve two or more equations, requiring substitution or elimination methods.
Geometry Practice Problems
Geometry exercises focus on shapes, their properties, and spatial relationships. These problems encourage visualization and application of formulas for measurement.
- Area and Perimeter: Solve for the area and perimeter of various shapes, including triangles, rectangles, and circles.
- Volume of Solids: Work on problems that involve calculating the volume of three-dimensional figures such as spheres, cylinders, and prisms.
- Angle Relationships: Use properties of angles, such as complementary and supplementary angles, to solve problems involving intersecting lines and polygons.
By revisiting and solving these key exercises, learners can deepen their comprehension and enhance their ability to approach future problems with confidence and precision.
Tips for Efficient Study of Module 8
Efficient studying is crucial for mastering complex topics and achieving success. By using the right strategies, learners can optimize their study time, enhance retention, and strengthen their understanding of the concepts. This section provides practical tips to help streamline your approach and ensure that you’re getting the most out of your study sessions.
1. Break Down the Material into Manageable Segments
Rather than trying to absorb everything at once, divide the content into smaller, more manageable sections. Focus on mastering one concept at a time, allowing yourself to fully grasp each idea before moving on to the next.
- Identify Key Concepts: Prioritize the most important topics, such as algebraic manipulation and geometric formulas, and tackle them first.
- Review Step by Step: After understanding a concept, practice applying it through examples before moving to the next topic.
2. Practice Consistently with Problems and Exercises
Consistency is key when it comes to strengthening problem-solving skills. Regularly practicing problems allows you to apply theoretical knowledge and identify areas where you need further improvement.
- Work on Practice Sets: Solve various problems related to the topics covered, using exercises from the textbook or additional resources.
- Simulate Real Test Conditions: Time yourself while solving exercises to improve speed and efficiency under pressure.
3. Use Visual Aids for Better Understanding
Sometimes, seeing the information visually can make complex concepts much easier to understand. Diagrams, charts, and other visual tools help reinforce key ideas and can improve your spatial reasoning.
- Draw Diagrams: For geometry problems, always sketch the figures to better understand the relationships between shapes and angles.
- Use Graphs: When working with algebraic expressions, plotting graphs can provide insight into the behavior of functions and equations.
4. Review Mistakes and Learn from Them
Don’t overlook errors you make while practicing problems. Each mistake is an opportunity to learn and improve your approach. Reviewing mistakes helps identify weaknesses and ensures that similar errors don’t occur in the future.
- Analyze Errors: When you make a mistake, take the time to review the correct solution and understand where you went wrong.
- Seek Clarification: If a concept still seems unclear after practicing, don’t hesitate to ask for help or consult additional resources.
By implementing these strategies, you can study more effectively, retain more information, and build a solid foundation for solving problems efficiently.
How Module 8 Prepares Students for Advanced Topics
Understanding foundational principles is key to excelling in more complex areas. The approach taken in this section helps students build the necessary skills and knowledge to succeed as they progress to more advanced subjects. By mastering core concepts and engaging in hands-on problem solving, students are better equipped to tackle challenging material in the future.
1. Strengthening Fundamental Concepts
The first step in preparing for advanced topics is ensuring that the basics are thoroughly understood. By focusing on essential concepts such as algebra, geometry, and logic, students develop a strong foundation that will support their learning in more specialized fields.
- Mastering Key Techniques: Learning how to manipulate expressions, solve equations, and apply geometric formulas is essential for tackling more advanced math topics.
- Building Problem-Solving Skills: Exposure to various problem types helps students approach complex challenges with confidence and clarity.
2. Developing Critical Thinking and Analytical Skills
Advanced topics often require students to think critically and analyze complex scenarios. This section encourages students to approach problems methodically, breaking them down into manageable parts, which is a valuable skill in advanced studies.
- Logical Reasoning: Students learn how to apply logical steps to solve problems, preparing them for higher-level courses that demand more abstract thinking.
- Critical Analysis: By regularly practicing problem-solving, students improve their ability to analyze different methods and solutions, a key skill for tackling advanced topics.
3. Preparing for Higher-Level Applications
As students advance, they will encounter real-world applications that require a deep understanding of theoretical principles. This section ensures that learners are exposed to practical problem-solving techniques that will serve them in more advanced studies.
- Real-World Connections: Applying abstract concepts to real-world problems makes it easier for students to relate to more advanced material in fields like calculus or engineering.
- Building Versatile Skills: The skills developed in this section are not only applicable to mathematical problems but also to other disciplines, preparing students for interdisciplinary challenges.
4. Confidence for Future Challenges
By reinforcing essential skills and providing opportunities to practice complex problems, students build the confidence needed to take on more difficult content in the future. This confidence is crucial as they encounter increasingly challenging topics and expand their knowledge base.
- Encouraging Perseverance: Students learn to approach challenges with patience and persistence, essential qualities for tackling advanced coursework.
- Boosting Confidence: As students solve increasingly difficult problems, they gain the assurance needed to excel in future academic pursuits.
By focusing on core principles, developing critical thinking, and ensuring practical application, this section effectively prepares students for success in higher-level subjects, fostering the skills and mindset needed for tackling future challenges with confidence.
Improving Understanding Through Practice
Reinforcing concepts through repeated practice is a proven method to solidify knowledge and improve overall comprehension. By actively engaging with the material, students can deepen their understanding and become more proficient in solving problems. The more students practice, the more confident they become in applying learned techniques to various challenges.
1. The Power of Repetition
Repetition helps internalize key concepts, allowing students to recognize patterns and apply solutions more efficiently. The more students encounter different problem types, the quicker they can identify the most effective approach to solving them.
- Faster Recall: Repeated practice helps students recall information faster, which is crucial for problem-solving in time-sensitive scenarios.
- Strengthening Neural Connections: Consistent practice reinforces neural pathways, making it easier for students to apply learned strategies when faced with new challenges.
2. Gaining Confidence Through Practice
As students work through various exercises, they build confidence in their abilities. Mastery of key concepts comes not just from understanding them but from successfully applying them in different contexts.
- Boosting Self-Assurance: Successfully solving problems over time leads to increased self-assurance, making students more willing to take on difficult material.
- Learning from Mistakes: Practice allows students to make mistakes in a low-pressure environment, where they can learn from them and adjust their approach accordingly.
3. Developing Problem-Solving Strategies
Repeated practice helps students refine their approach to solving problems. With each new exercise, students develop better strategies and learn how to adapt their methods depending on the problem at hand.
- Identifying Patterns: Through practice, students begin to see recurring structures in problems, enabling them to apply the most effective techniques.
- Adapting Approaches: Regular exposure to diverse problems allows students to adjust their problem-solving strategies, improving flexibility in their thinking.
4. Building Long-Term Retention
Practice not only improves immediate problem-solving abilities but also enhances long-term retention of the material. By continuously revisiting concepts, students strengthen their understanding, making it easier to recall knowledge in the future.
- Reinforcing Memory: Repetition strengthens memory, ensuring that concepts stay fresh over time and are easier to recall when needed.
- Long-Term Mastery: Consistent engagement with the material results in deeper mastery, which is essential for more advanced coursework and real-world applications.
In summary, the more students practice, the more their understanding solidifies. By repeatedly engaging with the material, they build both confidence and proficiency, ultimately mastering the skills necessary for tackling more complex tasks with ease.
Assessing Your Progress in Module 8
Regular self-assessment is essential for tracking your understanding and growth. By evaluating how well you are grasping the concepts, you can identify areas that need improvement and refine your skills. This process not only helps measure your current level of knowledge but also guides your future learning efforts.
1. Reflecting on Completed Exercises
One of the best ways to gauge your progress is by reviewing the exercises you’ve completed. Reflecting on the solutions and the methods used to solve problems provides valuable insights into your comprehension and areas that might need more focus.
- Check for Consistency: Are you consistently applying the correct techniques to solve problems, or do you find yourself making frequent errors?
- Identify Patterns: Can you identify any common strategies or patterns that have worked well, or do you still struggle with certain types of problems?
2. Using Practice Tests to Track Progress
Taking regular practice tests is an effective way to measure how much you’ve retained and where you stand in terms of readiness. These tests allow you to apply your knowledge under timed conditions, mimicking real-life scenarios.
- Compare Results: Look at how your scores have improved over time. This can indicate whether your understanding is deepening or if there are areas still needing attention.
- Analyze Mistakes: When you make errors, take the time to understand why. This helps prevent similar mistakes in the future and solidifies your grasp of the material.
3. Seeking Feedback from Others
Another valuable method of assessing progress is through feedback from peers, mentors, or instructors. They can offer an external perspective on your strengths and areas for improvement, helping you identify blind spots that you might miss on your own.
- Peer Review: Sharing your solutions with others allows for collaborative learning and can help you refine your approach.
- Mentor Guidance: An experienced mentor can offer tailored advice and point out specific areas where you can improve.
4. Setting Personal Goals
Setting clear, measurable goals helps you stay motivated and focused. By regularly reassessing these goals and adjusting them based on your progress, you can continue to challenge yourself and push forward in your learning journey.
- Short-Term Goals: Set smaller, achievable objectives that allow you to track incremental progress and stay encouraged.
- Long-Term Goals: Establish broader goals to work toward, ensuring that your learning efforts align with your overall academic or personal aspirations.
By incorporating these strategies, you can effectively assess your progress and make informed decisions about where to focus your efforts next. Regular reflection, feedback, and goal-setting ensure continued growth and improvement in your skills.