
Preparing for a standardized test in mathematics can be a daunting task, but with the right approach, it becomes a manageable and rewarding experience. Understanding the structure and content of the test is crucial for maximizing your potential. Success lies in a solid grasp of fundamental principles, effective problem-solving strategies, and time management during the test itself.
Strategic preparation involves reviewing essential concepts, practicing sample questions, and familiarizing yourself with the types of problems you are likely to encounter. Analyzing patterns in the types of questions asked allows you to focus your efforts on the areas that matter most. By refining your approach to complex calculations and logical reasoning, you can build confidence and improve your performance.
In this section, we will guide you through essential techniques and tips that will not only prepare you for success but also help you navigate challenges with greater ease. Whether you’re tackling equations, graphs, or problem-solving scenarios, mastering these skills will make a significant difference in your results.
Algebra 1 Regents Exam Answers
In any mathematics assessment, solving the right problems accurately is key to achieving high marks. The evaluation focuses on testing a student’s understanding of core principles and their ability to apply them under timed conditions. Here, we will explore how to approach and understand typical questions that appear in these types of assessments, as well as how to check your solutions effectively.
To achieve success, it’s important to break down the question types, identify which methods to apply, and understand the common steps involved in solving them. Below is a table that outlines common problem types and the typical solutions associated with each:
| Problem Type | Solution Method |
|---|---|
| Linear Equations | Solve using substitution or elimination methods |
| Quadratic Equations | Factor, complete the square, or use the quadratic formula |
| Graphing Functions | Plot key points and analyze slope and intercepts |
| Word Problems | Translate the situation into an equation and solve |
| Inequalities | Graph and interpret solution sets |
Mastering these strategies can provide clarity when solving problems and ensure that your approach is structured and efficient. While specific methods may vary, the key is to stay consistent with your process and double-check your work for accuracy.
Overview of the Algebra 1 Exam
The assessment is designed to evaluate students’ understanding of fundamental mathematical concepts and their ability to apply these principles to solve problems. It tests a variety of topics, ensuring that students can not only recall facts but also demonstrate problem-solving skills in different contexts. This section provides an overview of the key components and structure of the assessment.
The test is typically divided into several sections, each focusing on a specific aspect of mathematics. Here are the main topics you will encounter:
- Linear equations and inequalities
- Graphing functions and interpreting data
- Quadratic equations and polynomial expressions
- Word problems and real-life applications
- Systems of equations and solutions
The format usually includes multiple-choice questions, short answer problems, and extended response tasks. Each section is crafted to test different levels of understanding, from basic computation to more complex analysis. The test is structured to assess both theoretical knowledge and practical application of various mathematical tools.
By understanding the breakdown of the test and reviewing each topic thoroughly, students can increase their chances of performing well. It’s important to familiarize yourself with the problem types and practice solving them efficiently to manage time effectively during the test.
Common Topics on the Exam
The assessment covers a wide range of topics, focusing on essential mathematical concepts that students are expected to master. These topics require not only an understanding of theoretical principles but also the ability to apply them to solve practical problems. Below are the most commonly tested subjects that you should focus on during your preparation.
Linear Relationships and Equations
One of the fundamental areas of focus is linear relationships. This includes solving equations, graphing lines, and interpreting slope and intercepts. Understanding how to manipulate these equations and represent them visually is critical to performing well in this section.
Quadratic Functions and Expressions
Another key area of the test involves quadratic equations and functions. This section tests your ability to factor expressions, solve for roots, and understand the properties of parabolas. Mastery of these concepts is essential for success in many of the assessment’s questions.
| Topic | Key Concepts |
|---|---|
| Linear Equations | Slope, y-intercept, graphing, solving |
| Systems of Equations | Substitution, elimination, graphing solutions |
| Quadratic Functions | Factoring, solving for roots, vertex form |
| Inequalities | Solving, graphing, interpreting solution sets |
| Word Problems | Translating real-world situations into equations |
Familiarizing yourself with these common topics and practicing related problems will help you feel confident and prepared when you encounter similar questions during the assessment. The more you practice, the more you will develop the ability to recognize patterns and solve problems efficiently.
How to Approach Algebra 1 Problems
Successfully solving mathematical problems requires a clear, structured approach. Understanding the problem, identifying the necessary steps, and applying the right techniques are essential for achieving the correct solutions. This section will guide you through an effective method for tackling problems with confidence and accuracy.
Start by carefully reading each question to ensure you understand what is being asked. Break the problem into manageable parts, and look for clues that indicate which mathematical tools are required. Whether you are solving equations, graphing, or working with word problems, taking the time to organize your thoughts will help you stay focused and on track.
Next, choose an appropriate strategy based on the type of problem. For equations, decide whether to use substitution, elimination, or graphing. For quadratic expressions, consider factoring, completing the square, or applying the quadratic formula. Each problem type has specific approaches that work best, and practicing these methods will make solving problems more efficient.
Lastly, after solving the problem, always review your solution. Double-check your calculations and ensure that your answer makes sense in the context of the question. This final step can help catch simple mistakes and ensure accuracy in your results.
Time Management Strategies for Test Day
Effective time management is crucial for performing well on any assessment. With limited time to complete a variety of questions, it’s essential to stay organized, stay calm, and allocate your time wisely. Proper planning can help ensure that you have enough time to tackle all sections and review your work thoroughly.
Start by familiarizing yourself with the structure of the test. Knowing how much time you have for each section will allow you to pace yourself accordingly. Aim to spend more time on the questions that carry more weight or require more complex reasoning, while ensuring you don’t spend too much time on any single problem.
A good strategy is to begin with the questions that you find easiest. This boosts confidence and ensures that you secure quick points early in the test. Afterward, move on to the more challenging problems, and leave any especially tough ones for the end. This approach helps you manage time efficiently and prevents you from getting stuck on difficult questions.
Additionally, keep track of the time during the test. Set checkpoints for yourself to monitor your progress. For example, you can aim to finish a section within a set amount of time, and if you fall behind, adjust your pace accordingly. This will help you stay on track and avoid rushing at the end.
Finally, leave a few minutes at the end of the test to review your answers. This final check can help catch any mistakes and improve the accuracy of your solutions.
Understanding Key Algebraic Concepts
To perform well in any mathematical assessment, it’s essential to have a strong understanding of core mathematical ideas. Mastering these concepts will allow you to solve problems more effectively and build a solid foundation for more complex topics. This section focuses on the fundamental principles you should grasp before tackling more advanced questions.
Core Principles to Focus On
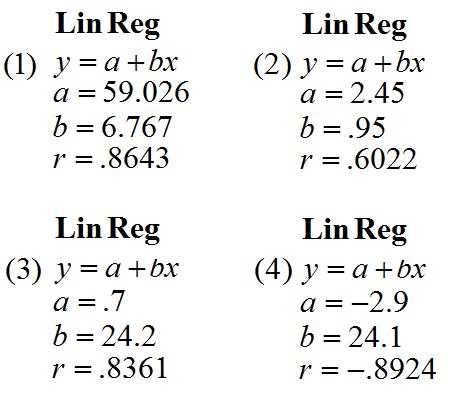
There are several key concepts that form the foundation of problem-solving in mathematics. Understanding these principles will help you approach different types of questions with greater confidence:
- Linear relationships and their properties
- Manipulating equations and inequalities
- Functions and their graphical representations
- Working with exponents and polynomials
- Factoring expressions and solving quadratic equations
Applying These Concepts
Once you understand these fundamental ideas, the next step is learning how to apply them effectively. For example, solving linear equations involves finding unknown values by isolating variables. Similarly, solving quadratic equations requires factoring, completing the square, or using the quadratic formula. By practicing these methods and understanding when to use each technique, you’ll improve your problem-solving skills.
Be sure to practice each concept regularly to reinforce your understanding. The more familiar you become with these ideas, the more efficiently you’ll be able to solve problems during the assessment.
Common Mistakes to Avoid During the Test
When working under time pressure, it’s easy to make mistakes, even with a solid understanding of the material. Being aware of common errors can help you avoid them and improve your overall performance. This section highlights typical pitfalls that students often fall into and provides tips on how to prevent them.
One of the most frequent mistakes is rushing through questions without fully reading them. Skipping key details can lead to misinterpreting the problem and making unnecessary errors. Always take a moment to read each question carefully and ensure you understand what is being asked before starting to solve it.
Another common issue is forgetting to check your work. Many students solve a problem but fail to double-check their calculations, which can result in simple arithmetic mistakes that cost valuable points. Set aside a few minutes at the end to review your answers, especially those you found more challenging.
Additionally, failing to follow the correct order of operations can lead to errors in solving equations and expressions. Remember to perform calculations step-by-step, paying attention to parentheses, exponents, multiplication, division, addition, and subtraction in the correct sequence.
Finally, misreading or overlooking negative signs is a common mistake in equations and inequalities. Be careful when working with positive and negative numbers, as small errors here can change the outcome significantly. Always check for negative signs in your final answers and throughout your steps to avoid costly mistakes.
Practice Questions and Solutions
To strengthen your understanding and improve your problem-solving skills, it is essential to practice a variety of questions. Working through different types of problems helps you familiarize yourself with common scenarios and refine your techniques. Below, you will find practice questions along with detailed solutions to guide you through the process of solving them.
By reviewing the solutions after attempting each problem, you can identify any areas that need further attention and ensure that you’re applying the correct methods. Let’s go through a few practice problems together:
| Question | Solution |
|---|---|
| Solve for x: 2x + 5 = 15 | Subtract 5 from both sides: 2x = 10. Then divide by 2: x = 5. |
| Graph the equation: y = 3x – 4 | Plot the y-intercept (0, -4), then use the slope (3) to find another point (e.g., (1, -1)). Draw the line through the two points. |
| Solve for y: x^2 + y^2 = 25, when x = 3 | Substitute x = 3 into the equation: 9 + y^2 = 25. Subtract 9 from both sides: y^2 = 16. Take the square root: y = ±4. |
| Factor: x^2 + 5x + 6 | The factors of 6 that add to 5 are 2 and 3. So, the factored form is (x + 2)(x + 3). |
By consistently practicing these types of problems, you’ll become more comfortable with the methods required to solve them quickly and accurately. Be sure to attempt additional questions on your own and compare your solutions with the examples provided to track your progress.
How to Use the Calculator Effectively
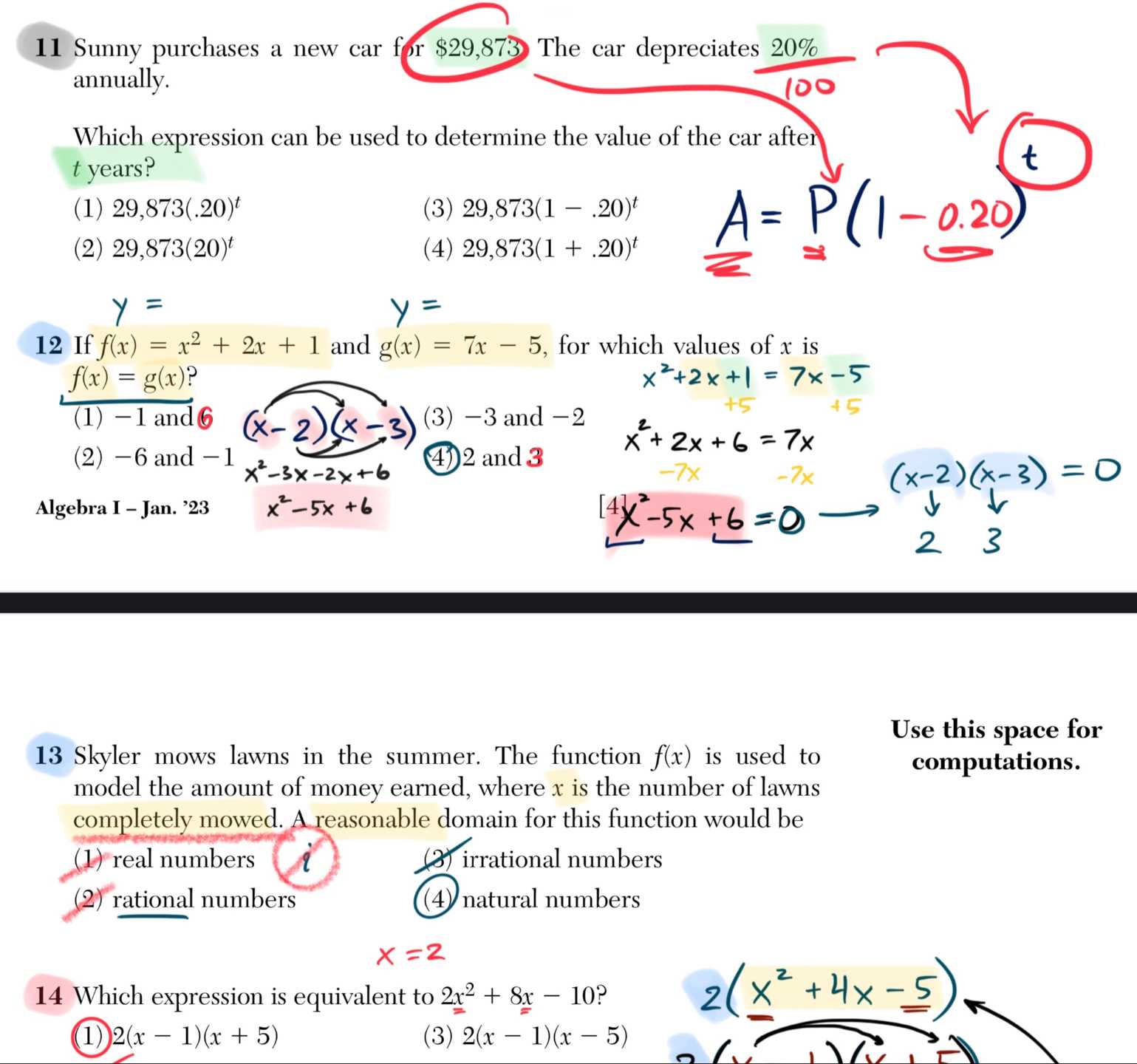
A calculator can be a powerful tool to help you solve problems quickly and accurately, but using it effectively requires understanding its functions and limitations. Relying on the calculator too much without knowing how to apply it properly can lead to mistakes and missed opportunities for deeper understanding. This section offers tips on how to maximize the benefits of a calculator while maintaining accuracy and efficiency.
First, familiarize yourself with the different functions of your calculator. Knowing how to use basic operations such as addition, subtraction, multiplication, and division is essential. However, most calculators also offer advanced functions, such as square roots, exponentiation, and trigonometric calculations. Understanding how to access these features and when to use them can save you valuable time.
Next, be mindful of order of operations when entering calculations. Many calculators follow a specific sequence when performing calculations, so it’s crucial to input equations carefully. Use parentheses to group terms and clarify the order in which operations should be performed. This helps prevent mistakes that arise from misinterpreting how the calculator processes the input.
Another useful strategy is to check intermediate steps. When solving a complex problem, it’s easy to make errors in your calculations. By periodically reviewing your input and results, you can catch mistakes before they affect the final answer.
Finally, remember that a calculator is a tool, not a replacement for understanding the underlying concepts. Use it to streamline calculations, but ensure you also know how to solve problems manually. This will give you more confidence and help you use the calculator effectively when needed.
Tips for Solving Word Problems
Word problems can often feel overwhelming, especially when they seem to contain a lot of information at once. However, breaking them down into smaller, manageable steps can make the process much easier. The key to solving these types of questions is understanding the information presented and translating it into mathematical expressions. Here are some strategies to help you approach word problems with confidence and clarity.
Steps to Tackle Word Problems
Follow these steps to effectively solve word problems:
- Read the problem carefully: Take your time to understand what the problem is asking. Highlight or underline key pieces of information, such as numbers, units, or relationships between variables.
- Identify what you need to find: Clearly define the unknowns in the problem. What is being asked? Are you solving for a specific value, such as a total or a rate?
- Translate words into math: Convert the problem’s description into mathematical expressions or equations. Use variables to represent unknown values and translate terms like “total,” “difference,” “sum,” and “product” into their corresponding operations.
- Set up the equation: Organize the information into a solvable equation. Be sure to check that all relevant details are included and that the equation accurately reflects the relationships described in the problem.
- Solve and check your work: Solve the equation step by step, and once you have a solution, review your answer in the context of the original question to make sure it makes sense.
Common Pitfalls to Avoid
While solving word problems, be aware of these common mistakes:
- Overlooking units: Always pay attention to the units given in the problem (e.g., inches, dollars, hours). Failing to convert or match units correctly can lead to incorrect answers.
- Skipping key details: Word problems often contain extraneous information designed to distract you. Stay focused on the important details that directly relate to solving the problem.
- Not checking your solution: Always double-check your final answer to make sure it logically fits the context of the problem. This can help you catch small mistakes you might have missed during the solving process.
By following these strategies and staying patient, you’ll find that word problems become easier to manage, helping you to perform better on tests and assignments.
Breaking Down Quadratic Equations
Quadratic equations are a staple in many mathematical problems, and while they may seem complex at first, they can be broken down into manageable parts. Understanding the structure of these equations allows for the application of various methods to solve them. In this section, we’ll explore the essential steps to tackle these problems efficiently and correctly.
A quadratic equation typically follows the form ax² + bx + c = 0, where a, b, and c are constants. The goal is to find the values of x that satisfy the equation. Here’s a step-by-step approach to breaking it down:
Steps to Solve a Quadratic Equation
Follow these key steps to solve a quadratic equation:
- Identify the coefficients: Recognize the values of a, b, and c in the equation. For example, in the equation 2x² + 5x – 3 = 0, a = 2, b = 5, and c = -3.
- Choose a method to solve: There are multiple ways to solve quadratic equations:
- Factoring: Look for two numbers that multiply to ac and add up to b.
- Completing the square: This involves rearranging the equation to make one side a perfect square trinomial.
- Using the quadratic formula: Apply the formula x = (-b ± √(b² – 4ac)) / 2a to find the roots.
- Solve for x: Depending on the method chosen, solve for the value(s) of x. If using factoring, you’ll find two factors of ax² + bx + c = 0 that make the equation true. For the quadratic formula, substitute the coefficients a, b, and c into the formula.
- Check your solutions: Once you’ve solved for x, substitute your solutions back into the original equation to verify that they are correct.
Common Mistakes to Avoid
While solving quadratic equations, be mindful of the following common errors:
- Forgetting to factor correctly: If you’re factoring, ensure you identify the correct factors that multiply to ac and add to b. This is one of the most frequent mistakes.
- Misapplying the quadratic formula: Double-check the values of a, b, and c before substituting them into the formula. A small error can lead to an incorrect solution.
- Ignoring complex solutions: When the discriminant (b² – 4ac) is negative, the equation will have complex solutions. Be sure to recognize and handle them properly.
By following these steps and avoiding common pitfalls, you can confidently solve quadratic equations and understand their solutions better.
Interpreting Graphs and Functions
Understanding how to read and analyze graphical representations of mathematical relationships is essential for solving many problems. A graph can provide insight into the behavior of a function, including its key features such as intercepts, slopes, and trends. Being able to interpret these visual cues is crucial for making informed decisions about the function’s properties and solutions.
In this section, we will focus on how to interpret graphs and functions, identifying key aspects like the slope, intercepts, and general shape of the graph. These tools help visualize relationships between variables and provide valuable information for solving problems more efficiently.
Identifying Key Features of a Graph
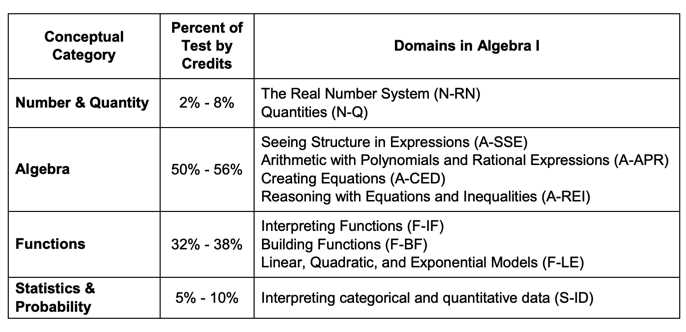
When interpreting a graph, there are several key features to look for:
- Intercepts: The points where the graph crosses the x-axis and y-axis are critical. The x-intercept is where the function equals zero, and the y-intercept is where the graph intersects the y-axis.
- Slope: The steepness or incline of the graph represents the rate of change of the function. A positive slope indicates an increasing function, while a negative slope indicates a decreasing function.
- Curvature: Some graphs curve in specific ways, indicating non-linear relationships. Identifying whether the graph is a straight line, parabola, or another shape can give insights into the function’s behavior.
Understanding Functions from Graphs
Interpreting a function from its graph requires analyzing the overall trend and specific details:
- Linear Functions: A straight line graph indicates a linear relationship between variables. The slope and intercept can easily be determined from the graph.
- Quadratic Functions: These graphs typically form a U-shape (parabola). Identifying the vertex and direction of the curve helps understand the function’s behavior.
- Piecewise Functions: Graphs of piecewise functions consist of multiple segments, each representing a different expression for the function. It’s important to observe where the graph switches between pieces.
By paying attention to these features, you can gain a deeper understanding of the relationships between variables and solve problems more effectively. Whether you are dealing with linear, quadratic, or other types of functions, interpreting graphs is a vital skill in mathematics.
Preparing for Multiple Choice Questions

Multiple choice questions are designed to test both your knowledge and your ability to analyze options quickly. To effectively approach these types of questions, it’s essential to develop a strategic method that allows you to select the correct answer while avoiding common pitfalls. Preparation involves not only reviewing the material but also practicing techniques to identify the most likely answers under time constraints.
In this section, we’ll cover effective strategies to tackle multiple choice questions, including how to eliminate incorrect choices, manage your time, and ensure that you approach each question with confidence. These techniques can significantly improve your performance when faced with this question format.
Effective Strategies for Answer Selection
When facing multiple choice questions, the following strategies can help guide your decision-making process:
- Eliminate Clearly Incorrect Options: Start by crossing out the options that are obviously wrong. This increases the chances of selecting the correct answer even if you’re unsure about the remaining options.
- Look for Clues in the Question: Pay close attention to any keywords or phrasing in the question that might give hints about the correct answer. Words like “always,” “never,” or “only” can provide insight into the answer’s nature.
- Consider All Options: Don’t rush to pick the first option that seems correct. Often, multiple choices can seem right at first glance. Make sure to review all the answers before making your final selection.
Time Management for Multiple Choice Questions

Managing your time efficiently during a test with multiple choice questions is essential. Here are a few tips:
- Set a Time Limit per Question: Allocate a specific amount of time to each question, and stick to it. If you’re unsure about an answer, move on and come back to it later if time permits.
- Prioritize Easier Questions: Begin with the questions that seem easiest to answer. This helps build confidence and ensures that you complete the questions you are most comfortable with first.
- Leave Time for Review: After answering all questions, review your choices. Double-check for any mistakes or questions you may have missed and ensure that your answers are consistent with your calculations.
By practicing these strategies, you’ll be better prepared to handle multiple choice questions efficiently and confidently, improving your overall performance on any test.
Reviewing Algebraic Properties and Theorems
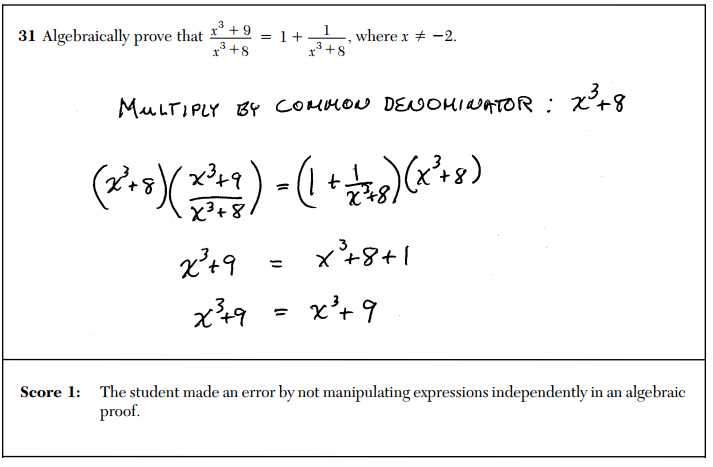
Understanding fundamental properties and theorems is essential for solving complex problems efficiently. These concepts form the backbone of mathematical reasoning and help simplify various calculations and proofs. By mastering these key ideas, you’ll be able to approach problems with a clearer perspective and apply logical methods to arrive at the correct solution.
In this section, we will explore some of the most important properties and theorems that play a critical role in mathematical problem-solving. Familiarizing yourself with these concepts ensures that you can apply them effectively under different conditions, enhancing your overall problem-solving skills.
Essential Mathematical Properties
The following properties are crucial in solving equations and simplifying expressions:
- Commutative Property: This property states that the order in which two numbers are added or multiplied does not affect the result. For example, a + b = b + a and ab = ba.
- Associative Property: It states that the grouping of numbers does not affect the sum or product. For instance, (a + b) + c = a + (b + c) and (ab)c = a(bc).
- Distributive Property: This property allows you to multiply a number by a sum or difference. For example, a(b + c) = ab + ac.
Key Theorems for Problem-Solving
Several theorems are essential for understanding and solving equations, especially in more advanced topics:
- Pythagorean Theorem: This well-known theorem helps in determining the relationship between the sides of a right triangle. It states that a² + b² = c², where c is the hypotenuse.
- Quadratic Formula: Used to solve quadratic equations, the formula is x = (-b ± √(b² – 4ac)) / 2a, where ax² + bx + c = 0.
- Zero Product Property: This theorem is crucial for solving equations involving products. It states that if the product of two factors equals zero, then at least one of the factors must be zero. For example, ab = 0 implies a = 0 or b = 0.
Mastering these properties and theorems provides the foundation for more advanced topics and problem-solving techniques, helping you approach challenges with confidence and efficiency.
Improving Speed and Accuracy
Enhancing both speed and accuracy is essential for tackling mathematical problems effectively, especially when working under time constraints. The ability to solve problems quickly without sacrificing precision can make a significant difference in achieving success. This section will explore practical strategies to help you improve your problem-solving efficiency while ensuring correctness.
By practicing specific techniques, such as honing mental math skills and organizing your approach, you can increase your confidence and reduce errors. The goal is to develop a rhythm that allows you to navigate through challenges with both speed and precision, ultimately maximizing your performance.
Effective Time Management
Proper time management is crucial for solving problems quickly. Here are a few strategies to consider:
- Prioritize Easy Questions: Start with questions you are most comfortable with to build confidence and save time for more complex ones.
- Set a Time Limit: Assign a specific amount of time to each problem and stick to it, ensuring you don’t spend too much time on any single question.
- Avoid Overthinking: Don’t get bogged down by unnecessary details. Stick to the basics and move on if you’re stuck.
Minimizing Mistakes
Reducing errors is equally important as improving speed. Some tips to help avoid mistakes include:
- Double-Check Key Steps: Quickly review the crucial parts of your calculations to make sure you haven’t overlooked any errors.
- Write Neatly: Clear organization of your work helps prevent misunderstandings or missed steps.
- Use Process of Elimination: If unsure about an answer, systematically eliminate the obviously incorrect choices.
With consistent practice and these strategies, you’ll gradually become more efficient and precise, ensuring you perform well under timed conditions.
How to Review After the Exam
Reviewing your performance after completing any assessment is a key step in improving future results. Reflecting on the process allows you to identify areas of strength and weaknesses, ensuring that you are better prepared next time. This section will outline effective strategies for post-assessment review to help you make the most of your experience and enhance your skills for future challenges.
Once the test is finished, it’s important to go beyond simply looking at your score. Focusing on the mistakes you made and understanding why they occurred will provide valuable insights for your next approach. By breaking down the questions you struggled with, you can pinpoint specific concepts that need more attention and practice.
Review the Mistakes
Start by analyzing any questions you answered incorrectly. Here’s how to go about it:
- Identify Patterns: Are there any recurring themes in the questions you struggled with? This could point to gaps in your understanding of certain concepts.
- Understand the Missteps: Take time to figure out why you made certain mistakes. Did you misinterpret the question or make a calculation error? Pinpointing the issue will help you avoid it next time.
- Seek Help if Needed: If you can’t figure out why a mistake was made, don’t hesitate to ask for guidance from a teacher, tutor, or study group.
Reinforce Your Knowledge
After reviewing your mistakes, the next step is to reinforce the concepts that need improvement. Consider these strategies:
- Practice Similar Problems: Once you’ve identified areas of weakness, practice similar problems to strengthen your understanding and build confidence.
- Use Resources: Take advantage of textbooks, online tutorials, or study guides to further explain and clarify difficult topics.
- Create a Study Plan: Based on your review, develop a focused study plan to address weak areas and prepare for future assessments.
By taking the time to review after each test, you not only improve your performance but also gain a deeper understanding of the material. With regular reflection and practice, you’ll continuously grow and become more adept at handling challenges in the future.